Chapter 1, Problem 1.39P

To determine

The position of the ball as a function of time and to show that the range of the ball is $R=\frac{2v_0^2\sin \theta \cos \left(\theta +\varphi \right)}{g{\cos }^2\varphi }$ and the maximum possible range is $R_{\text{max}}=\frac{v_0^2}{g\left(1+\sin \varphi \right)}$.

Answer to Problem 1.39P

The position of the ball as a function of time is $\left(\left(v_0\cos \theta \right)t-\frac{1}{2}\left(g\sin \varphi \right)t^2,\left(v_0\sin \theta \right)t-\frac{1}{2}\left(g\cos \varphi \right)t^2,0\right)$ and it is showed that the range of the ball is $R=\frac{2v_0^2\sin \theta \cos \left(\theta +\varphi \right)}{g{\cos }^2\varphi }$ and the maximum possible range is $R_{\text{max}}=\frac{v_0^2}{g\left(1+\sin \varphi \right)}$.

Explanation of Solution

Assume positive x-axis to be pointing up along the inclined plane and the positive y-axis to be pointing normal to the inclined plane.

The velocity components of the ball are shown in figure 1.

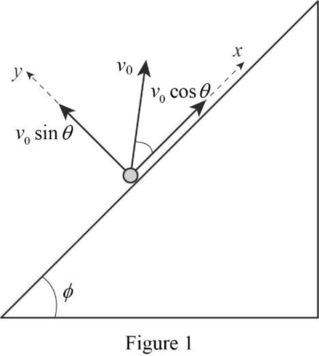
Write the equation for the component of initial velocity of the ball in x-direction.

  $v_{0x}=v_0\cos \theta$        (I)

Here, $v_{0x}$ is the component of initial velocity of the ball in x-direction, $v_0$ is the initial velocity of the ball and $\theta$ angle of the initial velocity above the inclined plane.

Write the equation for the component of initial velocity of the ball in y-direction.

  $v_{0y}=v_0\sin \theta$        (II)

Here, $v_{0y}$ is the component of initial velocity of the ball in y-direction.

The weight components of the ball are shown in figure 2.

From the diagram, it is clear that the only force acting on the ball in x-direction is sine component of the weight of the ball.

Write the equation for the net force on the ball in x-direction.

  $F_x=-mg\sin \varphi$

Here, $F_x$ is the net force on the ball in x-direction, $m$ is the mass of the ball, $g$ is the acceleration due to gravity and $\varphi$ is the angle at which the plane is inclined above the horizontal.

Write the equation for net force in x-direction.

  $F_x=m{a}_x$

Here, $a_x$ is the acceleration of the ball in x-direction.

Compare the above two equations and write the equation for the acceleration of the ball in x-direction.

  $a_x=-g\sin \varphi$        (III)

Write the equation for the displacement of the ball along x-axis during time $t$ .

  $x=v_{0x}t+\frac{1}{2}{a}_x{t}^2$        (IV)

Here, $x$ is the displacement of the ball along x-axis during time $t$

Put equations (I) and (III) in equation (II).

  $\begin{array}{c}x=\left(v_0\cos \theta \right)t+\frac{1}{2}\left(-g\sin \varphi \right)t^2\\ =\left(v_0\cos \theta \right)t-\frac{1}{2}\left(g\sin \varphi \right)t^2\end{array}$        (V)

From the diagram, it is clear that the only force acting on the ball in y-direction is cosine component of the weight of the ball.

Write the equation for the net force on the ball in y-direction.

  $F_y=-mg\cos \varphi$

Here, $F_y$ is the net force on the ball in y-direction.

Write the equation for net force in y-direction.

  $F_y=m{a}_y$

Here, $a_y$ is the acceleration of the ball in y-direction.

Compare the above two equations and write the equation for the acceleration of the ball in y-direction.

  $a_y=-g\cos \varphi$        (VI)

Write the equation for the displacement of the ball along y-axis during time $t$ .

  $y=v_{0y}t+\frac{1}{2}{a}_y{t}^2$        (VII)

Here, $y$ is the displacement of the ball along y-axis during time $t$

Put equations (II) and (VI) in equation (VII).

  $\begin{array}{c}y=\left(v_0\sin \theta \right)t+\frac{1}{2}\left(-g\cos \varphi \right)t^2\\ =\left(v_0\sin \theta \right)t-\frac{1}{2}\left(g\cos \varphi \right)t^2\end{array}$        (VIII)

Write the expression for the displacement of the ball along the $z$ direction.

  $z=0$        (IX)

Here, $z$ is the displacement of the ball along the $z$ direction.

Write the equation for the position of the ball after time $t$ .

  $\overrightarrow{r}=\left(x,y,z\right)$

Here, $\overrightarrow{r}$ is the position of the ball after time $t$ .

Put equations (V), (VIII) and (IX) in the above equation.

  $\left(\left(v_0\cos \theta \right)t-\frac{1}{2}\left(g\sin \varphi \right)t^2,\left(v_0\sin \theta \right)t-\frac{1}{2}\left(g\cos \varphi \right)t^2,0\right)$

When the ball lands on the inclined plane, its total displacement in y-direction will be zero.

Substitute $0$ for $y$ in equation (VIII).

  $\begin{array}{c}0=\left(v_0\sin \theta \right)t-\frac{1}{2}\left(g\cos \varphi \right)t^2\\ \frac{1}{2}\left(g\cos \varphi \right)t=\left(v_0\sin \theta \right)\\ t=\frac{2\left(v_0\sin \theta \right)}{g\cos \varphi }\end{array}$

Here, $t$ is the time of flight of the ball.

The horizontal displacement of the ball during the time of flight is its range.

Put the above equation in equation (V) to find the expression for the range.

  $\begin{array}{c}R=\left(v_0\cos \theta \right)\left(\frac{2\left(v_0\sin \theta \right)}{g\cos \varphi }\right)-\frac{1}{2}\left(g\sin \varphi \right){\left(\frac{2\left(v_0\sin \theta \right)}{g\cos \varphi }\right)}^2\\ =\frac{2v_0^2\sin \theta \cos \theta }{g\cos \varphi }-\frac{2v_0^2\sin \varphi {\sin }^2\theta }{g{\cos }^2\varphi }\\ =\frac{2v_0^2\sin \theta }{g{\cos }^2\varphi }\left(\cos \theta \cos \varphi -\sin \varphi \sin \theta \right)\\ =\frac{2v_0^2\sin \theta }{g{\cos }^2\varphi }\cos \left(\theta +\varphi \right)\end{array}$        (X)

Here, $R$ is the range of the ball.

The range will be maximum when the first derivative of the range with respect to the angle of projection is zero.

Write the condition for maximum range.

  $\frac{dR}{d\theta }=0$        (XI)

Take the derivative of equation (X) with respect to $\theta$ .

  $\begin{array}{c}\frac{dR}{d\theta }=\frac{d}{d\theta }\left(\frac{2v_0^2\sin \theta }{g{\cos }^2\varphi }\cos \left(\theta +\varphi \right)\right)\\ =\frac{2v_0^2}{g{\cos }^2\varphi }\left[\cos \theta \cos \left(\theta +\varphi \right)+\sin \theta \left(-\sin \left(\theta +\varphi \right)\right)\right]\\ =\frac{2v_0^2\cos \theta }{g{\cos }^2\varphi }\cos \left(\theta +\varphi \right)-\frac{2v_0^2\sin \theta }{g{\cos }^2\varphi }\sin \left(\theta +\varphi \right)\end{array}$

Put the above equation in equation (XI).

  $\begin{array}{c}\frac{2v_0^2\cos \theta }{g{\cos }^2\varphi }\cos \left(\theta +\varphi \right)-\frac{2v_0^2\sin \theta }{g{\cos }^2\varphi }\sin \left(\theta +\varphi \right)=0\\ \cos \theta \cos \left(\theta +\varphi \right)-\sin \theta \sin \left(\theta +\varphi \right)=0\\ \cos \left(\theta +\left(\theta +\varphi \right)\right)=0\\ \cos \left(2\theta +\varphi \right)=0\end{array}$

The value of $\theta$ for which $\cos \theta$ becomes zero is $\frac{\pi }{2}$ .

Equate $2\theta +\varphi$ to $\frac{\pi }{2}$ .

  $\begin{array}{c}2\theta +\varphi =\frac{\pi }{2}\\ 2\theta =\frac{\pi }{2}-\varphi \\ =\frac{\pi -2\varphi }{2}\\ \theta =\frac{\pi -2\varphi }{4}\end{array}$

Substitute the above determined value of $\theta$ in equation (X) to find the maximum range.

  $\begin{array}{c}{R}_{\text{max}}=\frac{2v_0^2\sin \left(\frac{\pi -2\varphi }{4}\right)}{g{\cos }^2\varphi }\cos \left(\frac{\pi -2\varphi }{4}+\varphi \right)\\ =\frac{2v_0^2\sin \left(\frac{\pi -2\varphi }{4}\right)\cos \left(\frac{\pi -2\varphi +4\varphi }{4}\right)}{g{\cos }^2\varphi }\\ =\frac{2v_0^2\sin \left(\frac{\pi -2\varphi }{4}\right)\cos \left(\frac{\pi +2\varphi }{4}\right)}{g{\cos }^2\varphi }\end{array}$

Here, $R_{\text{max}}$ is the maximum possible range of the ball.

Use the trigonometric identity $2\sin A\cos B=\sin \left(A+B\right)+\sin \left(A-B\right)$ in the above equation.

  $\begin{array}{c}{R}_{\text{max}}=\frac{v_0^2\left(\sin \left(\frac{\pi -2\varphi }{4}+\frac{\pi +2\varphi }{4}\right)+\sin \left(\frac{\pi -2\varphi }{4}-\left(\frac{\pi +2\varphi }{4}\right)\right)\right)}{g{\cos }^2\varphi }\\ =\frac{v_0^2\left(\sin \frac{\pi }{2}+\sin \left(-\varphi \right)\right)}{g\left(1-{\sin }^2\varphi \right)}\\ =\frac{v_0^2\left(1-\sin \varphi \right)}{g\left(1-\sin \varphi \right)\left(1+\sin \varphi \right)}\\ =\frac{v_0^2}{g\left(1+\sin \varphi \right)}\end{array}$

Conclusion:

Therefore, the position of the ball as a function of time is $\left(\left(v_0\cos \theta \right)t-\frac{1}{2}\left(g\sin \varphi \right)t^2,\left(v_0\sin \theta \right)t-\frac{1}{2}\left(g\cos \varphi \right)t^2,0\right)$ and it is showed that the range of the ball is $R=\frac{2v_0^2\sin \theta \cos \left(\theta +\varphi \right)}{g{\cos }^2\varphi }$ and the maximum possible range is $R_{\text{max}}=\frac{v_0^2}{g\left(1+\sin \varphi \right)}$.