You are interested in finding a 90% confidence interval for the mean number of visits for physical therapy patients. The data below show the number of visits for 13 randomly selected physical therapy patients. 8 17 21 18 22 16 27 17 25 18 20 6 6 a. To compute the confidence interval use a ? t z distribution. b. With 90% confidence the population mean number of visits per physical therapy patient is between and visits. c. If many groups of 13 randomly selected physical therapy patients are studied, then a different confidence interval would be produced from each group. About percent of these confidence intervals will contain the true population mean number of visits per patient and about percent will not contain the true population mean number of visits per patient.
You are interested in finding a 90% confidence interval for the
| 8 | 17 | 21 | 18 | 22 | 16 | 27 | 17 | 25 | 18 | 20 | 6 | 6 |
a. To compute the confidence interval use a ? t z distribution.
b. With 90% confidence the population mean number of visits per physical therapy patient is between and visits.
c. If many groups of 13 randomly selected physical therapy patients are studied, then a different confidence interval would be produced from each group. About percent of these confidence intervals will contain the true population mean number of visits per patient and about percent will not contain the true population mean number of visits per patient.
To compute the 90% confidence interval for the mean number of visits for physical therapy patients t distribution is used since because the population standard deviation is not known to construct confidence interval using Z moreover the sample is less than 30.
Correct option: t distribution.
Obtain the 90% confidence interval for the mean number of visits for physical therapy patients.
The 90% confidence interval for the mean number of visits for physical therapy patients is obtained below:
The formula for the Confidence interval for mean is:
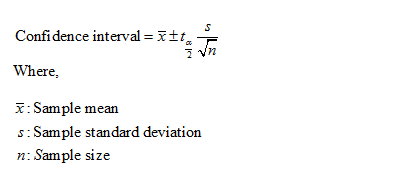
Use EXCEL Procedure for mean and standard deviation for the given samples.
Follow the instruction to obtain the values of mean and SD:
- Open EXCEL
- Go to Data>Data Analysis.
- Choose Descriptive Statistics.
- Enter the input range as $A$1:$A$14.
- Check the Labels in the first row.
- Check the option Summary statistics
- Click OK.
EXCEL output:
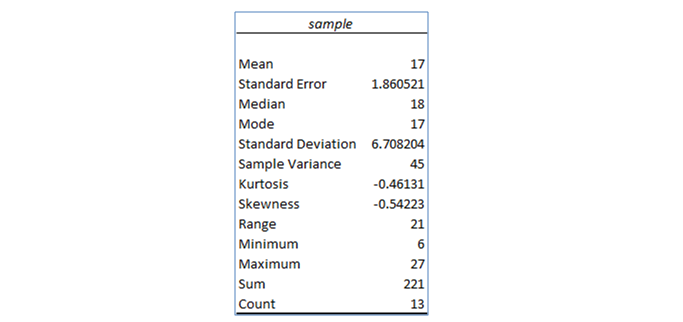
From the EXCEL output, the value of mean is 17 and the standard deviation is 6.7082.
Obtain the degrees of freedom.
The degrees of freedom is obtained below:
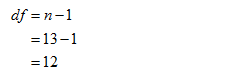
Use EXCEL Procedure for finding the critical value of t.
Follow the instruction to obtain the critical value of t:
- Open EXCEL
- Go to Formula bar.
- In formula bar enter the function as“=TINV”
- Enter the probability as 0.10.
- Enter the degrees of freedom as 12.
- Click enter
EXCEL output:
From the EXCEL output, the critical value of t at the 0.10 level of significance with the 12 degrees of freedom is 1.7823
The required confidence interval is,

The 90% confidence interval for the mean number of visits for physical therapy patients lies between 13.6840 visits and 20.3160 visits.
Trending now
This is a popular solution!
Step by step
Solved in 3 steps with 4 images









