use the inverse matrix of coefficients to find the solution of this system of equations how the work above, and write your answer in the box below he work abor y = 3.48 Z=-2.28 X = 3.44 W = 7.76
use the inverse matrix of coefficients to find the solution of this system of equations how the work above, and write your answer in the box below he work abor y = 3.48 Z=-2.28 X = 3.44 W = 7.76
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
I need help on c. The info is on number 6
![### Production Matrix and System of Equations
**(c) Production Matrix**
The production matrix \( X \) is given by:
\[
X = \begin{bmatrix} 25.0100 \\ 25.1695 \\ 49.4685 \end{bmatrix}
\]
**6. System of Equations**
Consider the following system of equations:
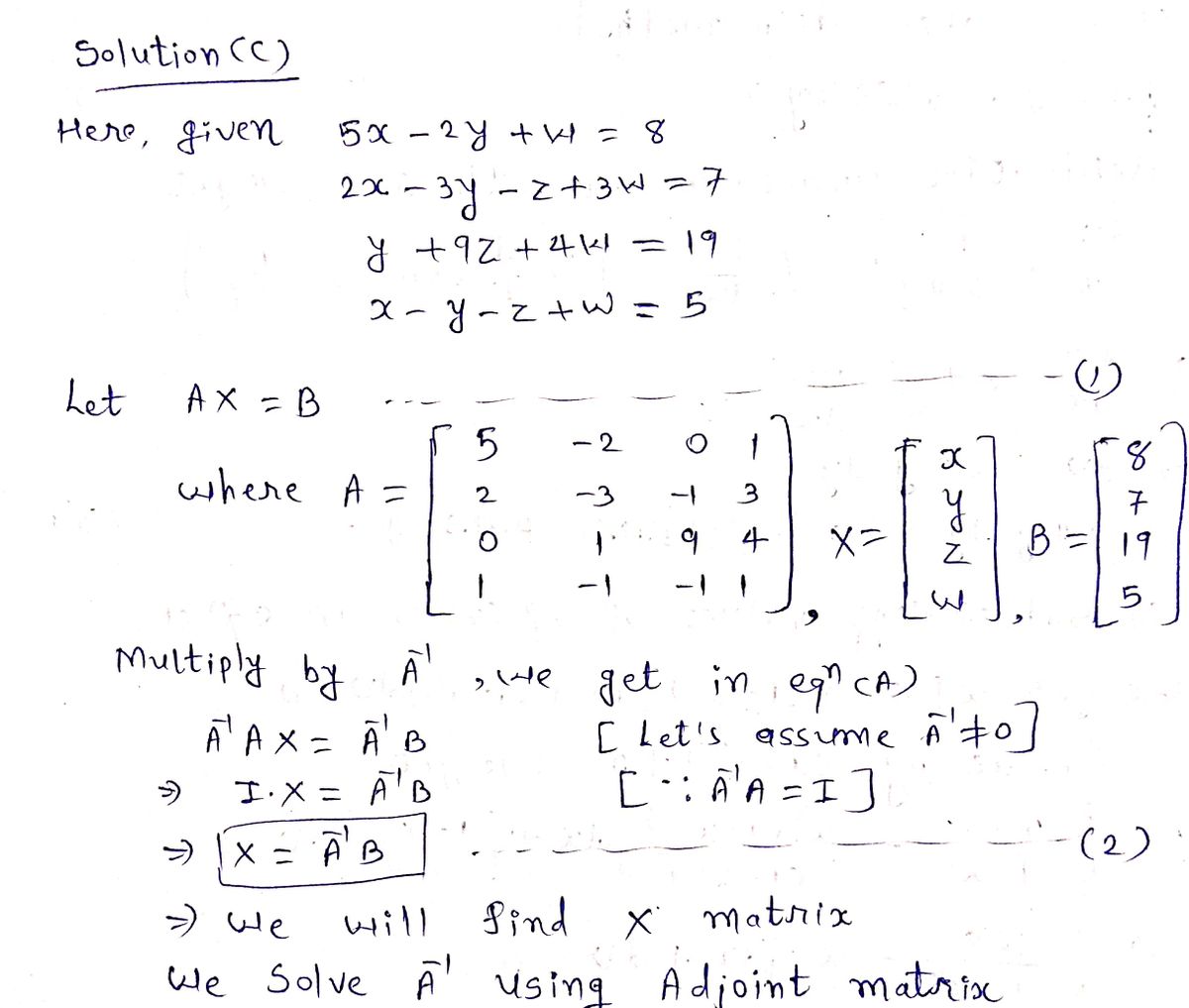
1. \( 5x - 2y + w = 8 \)
2. \( 2x - 3y - z + 3w = 7 \)
3. \( y + 9z + 4w = 19 \)
4. \( x - y - z + w = 5 \)
**(a) Matrix of Coefficients**
The matrix of coefficients \( A \) is:
\[
A = \begin{bmatrix}
5 & -2 & 0 & 1 \\
2 & -3 & -1 & 3 \\
0 & 1 & 9 & 4 \\
1 & -1 & -1 & 1
\end{bmatrix}
\]
This matrix \( A \) represents the coefficients of the variables \( x \), \( y \), \( z \), and \( w \) from each of the equations in the system. Each row corresponds to an equation, and each column corresponds to a variable in the order \( x \), \( y \), \( z \), \( w \).](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2Fc62debf9-2696-4946-934a-f308bf93cf3b%2Fdec2b111-193e-4a30-8808-581aca7a1698%2Ff92qn45_processed.jpeg&w=3840&q=75)
Transcribed Image Text:### Production Matrix and System of Equations
**(c) Production Matrix**
The production matrix \( X \) is given by:
\[
X = \begin{bmatrix} 25.0100 \\ 25.1695 \\ 49.4685 \end{bmatrix}
\]
**6. System of Equations**
Consider the following system of equations:
1. \( 5x - 2y + w = 8 \)
2. \( 2x - 3y - z + 3w = 7 \)
3. \( y + 9z + 4w = 19 \)
4. \( x - y - z + w = 5 \)
**(a) Matrix of Coefficients**
The matrix of coefficients \( A \) is:
\[
A = \begin{bmatrix}
5 & -2 & 0 & 1 \\
2 & -3 & -1 & 3 \\
0 & 1 & 9 & 4 \\
1 & -1 & -1 & 1
\end{bmatrix}
\]
This matrix \( A \) represents the coefficients of the variables \( x \), \( y \), \( z \), and \( w \) from each of the equations in the system. Each row corresponds to an equation, and each column corresponds to a variable in the order \( x \), \( y \), \( z \), \( w \).

Transcribed Image Text:Title: Optimization Problem for Bread Production
---
**Problem Statement:**
A baker can bake at most 90 loaves of bread in one batch. The batch will consist of rye bread \( x \), and peasant bread \( y \).
- A loaf of rye bread contains 0.9 kg of rye flour and 0.1 kg of wheat flour.
- A loaf of peasant bread contains 0.3 kg of rye flour and 0.45 kg of wheat flour.
The baker has 75 kg of rye flour and 45 kg of wheat flour available.
- One loaf of rye bread sells for $6.
- One loaf of peasant bread sells for $7.
The baker wants to maximize the revenue.
**Tasks:**
(a) Formulate this optimization problem. Write the objective function and the constraints.
(b) Use the inverse matrix of coefficients to find the solution of this system of equations.
**Solution:**
Show the work above, and write your answer in the box below:
- \( X = 3.44 \)
- \( Y = 3.48 \)
- \( Z = 1.28 \)
- \( W = 7.76 \)
Expert Solution
Step 1
Step by step
Solved in 6 steps with 6 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

