Two progressive cosine waves y1(x, t) and y2(x, t) ( of type y(x, t) = Acos (kx ± ωt + φ) ) have the same amplitude A = 3.0cm, propagate in opposite directions, have the same wavelength λ = 1.5cm, the same propagation speed v = 15, 0cm/s, and they have a phase difference between them equal φ = +π/2 radians. Get the resulting waveform expression yR(x, t) of these two oscillatory motions. Show that it can be written as yR(x, t) = 6 cos (4, 19x +π/4) cos (62, 8t +π/4)
Two progressive cosine waves y1(x, t) and y2(x, t) ( of type y(x, t) = Acos (kx ± ωt + φ) ) have the same amplitude A = 3.0cm, propagate in opposite directions, have the same wavelength λ = 1.5cm, the same propagation speed v = 15, 0cm/s, and they have a phase difference between them equal φ = +π/2 radians. Get the resulting waveform expression yR(x, t) of these two oscillatory motions. Show that it can be written as yR(x, t) = 6 cos (4, 19x +π/4) cos (62, 8t +π/4)
Related questions
Question
100%
Two progressive cosine waves y1(x, t) and y2(x, t) ( of type y(x, t) = Acos (kx ± ωt + φ) ) have the same amplitude A = 3.0cm, propagate in opposite directions, have the same wavelength λ = 1.5cm, the same propagation speed v = 15, 0cm/s, and they have a phase difference between them equal φ = +π/2 radians. Get the resulting waveform expression yR(x, t) of these two oscillatory motions. Show that it can be written as
yR(x, t) = 6 cos (4, 19x +π/4) cos (62, 8t +π/4)
Expert Solution
Step 1
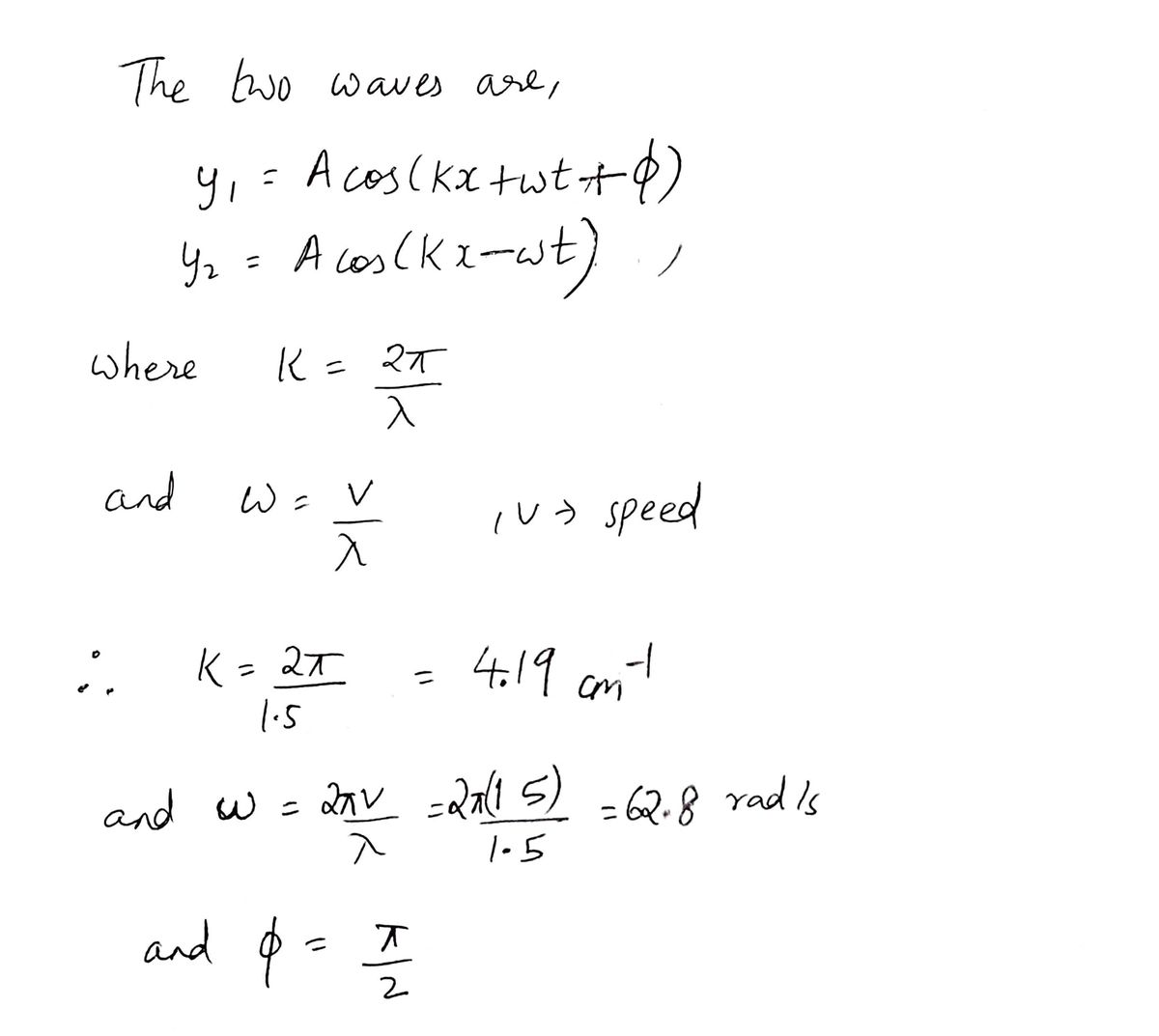
Step by step
Solved in 2 steps with 2 images
