The money raised and spent (both in millions of dollars) by all congressional campaigns for 8 recent 2-year periods are shown in the table. The equation of the regression line is y = 0.942x + 27.609. Find the standard error of estimate s, and interpret the result. 1042.3 957.7 1203.3 450.7 673.7 745.1 778.6 793.9 Money raised, x Money spent, y 734.8 1024.2 929.1 1160.6 448.6 697.9 735.7 751.2 Find the standard error of estimate s, and interpret the result. (Round to three decimal places as needed.) How can the standard error of estimate be interpreted? O A. The standard error of estimate of the money raised for a specific amount of money spent is about s, million dollars O B. The standard error of estimate of the money spent for a specific amount of money raised is about s, million dollars.
Correlation
Correlation defines a relationship between two independent variables. It tells the degree to which variables move in relation to each other. When two sets of data are related to each other, there is a correlation between them.
Linear Correlation
A correlation is used to determine the relationships between numerical and categorical variables. In other words, it is an indicator of how things are connected to one another. The correlation analysis is the study of how variables are related.
Regression Analysis
Regression analysis is a statistical method in which it estimates the relationship between a dependent variable and one or more independent variable. In simple terms dependent variable is called as outcome variable and independent variable is called as predictors. Regression analysis is one of the methods to find the trends in data. The independent variable used in Regression analysis is named Predictor variable. It offers data of an associated dependent variable regarding a particular outcome.

Obtain the value of the standard error of estimate.
The value of the standard error of estimate is obtained below as follows:
Use EXCEL to obtain the value of se.
EXCEL procedure:
- Go to EXCEL
- Go to Data>Data Analysis.
- Choose Regression.
- Input Y range as $B$1:$B$9.
- Input X range as $A$1:$A$9.
- Check the option Labels in First row.
- Click OK.
EXCEL output:
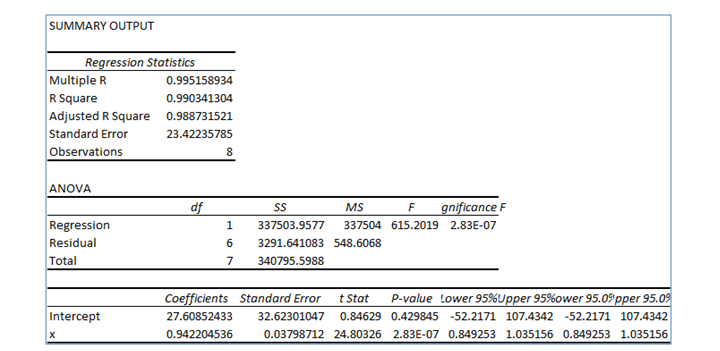
From the EXCEL output, the value of standard error is 23.422
Thus, the value of the standard error of the estimate is 23.422.
Step by step
Solved in 2 steps with 1 images









