The average American man consumes 9.9 grams of sodium each day. Suppose that the sodium consumption of American men is normally distributed with a standard deviation of 0.9 grams. Suppose an American man is randomly chosen. Let X = the amount of sodium consumed. Round all numeric answers to 4 decimal places where possible. a. What is the distribution of X? X - N( b. Find the probability that this American man consumes between 10.4 and 11.1 grams of sodium per day. c. The middle 30% of American men consume between what two weights of sodium? Low: High:
Contingency Table
A contingency table can be defined as the visual representation of the relationship between two or more categorical variables that can be evaluated and registered. It is a categorical version of the scatterplot, which is used to investigate the linear relationship between two variables. A contingency table is indeed a type of frequency distribution table that displays two variables at the same time.
Binomial Distribution
Binomial is an algebraic expression of the sum or the difference of two terms. Before knowing about binomial distribution, we must know about the binomial theorem.
![The average American man consumes 9.9 grams of sodium each day. Suppose that the sodium consumption of
American men is normally distributed with a standard deviation of 0.9 grams. Suppose an American man is
randomly chosen. Let X = the amount of sodium consumed. Round all numeric answers to 4 decimal places
where possible.
a. What is the distribution of X? X - N
b. Find the probability that this American man consumes between 10.4 and 11.1 grams of sodium per day.
c. The middle 30% of American men consume between what two weights of sodium?
Low:
High:
Hint:
Helpful videos:
• Find a Probability [+]
Finding a Value Given a Probability [+]
Hint
Question Help: Message instructor
Submit Question
logi
*2
*3
F5
%23
3.
W
R
T.
Y
U
*CO
లే N](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F5de1fd98-4e1b-4d1e-9092-2fb53b09f0c6%2Fa2898306-347e-4508-8909-e8d870af9226%2Fj628d84_processed.jpeg&w=3840&q=75)
Given:
a) The distribution of X is normal with mean 9.9 and standard deviation 0.9.
Thus, the distribution of X is X~N(9.9, )
b)
The probability that the american man consumes between 10.4 and 11.1 grams is obtained as below:
The p-value is obtained using Excel "=NORMSDIST(Z)"
Output from Excel is given below:

From the output, the p-value of P(Z<1.3333) is 0.9087.
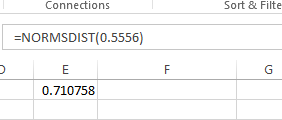
From the output, the p-value of P(Z<0.5556) is 0.7108.
Thus, the probability that the American man consumes between 10.4 and 11.1 grams is 0.1979.
Trending now
This is a popular solution!
Step by step
Solved in 2 steps with 3 images









