The annual yield
Q: Suppose that the walking step lengths of males are normally distributed with a mean of 2.5 feet and…
A: We have to find given probability.
Q: If the average low temperature of a winter month in Rochester, NY is 13∘13∘ and the standard…
A: Consider a variable, X: low temperature of a winter month in Rochester.To find the probability(in…
Q: 1. The nicotine content of a certain brand of king size cigarette has a normal distribution with a…
A:
Q: You own an investment which has an arithmetic mean return of 30% and a standard deviation of 15%.…
A:
Q: approximately normally distributed, find the probability that a randomly selected monthly payment…
A: The average monthly mortgage payment including principal and interest is P98200. If the standard…
Q: The net profit from a certain investment is normally distributed with a mean of $10,000 and a…
A: Random variable : X = Net profit from a certain investment Population mean : Population standard…
Q: Investors commonly use the standard deviation of the monthly percentage return for a mutual fund as…
A: Hey, since there are multiple subparts posted, we will answer first three subparts. If you want any…
Q: The quarterly returns for a group of 78 mutual funds with a mean of 3.2% and a standard deviation of…
A:
Q: 32. A stock has an average historical return of 11.3 percent and a standard deviation of 20.2…
A: The mean is μ=11.3The standard deviation is σ=20.2The objective is to choose the correct range of…
Q: Suppose that,to test the profitability of name changes in the more recent market (the past 5 years),…
A: The Z-score of a random variable X is defined as follows: Z = (X – µ)/σ. Here, µ and σ are the mean…
Q: Suppose the following data show the introductory interest rates on a sample of 5 credit cards: 5.4,…
A: Solution: The formula for the sample standard deviation as s=∑x2-(∑x)2nn-1 x x2 5.4 29.16…
Q: Investors commonly use the standard deviation of the monthly percentage return for a mutual fund as…
A: From the provided information, The p value is 0.0301. Level of significance (α) = 0.05
Q: Use the following information to calculate the expected return and standard deviation of a portfolio…
A: The expected return and standard deviation of a portfolio are given below,3 Doors, IncDown…
Q: The 5-year annual investment returns of MGM Resorts International (MGM) are below: Year Annual…
A: The standard deviation of annual returns can be calculated using the formula given by, SD = √[(Ri –…
Q: 4. Image a gambling casino consisting of 100 busy roulette tables. Suppose that each table brings in…
A: Let, x denotes the average hourly profit from each table . Number of such tables =100 Ex=50 σx=25
Q: d. What is the probability that average yield for 10 days is at most 800 tons? e. What is the…
A:
Q: 5. Changes in the value of two particular cryptocurrencies are modelled as Normal distributions.…
A:
Q: Maria and Zoe are taking Biology 105 but are in different classes. Maria’s class has an average of…
A:
Q: government bonds are normally distributed. Based on the information, what is the approximate…
A: Here given rate of return normally distributed Mean = 5.8 Standard deviation = 9.3% X = rate of…
Q: 3. In the long run, anqual real returns on common stocks have been normally distributed with mean…
A: Note : "Since you have posted a question with multiple subparts, we will solve first three subparts…
Q: The interest rate for the financing of a used car is on average 9% with a standard deviation of 3%.…
A:
Q: 1. Refer to the various selling prices of various homes in a community that follow the normal…
A:
Q: Suppose the following data show the introductory interest rates on a sample of 5 credit cards: 5.3,…
A:
Q: Today, the waves are crashing onto the beach every 4.6 seconds. The times from when a person arrives…
A: Continuous uniform distribution:A random variable X is said to have the rectangular distribution or…
Q: Suppose that we know that 33.8% of the people born in the United States between 1936 and 1951 have…
A:
Q: The historical returns on a portfolio had an average return of 9% and a standard deviation of 5%.…
A: Mean()=9standard deviation()=5
Q: Suppose that the walking step lengths of adult males are normally distributed with a mean of 2.72.7…
A: Answer Given a mean of 2.7 feet and a standard deviation of 0.2 feet. A sample of 63 men’s step…
Q: STATE: Andrew plans to retire in 4040 years. He plans to invest part of his retirement funds in…
A: The mean is 11% and the standard deviation is 17%.
Q: You make an investment. Assume that annual returns are normally distributed with a mean return of…
A: Given Information: Mean μ=0.05 Standard deviation σ=0.20
Q: The portfolio with 0% weight in the S&P500. The portfolio with 20% weight in the S&P500. The…
A: To calculate the average return and standard deviation for each portfolio, we'll first assume the…
Q: II. NORMAL PROBABILITY DISTRIBUTION a. The selling prices of home in a certain community is such…
A:
Q: a. What is the standard deviation of lifetimes? b. What is the probability that a component lasts…
A: It is given that the lifetime of a certain component has an exponential distribution with mean λ =…
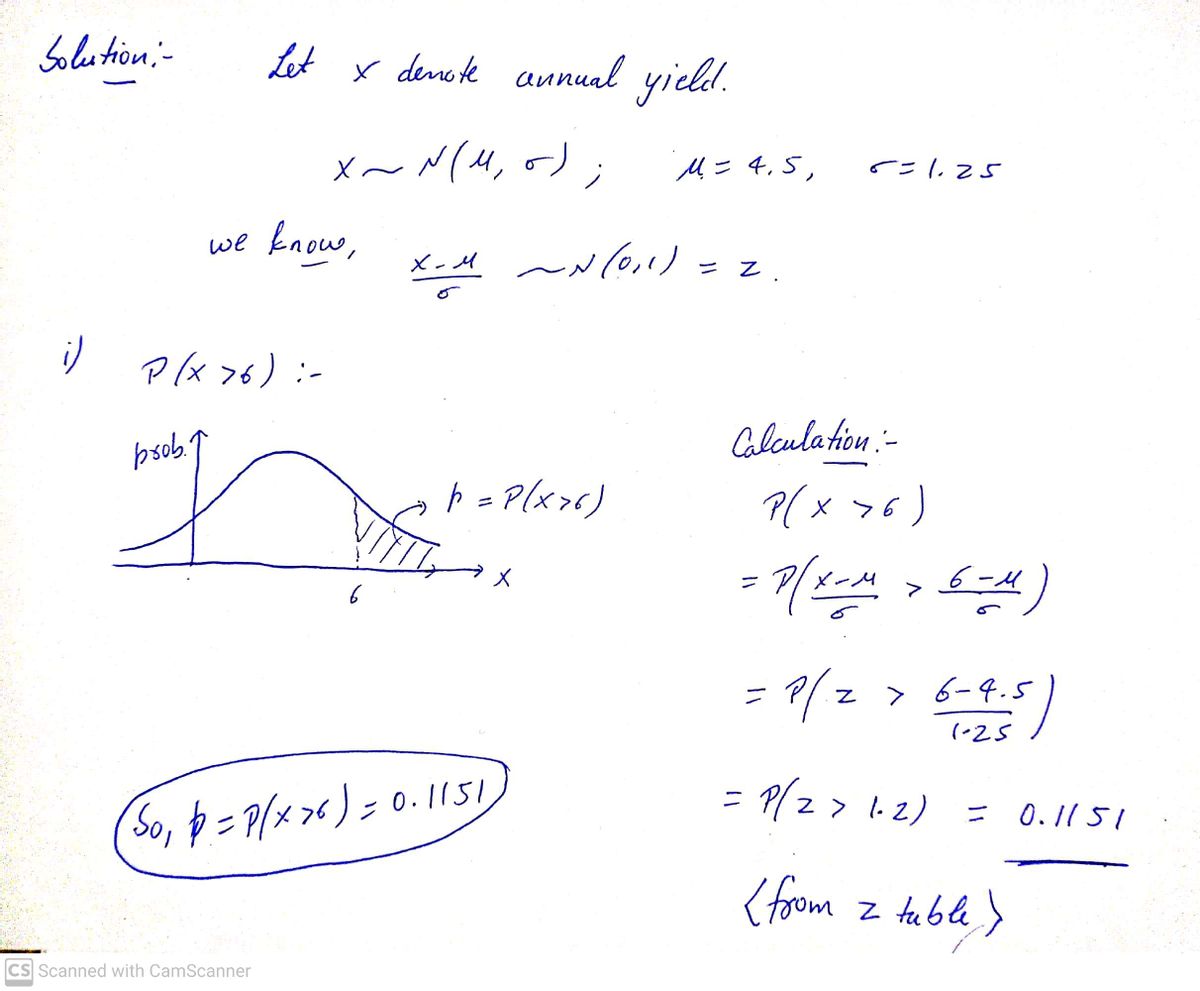
question 2b. The annual yield (expressed as a percentage) for all investments in a particular asset class in the latest financial year was
i. Represent the probability that a randomly selected investment in this class will have an annual yield greater than 6.00%on a diagram and determine this probability.
ii. Represent the value for annual yield that only 10% of investments in this class exceed on a diagram and determine this value.
Step by step
Solved in 2 steps with 2 images

- 1. Use the following to answer questions (a)-(c): The daily sales at a convenience store produce a distribution that is approximately normal with a mean of 1220 and a standard deviation of 130. a. The probability that the sales on a given day at this store are more than $1,405, rounded to four decimal places, is: b. The probability that the sales on a given day at this store are less than $1,305, rounded to four decimal places, is: c. The probability that the sales on a given day at this store are between $1,200 and $1,300, rounded to four decimal places, is:man.21. The amount of time a Tech student spends on Instagram has an approximately exponential distribution with mean 60 minutes. a. What is the probability a randomly selected student spends less than 45 on Instagram? b. Illustrate your answer from a. using the pdf c. Find the cumulative distribution function for the time students spend on Instagram. d. What is the probability a randomly selected student is on Instagram for a time within 2 standard deviation of the mean?
- The figure in the popup window, shows the one-year return distribution for RCS stock. Calculate: a. The expected return. b. The standard deviation of the return. Note: Make sure to round all intermediate calculations to at least five decimal places.Suppose the following data show the introductory interest rates on a sample of 5 credit cards: 6, 2.7, 3.3, 5.6, 3.7. Calculate the standard deviation of the sample. Note: the numbers may be different from the last problem. (please express your answer using 2 decimal places)Consider the following asset with it's returns over the last 3 years. The arithmetic mean is 8% 1yr 2yr 3yr 8% 4% 6% What is the standard deviation? Respuesta:
- Directions: Compute the total returns, the average of returns, and the standard deviation of the following stocks: 1) 2) EGRH Inc. MP, Ltd. AVERAGE OF STOCK RETURN YEA AVERAGE OF RETURNS (x) YEAR STOCK RETURN PRICE (x₁) PRICE RETU Jan-2021 Po Feb-2021 P8.6 Jan-2021 PO. Feb-2021 PO.090 Mar-2021 P0.097 Apr-2021 PO.189 May-2021 PO.164 Mar-2021 P9.14 Apr-2021 P13.30 May-2021 P13 Jun-2021 P60 Jul-2021 16.94 Jun-2021 P0.495 Jul-2021 PO.28 Aug-2021 PO Sep-2021 90 Aug-202 P13.70 Sep-2 P14.88 Oct-2021 0.375 Oct 21 P15.30 Nov-20 PO.325 N2021 P14.30 Dec-2 PO.330 ec-2021 P15.52 3) SD (8) GSM Inc. YEAR Jan-2021 P57. Feb-2021 P52.90 Mar-2021 P50.95 Apr-2021 P58.25 May-2021 P74.05 Jun-2021 P94.75 Jul-2021 P85.0 Aug-2021 P10 Sep-2021 P 00 Oct-2021 01.00 Nov-2021 100.40 Dec-202 P113.80 SD (8) = STOCK RETURN PRICE (x₁) AVERAGE OF RETURNS -x)² SD (8) = ACEE, Inc. YEAR STOCK RETURN PRICE (x₁) Jan-2021 P13.56 Feb-2021 P20.80 Mar-2021 P22.50 Apr-2021 P18.90 May-2021 P17.00 Jun-2021 P18.76 Jul-2021 P16.38…Directions: Compute the total returns, the average of returns, and the standard deviation of the following stocks: 1) 2) EGRH Inc. DMP, Ltd. STOCK RETURN YEA AVERAGE OF RETURNS (x) YEAR STOCK RETURN PRICE AVERAGE OF RETURNS (x) PRICE (x₁) Jan-2021 P8.30 Feb-2021 P8.60 Jan-2021 PO. Feb-2021 PO.090 Mar-2021 P0.097 Apr-2021 PO.189 May-2021 PO.164 Mar-2021 P9.14 Apr-2021 P13.30 May-2021 P13.74 Jun-2021 P14.80 Jul-2021 P16.94 Jun-2021 P0.495 Jul-2021 PO.28 Aug-2021 PO Sep-2021 90 Aug-2021 P13.70 Sep-2021 P14.88 Oct-2021 0.375 Oct-2021 P15.30 Nov-20 PO.325 Nov-2021 P14.30 Dec-2 PO.330 Dec-2021 P15.52 3) SD (8) GSM Inc. STOCK YEAR PRICE Jan-2021 P57.70 Feb-2021 P52.90 Mar-2021 P50.95 Apr-2021 P58.25 May-2021 P74.05 Jun-2021 P94.75 Jul-2021 P85.00 Aug-2021 P105.00 Sep-2021 P114.00 Oct-2021 | P101.00 Nov-2021 P100.40 Dec-2021 P113.80 SD (8) = RETURN (x₁) -x)² AVERAGE OF RETURNS (x-x)² (x) SD (8) = ACEE, Inc. YEAR STOCK RETURN PRICE (x₁) Jan-2021 P13.56 Feb-2021 P20.80 Mar-2021 P22.50 Apr-2021…Suppose that the walking step lengths of adult males are normally distributed with a mean of 2.32 feet and a standard deviation of 0.40 feet. A sample of 83 men’s step lengths is taken. Step 1 of 2 : Find the probability that an individual man’s step length is less than 2.1 feet. Round your answer to 4 decimal places, if necessary.



