The accompanying data represent the number of days absent, x, and the final exam score, y, for a sample of college students in a general education course at a large state university. Absences and Final Exam Scores No. of absences, x 0 1 2 3 4 5 6 7 8 9 Final exam score, y 89.7 86.1 83.6 81.8 78.7 74.1 64.4 71.2 64.5 66.4 Critical Values for Correlation Coefficient n 3 0.997 4 0.950 5 0.878 6 0.811 7 0.754 8 0.707 9 0.666 10 0.632 11 0.602 12 0.576 13 0.553 14 0.532 15 0.514 16 0.497 17 0.482 18 0.468 19 0.456 20 0.444 21 0.433 22 0.423 23 0.413 24 0.404 25 0.396 26 0.388 27 0.381 28 0.374 29 0.367 30 0.361 n (a) The least-squaresregressionline treating number of absences as the explanatory variable and the final exam score as the response variable. y=negative 2.907−2.907x+89.133 (b) Interpret the slope and the y-intercept, if appropriate. Choose the correct answer below and fill in any answer boxes in your choice. Round to 3 decimal places as needed. A. For every additional absence, a student's final exam score drops ___ points, on average. The average final exam score of students who miss no classes is . B. For every additional absence, a student's final exam score drops _ points, on average. It is not appropriate to interpret the y-intercept. C. The average final exam score of students who miss no classes is _______. It is not appropriate to interpret the slope. D. It is not appropriate to interpret the slope or the y-intercept.

MATLAB: An Introduction with Applications

6th Edition

ISBN:9781119256830

Author:Amos Gilat

Publisher:Amos Gilat

Chapter1: Starting With Matlab

Section: Chapter Questions

Problem 1P

See similar textbooks

Related questions

Question

The accompanying data represent the number of days absent, x, and the final exam score, y, for a sample of college students in a general education course at a large state university.

Absences and Final Exam Scores

No. of absences, x	0	1	2	3	4	5	6	7	8	9
Final exam score, y	89.7	86.1	83.6	81.8	78.7	74.1	64.4	71.2	64.5	66.4

Critical Values for Correlation Coefficient
n
3 0.997
4 0.950
5 0.878
6 0.811
7 0.754
8 0.707
9 0.666
10 0.632
11 0.602
12 0.576
13 0.553
14 0.532
15 0.514
16 0.497
17 0.482
18 0.468
19 0.456
20 0.444
21 0.433
22 0.423
23 0.413
24 0.404
25 0.396
26 0.388
27 0.381
28 0.374
29 0.367
30 0.361
n

(a) The least-squaresregressionline treating number of absences as the explanatory variable and the final exam score as the response variable.

y=negative 2.907−2.907x+89.133

(b) Interpret the slope and the y-intercept, if appropriate. Choose the correct answer below and fill in any answer boxes in your choice.

Round to 3 decimal places as needed.

For every additional absence, a student's final exam score drops

_______ points, on average. The average final exam score of students who miss no classes is ________.

For every additional absence, a student's final exam score drops _______ points, on average. It is not appropriate to interpret the y-intercept.

The average final exam score of students who miss no classes is

_________. It is not appropriate to interpret the slope.

It is not appropriate to interpret the slope or the y-intercept.

Expert Solution

(a)

Procedure to conduct regression analysis using Excel:

Enter the data of x and y in an excel sheet.
Go to Data > Data Analysis.
From Analysis Tool, choose Regression and click OK.
In Input Y Range, select the data of Final exam score, y.
In Input X Range, select the data of No. of absences, x.
Click OK.

Output:

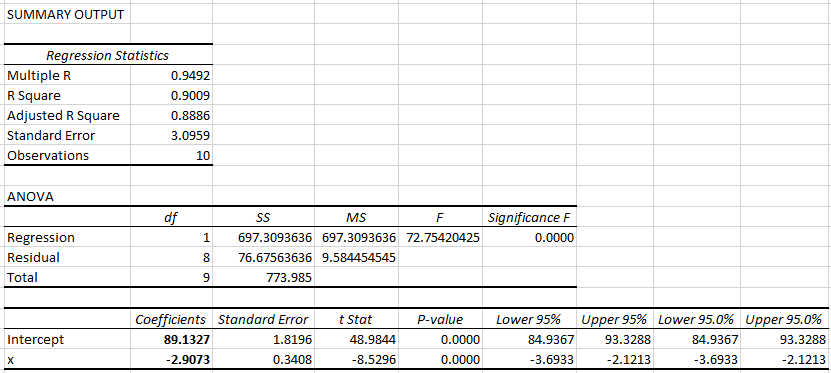
From the output, the least-squares regression line treating number of absences as the explanatory variable and the final exam score as the response variable is $y = - 2.907 x + 89.133$ .