The 30 students in MATH215A are invited to play “My Deal or NO DEAL!!” Each student will get to select one of 25 cases prior to any being opened. (It’s perfectly acceptable for two or more people to select the same case). After the cases have been selected I, as the host, will start opening the cases one by one eliminating those who didn’t select the case with the $1,000,000. If exactly 4 people selected the million dollar case, everyone will share the spoils. What is the probability that exactly 4 of you will select the correct case?
Contingency Table
A contingency table can be defined as the visual representation of the relationship between two or more categorical variables that can be evaluated and registered. It is a categorical version of the scatterplot, which is used to investigate the linear relationship between two variables. A contingency table is indeed a type of frequency distribution table that displays two variables at the same time.
Binomial Distribution
Binomial is an algebraic expression of the sum or the difference of two terms. Before knowing about binomial distribution, we must know about the binomial theorem.
The 30 students in MATH215A are invited to play “My Deal or NO DEAL!!” Each student will get to
select one of 25 cases prior to any being opened. (It’s perfectly acceptable for two or more people to
select the same case). After the cases have been selected I, as the host, will start opening the cases one
by one eliminating those who didn’t select the case with the $1,000,000. If exactly 4 people selected
the million dollar case, everyone will share the spoils. What is the
will select the correct case?
Given :
The 30 students in MATH215A are invited to play “My Deal or NO DEAL!!” Each student will get to
select one of 25 cases prior to any being opened. (It’s perfectly acceptable for two or more people to
select the same case). After the cases have been selected I, as the host, will start opening the cases one
by one eliminating those who didn’t select the case with the $1,000,000. If exactly 4 people selected
the million dollar case, everyone will share the spoils.
Play has two outcomes “My Deal or NO DEAL" and Each student will get to select one of 25 cases prior to any being opened.
Therefore , Probability of success = 1/25 = 0.04
30 students in MATH215A are invited to play .
Therefore , n = 30
Let, X be the number of people selected the million dollar case, everyone will share the spoils.
Here , X ~> B(n=30,p=0.04)
Binomial probability distribution function (PDF) is given as :
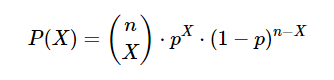
Step by step
Solved in 2 steps with 2 images









