Suppose (t + 4) y { (t — 6)y/ - = = P(t) a. This system of linear differential equations can be put in the form ÿ' = P(t)ÿ + ģ(t). Determine P(t) and ģ(t). 18 (8) = 8ty₁ + 7y2, 3y1 + 8ty2, g(t) = y₁ (1) = 0, y2 (1) = 2. b. Is the system homogeneous or nonhomogeneous? Choose Interval: help (inequalities) c. Find the largest interval a < t < b such that a unique solution of the initial value problem is guaranteed to exist.
Suppose (t + 4) y { (t — 6)y/ - = = P(t) a. This system of linear differential equations can be put in the form ÿ' = P(t)ÿ + ģ(t). Determine P(t) and ģ(t). 18 (8) = 8ty₁ + 7y2, 3y1 + 8ty2, g(t) = y₁ (1) = 0, y2 (1) = 2. b. Is the system homogeneous or nonhomogeneous? Choose Interval: help (inequalities) c. Find the largest interval a < t < b such that a unique solution of the initial value problem is guaranteed to exist.
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
![**Suppose**
\[
(t + 4) y_1' = 8ty_1 + 7y_2, \quad y_1(1) = 0,
\]
\[
(t - 6) y_2' = 3y_1 + 8ty_2, \quad y_2(1) = 2.
\]
**a.** This system of linear differential equations can be put in the form \(\vec{y}' = P(t)\vec{y} + \vec{g}(t)\). Determine \(P(t)\) and \(\vec{g}(t)\).
\[
P(t) = \begin{bmatrix}
\phantom{00} & \phantom{00} \\
\phantom{00} & \phantom{00}
\end{bmatrix}
\]
\[
\vec{g}(t) = \begin{bmatrix}
\phantom{00} \\
\phantom{00}
\end{bmatrix}
\]
**b.** Is the system homogeneous or nonhomogeneous? [Choose dropdown box]
**c.** Find the largest interval \(a < t < b\) such that a unique solution of the initial value problem is guaranteed to exist.
Interval: [ ] [Help (inequalities)]](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F6890296c-2fc0-4a83-b66a-ee1a85d807a1%2F3bd85f84-7ecd-446d-b68d-f4e962bd7aa0%2Fvlz1rp_processed.jpeg&w=3840&q=75)
Transcribed Image Text:**Suppose**
\[
(t + 4) y_1' = 8ty_1 + 7y_2, \quad y_1(1) = 0,
\]
\[
(t - 6) y_2' = 3y_1 + 8ty_2, \quad y_2(1) = 2.
\]
**a.** This system of linear differential equations can be put in the form \(\vec{y}' = P(t)\vec{y} + \vec{g}(t)\). Determine \(P(t)\) and \(\vec{g}(t)\).
\[
P(t) = \begin{bmatrix}
\phantom{00} & \phantom{00} \\
\phantom{00} & \phantom{00}
\end{bmatrix}
\]
\[
\vec{g}(t) = \begin{bmatrix}
\phantom{00} \\
\phantom{00}
\end{bmatrix}
\]
**b.** Is the system homogeneous or nonhomogeneous? [Choose dropdown box]
**c.** Find the largest interval \(a < t < b\) such that a unique solution of the initial value problem is guaranteed to exist.
Interval: [ ] [Help (inequalities)]
Expert Solution
Step 1: Introduction
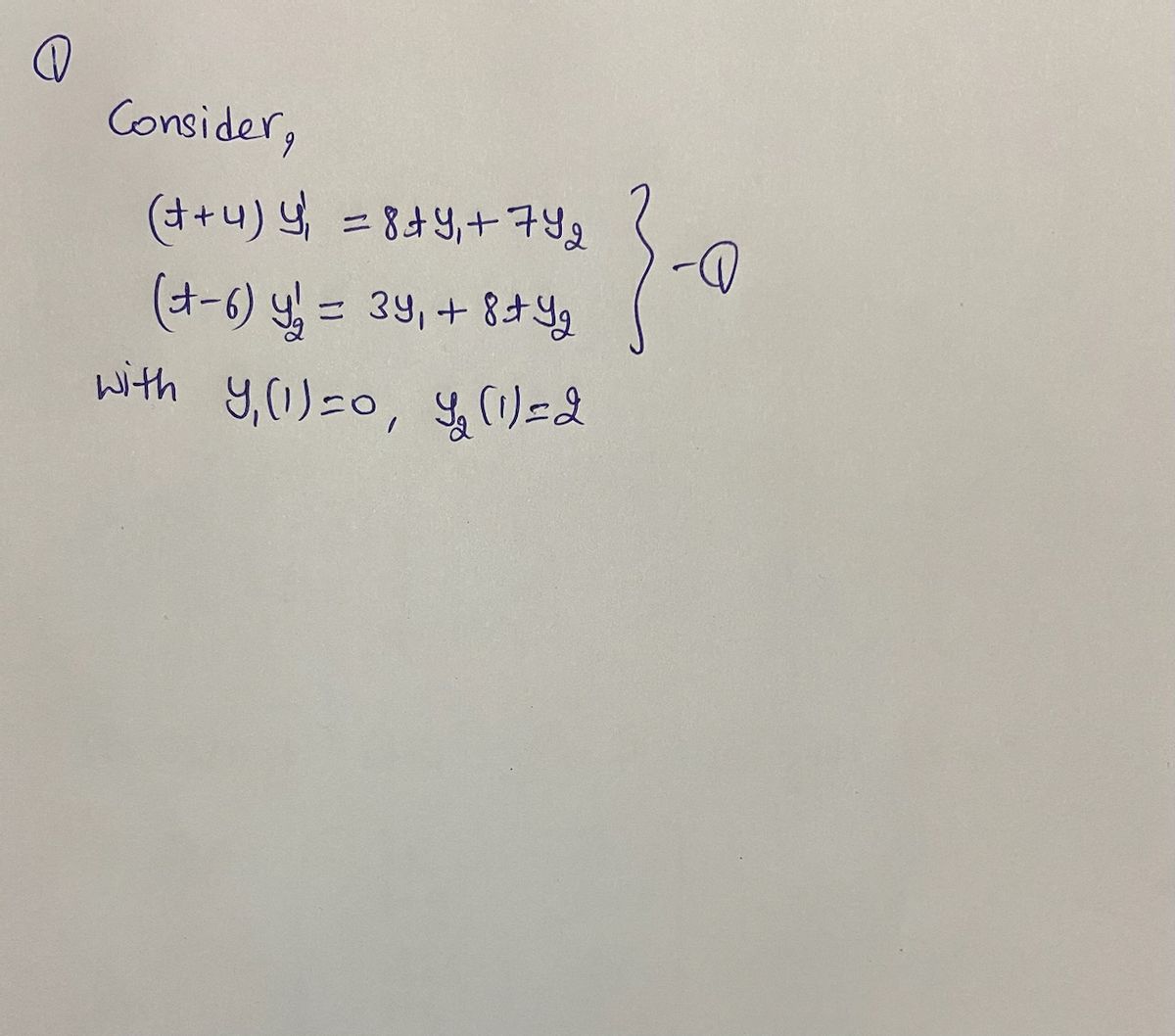
Step by step
Solved in 4 steps with 4 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

