5. Looking at your graph, do you think bagworm number is even among the sites? 6. When thinking about the categories associated with the sites (sites 1 and 5 are very close to junipers, sites 2 and 4 are < 100 m from junipers, site 3 is > 100 m from junipers), do you think there could be support for the hypothesis that distance to junipers might be influencing bagworm number on the trees in each site? Why or why not? 7. Now we will run an actual stat test to see if we can reject our null hypothesis. In order to know whether or not our null is supported, we will compare the number of bagworms we observed at each site to the number we would expect to see at each site, assuming each site has an equal number, given its surface area (m²). Because our sites were not all the same size, I have calculated the expected values and adjusted them according to surface area for each site. These expected values are listed for you in column K. Place your observed values from column G in column L for each site (paste special as values) and run an appropriate statistical test to determine whether what you observed was different from what you expected (use a one-tailed test for this). Run your test using the Basic Statistics Calculator. Copy the output to this tab and paste special as values. Make sure your output is labeled. Questions to answer about the statistical test: 8. Which inferential statistical test did you use? 9. What are your degrees of freedom (df) for this test? 10. What is the value of your test statistic (you can round to the nearest 100th place)? 11. What is the critical value, assuming a one-tailed test? 12. Was the null hypothesis supported or rejected and why? 13. What does this result tell you?
5. Looking at your graph, do you think bagworm number is even among the sites? 6. When thinking about the categories associated with the sites (sites 1 and 5 are very close to junipers, sites 2 and 4 are < 100 m from junipers, site 3 is > 100 m from junipers), do you think there could be support for the hypothesis that distance to junipers might be influencing bagworm number on the trees in each site? Why or why not? 7. Now we will run an actual stat test to see if we can reject our null hypothesis. In order to know whether or not our null is supported, we will compare the number of bagworms we observed at each site to the number we would expect to see at each site, assuming each site has an equal number, given its surface area (m²). Because our sites were not all the same size, I have calculated the expected values and adjusted them according to surface area for each site. These expected values are listed for you in column K. Place your observed values from column G in column L for each site (paste special as values) and run an appropriate statistical test to determine whether what you observed was different from what you expected (use a one-tailed test for this). Run your test using the Basic Statistics Calculator. Copy the output to this tab and paste special as values. Make sure your output is labeled. Questions to answer about the statistical test: 8. Which inferential statistical test did you use? 9. What are your degrees of freedom (df) for this test? 10. What is the value of your test statistic (you can round to the nearest 100th place)? 11. What is the critical value, assuming a one-tailed test? 12. Was the null hypothesis supported or rejected and why? 13. What does this result tell you?
MATLAB: An Introduction with Applications
6th Edition
ISBN:9781119256830
Author:Amos Gilat
Publisher:Amos Gilat
Chapter1: Starting With Matlab
Section: Chapter Questions
Problem 1P
Related questions
Question

Transcribed Image Text:**Questions to answer about the graph:**
5. Looking at your graph, do you think bagworm number is even among the sites?
6. When thinking about the categories associated with the sites (sites 1 and 5 are very close to junipers, sites 2 and 4 are < 100 m from junipers, site 3 is > 100 m from junipers), do you think there could be support for the hypothesis that distance to junipers might be influencing bagworm number on the trees in each site? Why or why not?
7. Now we will run an actual stat test to see if we can reject our null hypothesis. In order to know whether or not our null is supported, we will compare the number of bagworms we observed at each site to the number we would expect to see at each site, assuming each site has an equal number, given its surface area (m²). Because our sites were not all the same size, I have calculated the expected values and adjusted them according to surface area for each site. These expected values are listed for you in column K. Place your observed values from column G in column L for each site (paste special as values) and run an appropriate statistical test to determine whether what you observed was different from what you expected (use a one-tailed test for this). Run your test using the Basic Statistics Calculator. Copy the output to this tab and paste special as values. Make sure your output is labeled.
**Questions to answer about the statistical test:**
8. Which inferential statistical test did you use?
9. What are your degrees of freedom (df) for this test?
10. What is the value of your test statistic (you can round to the nearest 100th place)?
11. What is the critical value, assuming a one-tailed test?
12. Was the null hypothesis supported or rejected and why?
13. What does this result tell you?

Transcribed Image Text:### Bagworm Data Analysis
#### Data Summary
The table consists of data collected on the presence of bagworms across different sites. Here's a detailed breakdown:
- **Bagworm Sightings (Y/N):** Indicates whether bagworms were observed (Y) or not (N).
- **Bagworm #:** Number of bagworms spotted.
- **Site Distribution:** Data is categorized based on five different sites.
- **% Bagworms:** Percentage of total bagworms found at each site.
#### Site Details
- **Site 1:**
- Bagworms: 414
- % Bagworms: 9.43%
- Expected: 338.0033
- Observed: 414
- **Site 2:**
- Bagworms: 863
- % Bagworms: 19.66%
- Expected: 929.5875
- Observed: 863
- **Site 3:**
- Bagworms: 101
- % Bagworms: 2.30%
- Expected: 375.8202
- Observed: 101
- **Site 4:**
- Bagworms: 1050
- % Bagworms: 23.92%
- Expected: 1335.055
- Observed: 1050
- **Site 5:**
- Bagworms: 1961
- % Bagworms: 44.68%
- Expected: 1414.534
- Observed: 1961
#### Total Data:
- Total Bagworms observed: 4389
- Combined percentage: 100%
#### Pie Chart Explanation
- **Title:** The Sites of Bagworm
- **Segments:**
- Blue (Site 1) - 9%
- Orange (Site 2) - 20%
- Grey (Site 3) - 2%
- Yellow (Site 4) - 24%
- Light Blue (Site 5) - 45%
The pie chart visually represents the distribution of bagworms across the five sites. Site 5 displays the largest percentage at 45%, indicating a significant concentration of bagworms compared to other locations.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by step
Solved in 4 steps with 1 images

Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
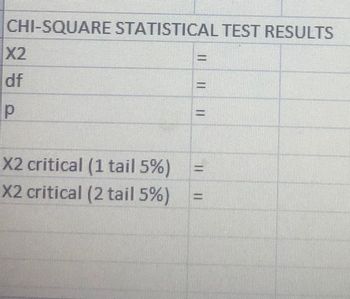
Transcribed Image Text:CHI-SQUARE STATISTICAL TEST RESULTS
X2
df
р
X2 critical (1 tail 5%)
X2 critical (2 tail 5%)
Solution
Recommended textbooks for you

MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc

Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning

Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning

MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc

Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning

Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning

Elementary Statistics: Picturing the World (7th E…
Statistics
ISBN:
9780134683416
Author:
Ron Larson, Betsy Farber
Publisher:
PEARSON

The Basic Practice of Statistics
Statistics
ISBN:
9781319042578
Author:
David S. Moore, William I. Notz, Michael A. Fligner
Publisher:
W. H. Freeman

Introduction to the Practice of Statistics
Statistics
ISBN:
9781319013387
Author:
David S. Moore, George P. McCabe, Bruce A. Craig
Publisher:
W. H. Freeman