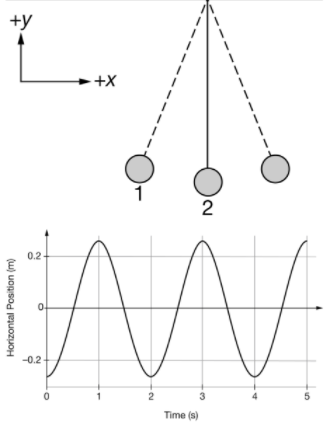
One end of a string is secured to the ceiling of a classroom, and the other end of the string is attached to a sphere of mass 0.2 kg. The sphere is raised at an angle of 15o above the sphere’s equilibrium position and then released from rest so that the pendulum oscillates, as shown in the figure. Location 1 is shown in the figure. A graph of the pendulum’s horizontal position as a function of time is shown in the graph. A)The dot below represents the sphere at location 2. Draw a free-body diagram that shows and labels the forces (not components) exerted on the sphere at the location. Draw the relative lengths of all vectors to reflect the relative magnitudes of all forces. B) Calculate the maximum kinetic energy of the sphere as it oscillates. C) The sphere is raised to a new angle above the sphere’s equilibrium position that is less than the angle described for part (a). The sphere is released from rest so that the pendulum oscillates. (c) Explain how the motion of the sphere changes, if at all, based on the appropriate evidence.
Simple harmonic motion
Simple harmonic motion is a type of periodic motion in which an object undergoes oscillatory motion. The restoring force exerted by the object exhibiting SHM is proportional to the displacement from the equilibrium position. The force is directed towards the mean position. We see many examples of SHM around us, common ones are the motion of a pendulum, spring and vibration of strings in musical instruments, and so on.
Simple Pendulum
A simple pendulum comprises a heavy mass (called bob) attached to one end of the weightless and flexible string.
Oscillation
In Physics, oscillation means a repetitive motion that happens in a variation with respect to time. There is usually a central value, where the object would be at rest. Additionally, there are two or more positions between which the repetitive motion takes place. In mathematics, oscillations can also be described as vibrations. The most common examples of oscillation that is seen in daily lives include the alternating current (AC) or the motion of a moving pendulum.
One end of a string is secured to the ceiling of a classroom, and the other end of the string is attached to a sphere of mass 0.2 kg. The sphere is raised at an angle of 15o above the sphere’s equilibrium position and then released from rest so that the pendulum oscillates, as shown in the figure. Location 1 is shown in the figure. A graph of the pendulum’s horizontal position as a function of time is shown in the graph.
A)The dot below represents the sphere at location 2. Draw a free-body diagram that shows and labels the forces (not components) exerted on the sphere at the location. Draw the relative lengths of all vectors to reflect the relative magnitudes of all forces.
B) Calculate the maximum kinetic energy of the sphere as it oscillates.
(c) Explain how the motion of the sphere changes, if at all, based on the appropriate evidence.


Given :
Mass of the sphere, m = 0.2 kg
Angle raised from its equilibrium,
Trending now
This is a popular solution!
Step by step
Solved in 4 steps with 3 images









