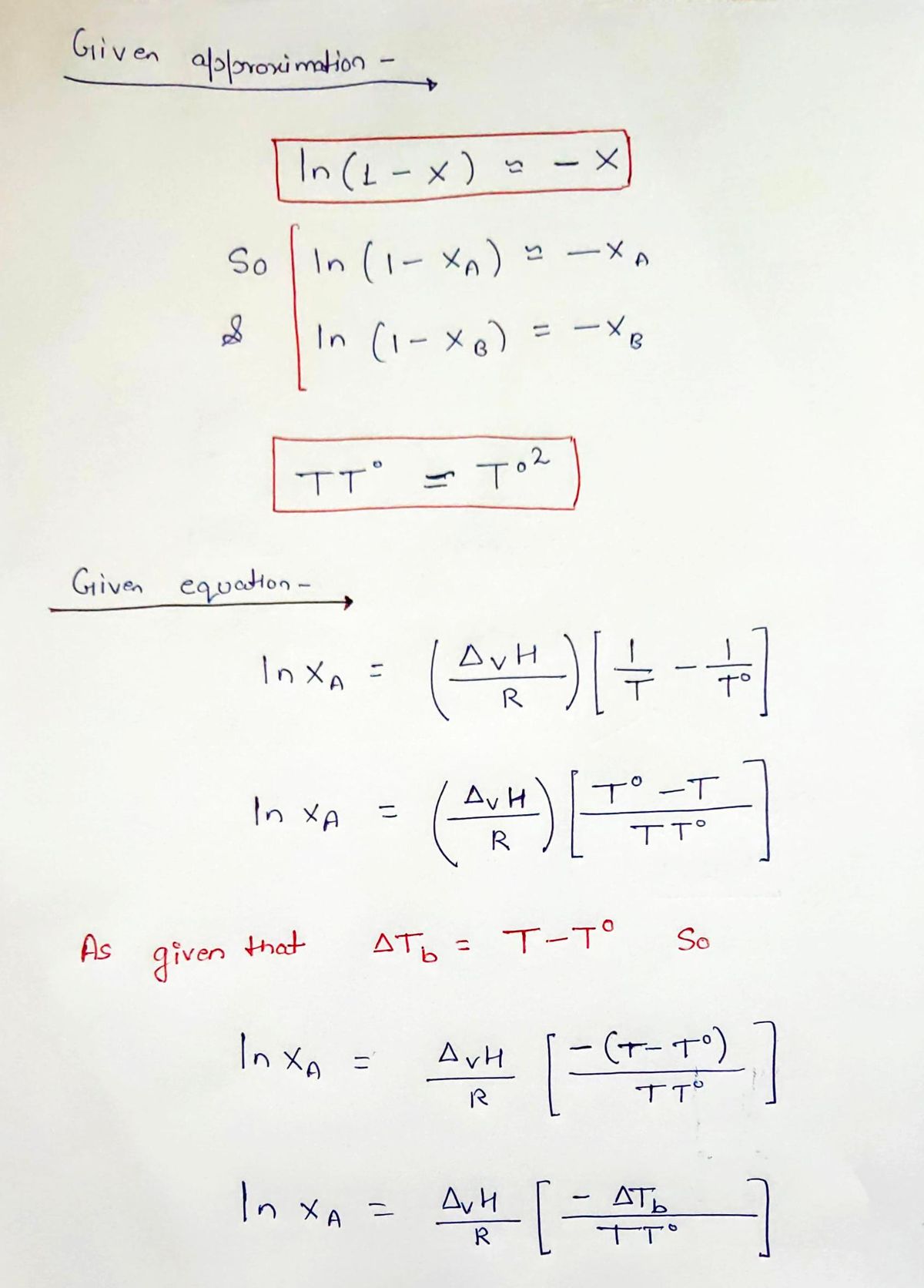
In class, we derived the expression: ln XA =(ΔvH/R) ((1/T)-(1/T°)) where A represents the solvent (and B would represent the solute, such that XA + XB = 1). Using the approximations given below, derive the expression: ∆Tb = XB (RT°2/ΔvH) where ∆Tb is the change in boiling point between the solution and the pure liquid, T − T°. Approximations: ln(1 − X) ≈ −X TT° ≈ T°2
In class, we derived the expression:
ln XA =(ΔvH/R) ((1/T)-(1/T°))
where A represents the solvent (and B would represent the solute, such that XA + XB = 1).
Using the approximations given below, derive the expression:
∆Tb = XB (RT°2/ΔvH)
where ∆Tb is the change in boiling point between the solution and the pure liquid, T − T°.
Approximations:
ln(1 − X) ≈ −X
TT° ≈ T°2
Note: this derived expression is often simplified in General Chemistry as ∆Tb = Kbm where Kb is the boiling point elevation constant, and m is the molality.
Interpretation-
To drive the expression which is given as ∆Tb = XB (RT°2/ΔvH) by using the equation ln XA =(ΔvH/R) ((1/T)-(1/T°)).
Concept-
As here already given that equation is -
ln XA =(ΔvH/R) ((1/T)-(1/T°))
here A represents the solvent and B would represent the solute, such that XA + XB = 1
∆Tb is the change in boiling point between the solution and the pure liquid, T − T° , so ∆Tb = T - To
Step by step
Solved in 4 steps with 3 images









