Identify the P-value. (Round to three decimal places as needed.) State the final conclusion that addresses the original claim. Ho. There is V evidence to warrant rejection of the claim that months of births of baseball players are independent of whether they are born in America.


In this scenario, the aim is to check whether there is sufficient evidence to warrant rejection of the claim that months of births of baseball players are independent of whether they are born in America.
Hypotheses:
The null hypothesis is:
H0 : Months of births of baseball players are independent of where they are born.
The alternative hypothesis is:
H1 : Months of births of baseball players are dependent of where they are born.
Calculation steps:
The calculations have been done in EXCEL.
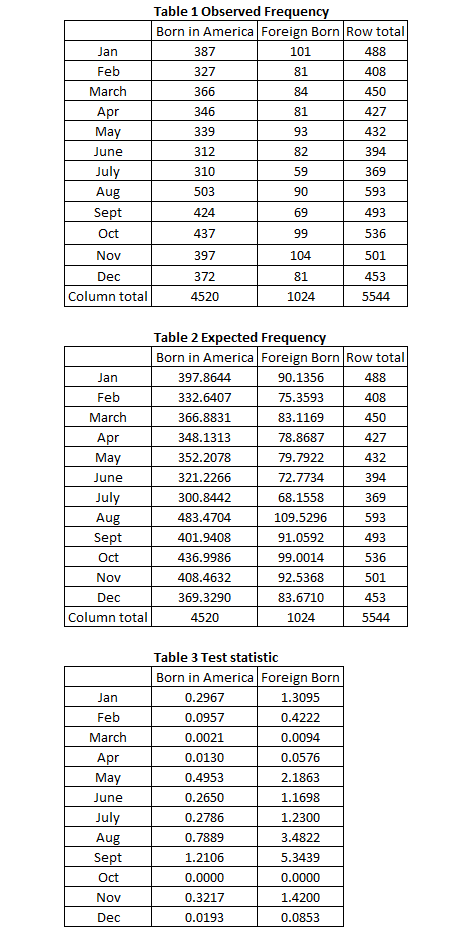
Denote oij as the observed frequency for ith row and jth column (i =1, 2, …, 12; j =1, 2) and eij as the expected frequency for ith row and jth column.
Table 1 provides the data values, oij.
Table 2 calculates the expected frequencies under the independence assumption. If independent, eij = (oi∙) ∙ (o∙j) / N. [o∙j = column total of jth column, N = grand total of all observations = 5544]. So, the first cell expected frequency will be e11 = (4520) ∙ (488) / 5544= 397.8644. Similarly, the others can be calculated.
Table 3:Test statistic:
The formula for the test statistic is χ2 = ∑ [(oij – eij)2 / eij], summed over all i and j.
Table 4 calculates [(oij – eij)2 / eij] for each (i, j). So, the value in the first cell will be (387– 397.8644)2 /397.8644≈ 0.2967.
The test statistic value can be calculated by adding all these cell values.
Step by step
Solved in 3 steps with 1 images









