College Physics
11th Edition
ISBN:9781305952300
Author:Raymond A. Serway, Chris Vuille
Publisher:Raymond A. Serway, Chris Vuille
Chapter1: Units, Trigonometry. And Vectors
Section: Chapter Questions
Problem 1CQ: Estimate the order of magnitude of the length, in meters, of each of the following; (a) a mouse, (b)...
Related questions
Question
I dont know how to do this
![**Question 3: The Electric Field of a Dipole Far from the Dipole in Spherical Coordinates**
In class, we found \( V \) far from the dipole in spherical coordinates. Here, you will use the gradient in spherical coordinates to calculate the electric field from the potential. The results are easier to analyze than the expressions obtained in class in Cartesian coordinates.
The dipole is along the z-axis and the point of interest is over the z-axis where the azimuthal angle, \( \phi = 0^\circ \). This is why our potential is only a function of \( r \) and \( \theta \).
**Equation:**
\[
V = \frac{k p \cos \theta}{r^2}
\]
**Electric Field Components in Spherical Coordinates:**
\[
E_r = -\frac{\partial V}{\partial r}
\]
\[
E_\theta = -\frac{1}{r} \frac{\partial V}{\partial \theta}
\]
These equations are used to determine the components of the electric field, \( E_r \) and \( E_\theta \), in spherical coordinates.
There is no graph or diagram that needs further explanation in this image.](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F249fe9e2-5f1e-4e39-9ada-88c965da2dda%2Fbb8a2d79-be9a-4b80-aabc-0aab5956a5bf%2Fv5n1q9s.jpeg&w=3840&q=75)
Transcribed Image Text:**Question 3: The Electric Field of a Dipole Far from the Dipole in Spherical Coordinates**
In class, we found \( V \) far from the dipole in spherical coordinates. Here, you will use the gradient in spherical coordinates to calculate the electric field from the potential. The results are easier to analyze than the expressions obtained in class in Cartesian coordinates.
The dipole is along the z-axis and the point of interest is over the z-axis where the azimuthal angle, \( \phi = 0^\circ \). This is why our potential is only a function of \( r \) and \( \theta \).
**Equation:**
\[
V = \frac{k p \cos \theta}{r^2}
\]
**Electric Field Components in Spherical Coordinates:**
\[
E_r = -\frac{\partial V}{\partial r}
\]
\[
E_\theta = -\frac{1}{r} \frac{\partial V}{\partial \theta}
\]
These equations are used to determine the components of the electric field, \( E_r \) and \( E_\theta \), in spherical coordinates.
There is no graph or diagram that needs further explanation in this image.
Expert Solution
Step 1
Consider the dipole placed at origin aligned along Z-axis, with two point charges of +q and -q, positioned at ⟨z = ± a/2⟩
By symmetry the potential is independent of azimuthal angle because our dipole is a point dipole then (r >> a).
The distance of this point to the two charges is given by;
± r² = [ (a²/4) + r² ± (ar Cos θ) ]
The potential of this point is given by,
V (r,θ) = (q/4πε [a²/4+ r² ±(arCosθ)]⁻¹/²) - (a²/4 + r² + arCosθ)⁻¹/²
Taylor expansion gives,
V(r,θ ) = [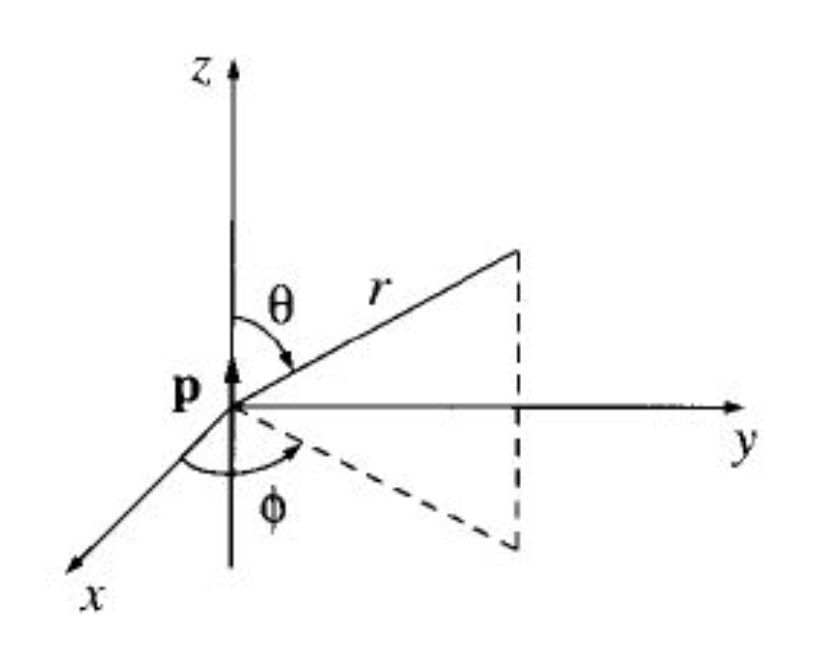
qa Cosθ /4πεr²]
Step by step
Solved in 2 steps with 1 images
Recommended textbooks for you
College Physics
Physics
ISBN:
9781305952300
Author:
Raymond A. Serway, Chris Vuille
Publisher:
Cengage Learning
University Physics (14th Edition)
Physics
ISBN:
9780133969290
Author:
Hugh D. Young, Roger A. Freedman
Publisher:
PEARSON
Introduction To Quantum Mechanics
Physics
ISBN:
9781107189638
Author:
Griffiths, David J., Schroeter, Darrell F.
Publisher:
Cambridge University Press
College Physics
Physics
ISBN:
9781305952300
Author:
Raymond A. Serway, Chris Vuille
Publisher:
Cengage Learning
University Physics (14th Edition)
Physics
ISBN:
9780133969290
Author:
Hugh D. Young, Roger A. Freedman
Publisher:
PEARSON
Introduction To Quantum Mechanics
Physics
ISBN:
9781107189638
Author:
Griffiths, David J., Schroeter, Darrell F.
Publisher:
Cambridge University Press
Physics for Scientists and Engineers
Physics
ISBN:
9781337553278
Author:
Raymond A. Serway, John W. Jewett
Publisher:
Cengage Learning
Lecture- Tutorials for Introductory Astronomy
Physics
ISBN:
9780321820464
Author:
Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina Brissenden
Publisher:
Addison-Wesley
College Physics: A Strategic Approach (4th Editio…
Physics
ISBN:
9780134609034
Author:
Randall D. Knight (Professor Emeritus), Brian Jones, Stuart Field
Publisher:
PEARSON