Consider a non-relativistic particle confined in a rectangular box of side Lr, Ly, and La, whose energy levels are given, as usual, by 器。) E, = En,ny,n. 2m L2 (this is the same as in problem 1 of Problem Set 2, but with the three sides of the box not necessarily equal).
Consider a non-relativistic particle confined in a rectangular box of side Lr, Ly, and La, whose energy levels are given, as usual, by 器。) E, = En,ny,n. 2m L2 (this is the same as in problem 1 of Problem Set 2, but with the three sides of the box not necessarily equal).
Related questions
Question
100%
Unsure on how to approach this problem!
![Consider a non-relativistic particle confined in a rectangular box of side Lr, Ly, and Lr,
whose energy levels are given, as usual, by
E, = Ensny,n:
2m
(this is the same as in problem 1 of Problem Set 2, but with the three sides of the box not
necessarily equal).
1
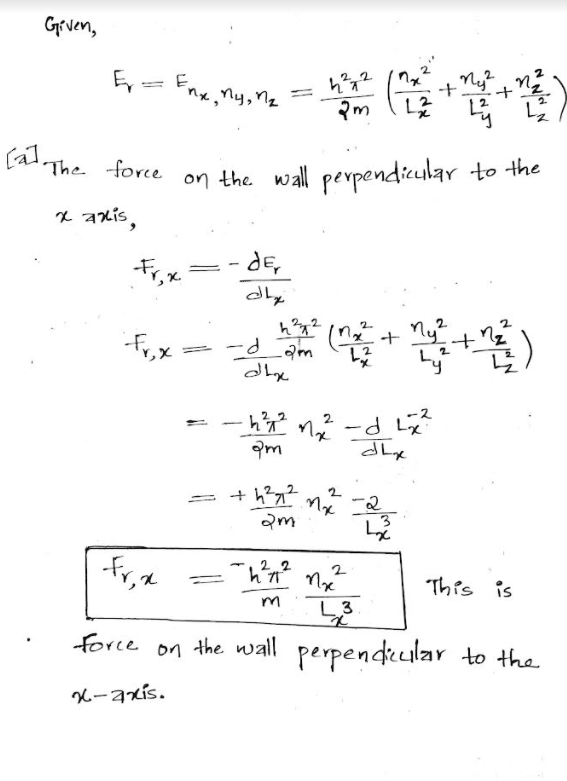
(a) Let the particle be in a given state r specified by particular values of the three integers
na, ny, and n.. Find the force on the wall perpendicular to the r-axis
aE,
and calculate from this the pressure p: (force per unit area) on this wall.
(b) Express the sum p, + Py + P: for any state in terms of the volume V = L,L,L, and the
energy E, of that state.
(c) Show that the mean pressure i exerted by the particle in equilibrium is related to its
mean energy E by
3V
[Hint: Use the result of part (b) and the fact that, in equilibrium, the mean pressure on
every wall is the same.)
(d) Show that if the length of the box is increased adiabatically and quasistatically from L.
to 8Lz, the work W done by the particle is 3/4 of the initial mean energy Enit. (Hint:
convert dW = pdV to a differential equation involving only E and V.]
(e) Show that an attempt to calculate the work done by averaging the finite energy change in
cach state resulting from an increase of length from L, to 8Lz over the initial distribution
of values of n, ny, and n, leads to the incorrect result W = (21/64)Enit. Explain clearly
why this method is not an appropriate description of a quasistatic process.](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F424c3c55-9c99-4f24-bee3-85bf667e2f9d%2Fdfb62c13-c29f-486e-9501-4bd11423444e%2Fw8fk1pp_processed.png&w=3840&q=75)
Transcribed Image Text:Consider a non-relativistic particle confined in a rectangular box of side Lr, Ly, and Lr,
whose energy levels are given, as usual, by
E, = Ensny,n:
2m
(this is the same as in problem 1 of Problem Set 2, but with the three sides of the box not
necessarily equal).
1
(a) Let the particle be in a given state r specified by particular values of the three integers
na, ny, and n.. Find the force on the wall perpendicular to the r-axis
aE,
and calculate from this the pressure p: (force per unit area) on this wall.
(b) Express the sum p, + Py + P: for any state in terms of the volume V = L,L,L, and the
energy E, of that state.
(c) Show that the mean pressure i exerted by the particle in equilibrium is related to its
mean energy E by
3V
[Hint: Use the result of part (b) and the fact that, in equilibrium, the mean pressure on
every wall is the same.)
(d) Show that if the length of the box is increased adiabatically and quasistatically from L.
to 8Lz, the work W done by the particle is 3/4 of the initial mean energy Enit. (Hint:
convert dW = pdV to a differential equation involving only E and V.]
(e) Show that an attempt to calculate the work done by averaging the finite energy change in
cach state resulting from an increase of length from L, to 8Lz over the initial distribution
of values of n, ny, and n, leads to the incorrect result W = (21/64)Enit. Explain clearly
why this method is not an appropriate description of a quasistatic process.
Expert Solution
Step 1
Since you have asked multiple subparts question, I am answering first 3 subparts. Please resubmit the question if you need answers of the other subparts as well and mention the required subparts.
Trending now
This is a popular solution!
Step by step
Solved in 3 steps with 3 images
