Homework Help is Here – Start Your Trial Now!
Science
Advanced Physics
The spin operator in an arbitrary direction can be written as (0,0) = sin cos o + y sin sin + ₂ cos 0, I where the Pauli spin matrices are given by 0 (13). 10 1. Find the eigenvectors and eigenvalues for the operator ô(0, 0). ÔT x 0 av = (15'). Oy i 0 = ôz = 0 0 -1

The spin operator in an arbitrary direction can be written as (0,0) = sin cos o + y sin sin + ₂ cos 0, I where the Pauli spin matrices are given by 0 (13). 10 1. Find the eigenvectors and eigenvalues for the operator ô(0, 0). ÔT x 0 av = (15'). Oy i 0 = ôz = 0 0 -1

icon
Related questions
Question
The spin operator in an arbitrary direction can be written as (0,0) = sin cos o + y sin sin + ₂ cos 0, I where the Pauli spin matrices are given by 0 (13), 1. Find the eigenvectors and eigenvalues for the operator ô(0, 6). ÔT x = Oy = 0 (² i). i 0 Oz = 0 0 -1
Transcribed Image Text:The spin operator in an arbitrary direction can be written as (0,0) = sin cos o + y sin sin + ₂ cos 0, I where the Pauli spin matrices are given by 0 (13), 1. Find the eigenvectors and eigenvalues for the operator ô(0, 6). ÔT x = Oy = 0 (² i). i 0 Oz = 0 0 -1
2. Choose = 0 and find for an entangled state of the form 0 0 |I) >- (B),B),-),B)) 1/12 = 2 1 0 1 0 the probability of detecting particle 1 in spin-up with respect to an angle 0₁ and at the same time particle 2 in spin-up with respect to an angle 02.
Transcribed Image Text:2. Choose = 0 and find for an entangled state of the form 0 0 |I) >- (B),B),-),B)) 1/12 = 2 1 0 1 0 the probability of detecting particle 1 in spin-up with respect to an angle 0₁ and at the same time particle 2 in spin-up with respect to an angle 02.
Expert Solution
trending now

Trending now

This is a popular solution!

steps

Step by step

Solved in 3 steps with 2 images

SEE SOLUTIONCheck out a sample Q&A here
Blurred answer
Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question

please solve the second part

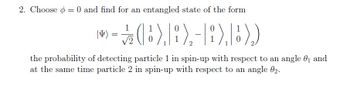
2. Choose = 0 and find for an entangled state of the form 0 0 |I) >- (B),B),-),B)) 1/12 = 2 1 0 1 0 the probability of detecting particle 1 in spin-up with respect to an angle 0₁ and at the same time particle 2 in spin-up with respect to an angle 02.
Transcribed Image Text:2. Choose = 0 and find for an entangled state of the form 0 0 |I) >- (B),B),-),B)) 1/12 = 2 1 0 1 0 the probability of detecting particle 1 in spin-up with respect to an angle 0₁ and at the same time particle 2 in spin-up with respect to an angle 02.
Solution
Bartleby Expert
SEE SOLUTION