Bruce's Material Company hauls gravel to a construction site, using a small truck and a large truck. The carrying capacity and operating cost per load are given in the accompanying table. Bruce must deliver a minimum of 300 cubic yards per day to satisfy his contract with the builder. The union contract with his drivers requires that the total number of loads per day is a minimum of 7. **How many loads should be made in each truck per day to minimize the total cost?** | | Small Truck | Large Truck | |------------------|-------------|-------------| | Capacity (yd³) | 50 | 60 | | Cost per Load | $72 | $54 | In order to minimize the total cost, the number of loads in a small truck that should be made is [blank], and the number of loads in a large truck that should be made is [blank].
![Bruce's Material Company hauls gravel to a construction site, using a small truck and a large truck. The carrying capacity and operating cost per load are given in the accompanying table. Bruce must deliver a minimum of 300 cubic yards per day to satisfy his contract with the builder. The union contract with his drivers requires that the total number of loads per day is a minimum of 7.
**How many loads should be made in each truck per day to minimize the total cost?**
| | Small Truck | Large Truck |
|------------------|-------------|-------------|
| Capacity (yd³) | 50 | 60 |
| Cost per Load | $72 | $54 |
In order to minimize the total cost, the number of loads in a small truck that should be made is [blank], and the number of loads in a large truck that should be made is [blank].](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F312de20a-253d-4366-a119-2f34c19d25d5%2F8ad6ec72-23e0-4468-81f9-71b268f4dc79%2Ftm3vbga.jpeg&w=3840&q=75)
This is a Linear Programming Problem, which we shall solve by the graphical method.
1. Define the variables:
Let X represent the Small Truck, Y represent the Large Truck.
Let Z: define the total cost function.
2. The objective is to determine the number of loads per day, such that the value of Z is minimized.
Minimize Z: 72 X + 54 Y
3. Mention the constraints:
It is given that Bruce must deliver at least 300 cubic yards per day.
Next, the contract requires that the total number of loads per day is minimum 7.
Therefore, the LPP is subjected to constraints;
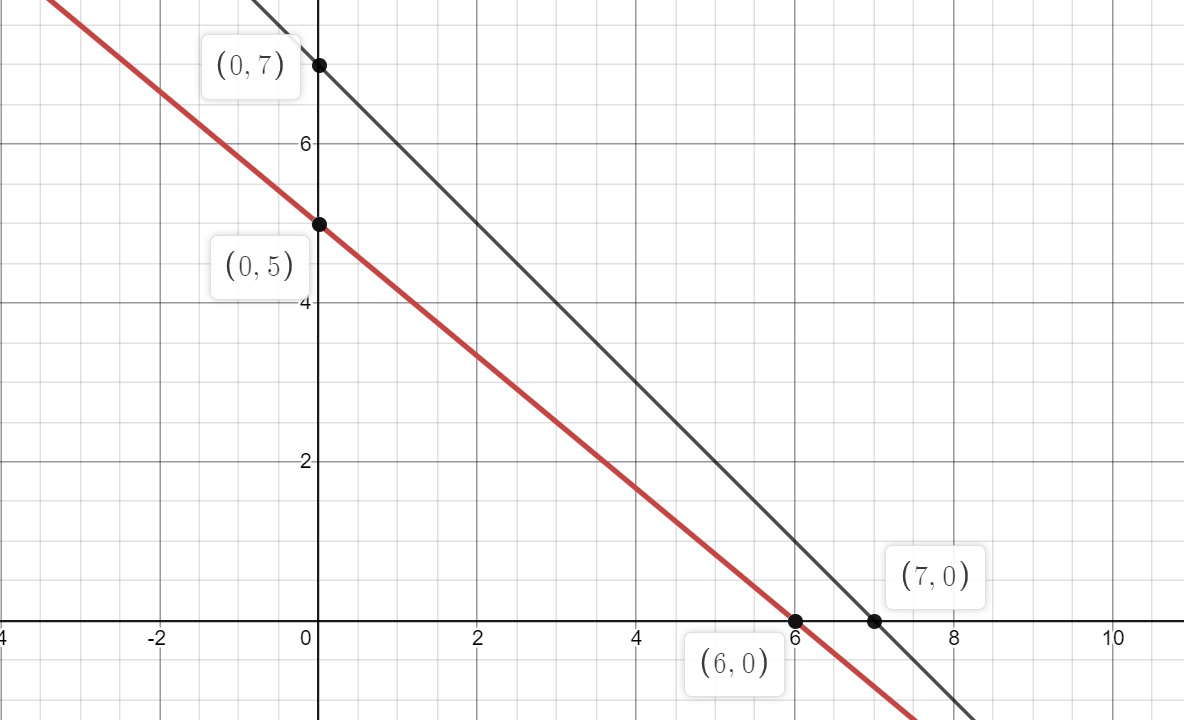
4. Now, plot the given constraints on X-Y plane. Note that the feasible region will lie in the first quadrant.
Trending now
This is a popular solution!
Step by step
Solved in 3 steps with 1 images









