Several years ago, the mean height of women 20 years of age or older was 63.7 inches. Suppose that a random sample of 45 women who are 20 years of age or older today results in a mean height of 64.1 inches (a) State the appropriate null and alternative hypotheses to assess whether women are taller today. (b) Suppose the P-value for this test is 0.11. Explain what this value represents. (c) Write a conclusion for this hypothesis test assuming an a= 0.05 level of significance. (a) State the appropriate null and alternative hypotheses to assess whether women are taller today. OA. H=64.1 in versus H, µ/64.1 in. OC. Ho H=63.7 in versus H, p<63.7 in. OE. Ho p=63.7 in versus H, p> 63.7 in. CITT OB. Ho u 63.7 in versus H, #63.7 in OD. Ho H=64.1 in versus H, p<64.1 in. OF. Hou 64.1 in versus H, µ> 64.1 in.
Several years ago, the mean height of women 20 years of age or older was 63.7 inches. Suppose that a random sample of 45 women who are 20 years of age or older today results in a mean height of 64.1 inches (a) State the appropriate null and alternative hypotheses to assess whether women are taller today. (b) Suppose the P-value for this test is 0.11. Explain what this value represents. (c) Write a conclusion for this hypothesis test assuming an a= 0.05 level of significance. (a) State the appropriate null and alternative hypotheses to assess whether women are taller today. OA. H=64.1 in versus H, µ/64.1 in. OC. Ho H=63.7 in versus H, p<63.7 in. OE. Ho p=63.7 in versus H, p> 63.7 in. CITT OB. Ho u 63.7 in versus H, #63.7 in OD. Ho H=64.1 in versus H, p<64.1 in. OF. Hou 64.1 in versus H, µ> 64.1 in.
MATLAB: An Introduction with Applications
6th Edition
ISBN:9781119256830
Author:Amos Gilat
Publisher:Amos Gilat
Chapter1: Starting With Matlab
Section: Chapter Questions
Problem 1P
Related questions
Question

Transcribed Image Text:### Hypothesis Testing on Women's Height
**Scenario:**
Several years ago, the mean height of women aged 20 years or older was 63.7 inches. A recent random sample of 45 women aged 20 or older shows a mean height of 64.1 inches.
### Questions and Explanation:
**(a) State the appropriate null and alternative hypotheses to assess whether women are taller today.**
Choose from the following options:
- **A.** \( H_0: \mu = 64.1 \text{ in.} \) versus \( H_1: \mu \neq 64.1 \text{ in.} \)
- **B.** \( H_0: \mu = 63.7 \text{ in.} \) versus \( H_1: \mu \neq 63.7 \text{ in.} \)
- **C.** \( H_0: \mu = 63.7 \text{ in.} \) versus \( H_1: \mu < 63.7 \text{ in.} \)
- **D.** \( H_0: \mu = 64.1 \text{ in.} \) versus \( H_1: \mu < 64.1 \text{ in.} \)
- **E.** \( H_0: \mu = 63.7 \text{ in.} \) versus \( H_1: \mu > 63.7 \text{ in.} \)
- **F.** \( H_0: \mu = 64.1 \text{ in.} \) versus \( H_1: \mu > 64.1 \text{ in.} \)
**(b) Suppose the P-value for this test is 0.11. Explain what this value represents.**
The P-value of 0.11 indicates that, assuming the null hypothesis \( H_0 \) is true, there is an 11% probability of obtaining a sample mean of 64.1 inches or more extreme by random chance.
**(c) Write a conclusion for this hypothesis test assuming an \( \alpha = 0.05 \) level of significance.**
Since the P-value of 0.11 is greater than the significance level \( \alpha \) of 0.05, we do not reject the
Expert Solution
Step 1: Providing given information
Population mean
sample size n= 45
sample mean = 64.1
Step by step
Solved in 5 steps with 6 images

Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
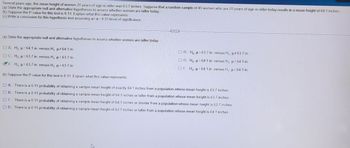
Transcribed Image Text:Several years ago, the mean height of women 20 years of age or older was 63.7 inches. Suppose that a random sample of 45 women who are 20 years of age or older today results in a mean height of 64.1 inches.
(a) State the appropriate null and alternative hypotheses to assess whether women are taller today.
(b) Suppose the P-value for this test is 0.11. Explain what this value represents.
(c) Write a conclusion for this hypothesis test assuming an α = 0.05 level of significance.
(a) State the appropriate null and alternative hypotheses to assess whether women are taller today.
- A. \( H_0: \mu = 64.1 \text{ in. versus } H_1: \mu \neq 64.1 \text{ in.} \)
- B. \( H_0: \mu = 63.7 \text{ in. versus } H_1: \mu \neq 63.7 \text{ in.} \)
- C. \( H_0: \mu = 63.7 \text{ in. versus } H_1: \mu < 63.7 \text{ in.} \)
- D. \( H_0: \mu = 64.1 \text{ in. versus } H_1: \mu < 64.1 \text{ in.} \)
- E. \( H_0: \mu = 63.7 \text{ in. versus } H_1: \mu > 63.7 \text{ in.} \) ✓
- F. \( H_0: \mu = 64.1 \text{ in. versus } H_1: \mu > 64.1 \text{ in.} \)
(b) Suppose the P-value for this test is 0.11. Explain what this value represents.
- A. There is a 0.11 probability of obtaining a sample mean height of exactly 64.1 inches from a population whose mean height is 63.7 inches.
- B. There is a 0.11 probability of obtaining a sample mean height of 64.1 inches or taller from a population whose mean height is 63.7 inches.
- C. There is a 0.11 probability of obtaining a sample mean height of 64.1 inches or
Solution
Recommended textbooks for you

MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc

Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning

Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning

MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc

Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning

Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning

Elementary Statistics: Picturing the World (7th E…
Statistics
ISBN:
9780134683416
Author:
Ron Larson, Betsy Farber
Publisher:
PEARSON

The Basic Practice of Statistics
Statistics
ISBN:
9781319042578
Author:
David S. Moore, William I. Notz, Michael A. Fligner
Publisher:
W. H. Freeman

Introduction to the Practice of Statistics
Statistics
ISBN:
9781319013387
Author:
David S. Moore, George P. McCabe, Bruce A. Craig
Publisher:
W. H. Freeman