As a warm-up, suppose we have a underdamped, unforced spring-mass system with mass 0.8 kg, spring constant 18 N/m, and damping coefficient 5 kg/s. We pull the mass 0.3 m from its rest position and let it go while imparting an initial velocity of 0.7 m/s. (a) Set up and solve the initial value problem for this spring-mass system. (b) Write your answer from part (a) in phase-amplitude form, i.e. as y(t) = Aet sin(ßt – ø) and graph the result. Compare with a graph of your answer from (a) to check that you have the correct amplitude and phase shift. (c) Find the values of t at which y(t) attains a local maximum (there will be infinitely many, of course), and observe that the time distance between any two consecutive maxima is always the same. (d) Compute the ratio of the displacements at two consecutive maxima. (That is, if y has a maximum at tn, and the next one occurs at tn+1, compute y(tn)/y(tn+1).) Observe that this ratio is constant.
As a warm-up, suppose we have a underdamped, unforced spring-mass system with mass 0.8 kg, spring constant 18 N/m, and damping coefficient 5 kg/s. We pull the mass 0.3 m from its rest position and let it go while imparting an initial velocity of 0.7 m/s. (a) Set up and solve the initial value problem for this spring-mass system. (b) Write your answer from part (a) in phase-amplitude form, i.e. as y(t) = Aet sin(ßt – ø) and graph the result. Compare with a graph of your answer from (a) to check that you have the correct amplitude and phase shift. (c) Find the values of t at which y(t) attains a local maximum (there will be infinitely many, of course), and observe that the time distance between any two consecutive maxima is always the same. (d) Compute the ratio of the displacements at two consecutive maxima. (That is, if y has a maximum at tn, and the next one occurs at tn+1, compute y(tn)/y(tn+1).) Observe that this ratio is constant.
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
TYPE ON 2-E
"Suppose we keep the mass and spring constant the same as in part (a), but we do not know the damping coefficient b."

Transcribed Image Text:Problem 2 (Estimating the Damping Constant). Recall that we can experimentally mea-
sure a spring constant using Hooke's law-we measure the force F required to stretch the
spring by a certain y from its natural length, and then we solve the equation F = ky for
the spring constant k. Presumably we would have to determine the damping coefficient of
a dashpot empirically as well, but how would we do so?
As a warm-up, suppose we have a underdamped, unforced spring-mass system with mass
0.8 kg, spring constant 18 N/m, and damping coefficient 5 kg/s. We pull the mass 0.3 m
from its rest position and let it go while imparting an initial velocity of 0.7 m/s.
%3D
(a) Set up and solve the initial value problem for this spring-mass system.
(b) Write your answer from part (a) in phase-amplitude form, i.e. as
y(t) = Aeºt sin(ßt – 4)
and graph the result. Compare with a graph of your answer from (a) to check that
you have the correct amplitude and phase shift.
(c) Find the values of t at which y(t) attains a local maximum (there will be infinitely
many, of course), and observe that the time distance between any two consecutive
maxima is always the same.
(d) Compute the ratio of the displacements at two consecutive maxima. (That is, if y has
a maximum at tn, and the next one occurs at tn+1, compute y(tn)/y(tn+1).) Observe
that this ratio is constant.
(e) Everything we have done so far generalizes to all underdamped spring-mass systems,
and we can use it to estimate the damping coefficient experimentally. Suppose we
keep the mass and spring constant the same as in part (a), but we do not know the
spring constant b. Redo parts (a)-(d) (except for graphing y(t)) and express your
answer in terms of b.
(f) Suppose that after we release the mass, the first maximum displacement is 0.5 m,
and then 4 seconds later another maximum displacement of 0.2 m occurs. Use your
answer from part (e) to estimate the damping coefficient.
Expert Solution
Step 1
Since you have posted a question with multiple sub-parts, we will solve the first three subparts for you. To get the remaining sub-parts solved please repost the complete question and mention the sub-parts.

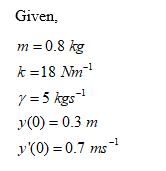
Step by step
Solved in 5 steps with 13 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, advanced-math and related others by exploring similar questions and additional content below.Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

