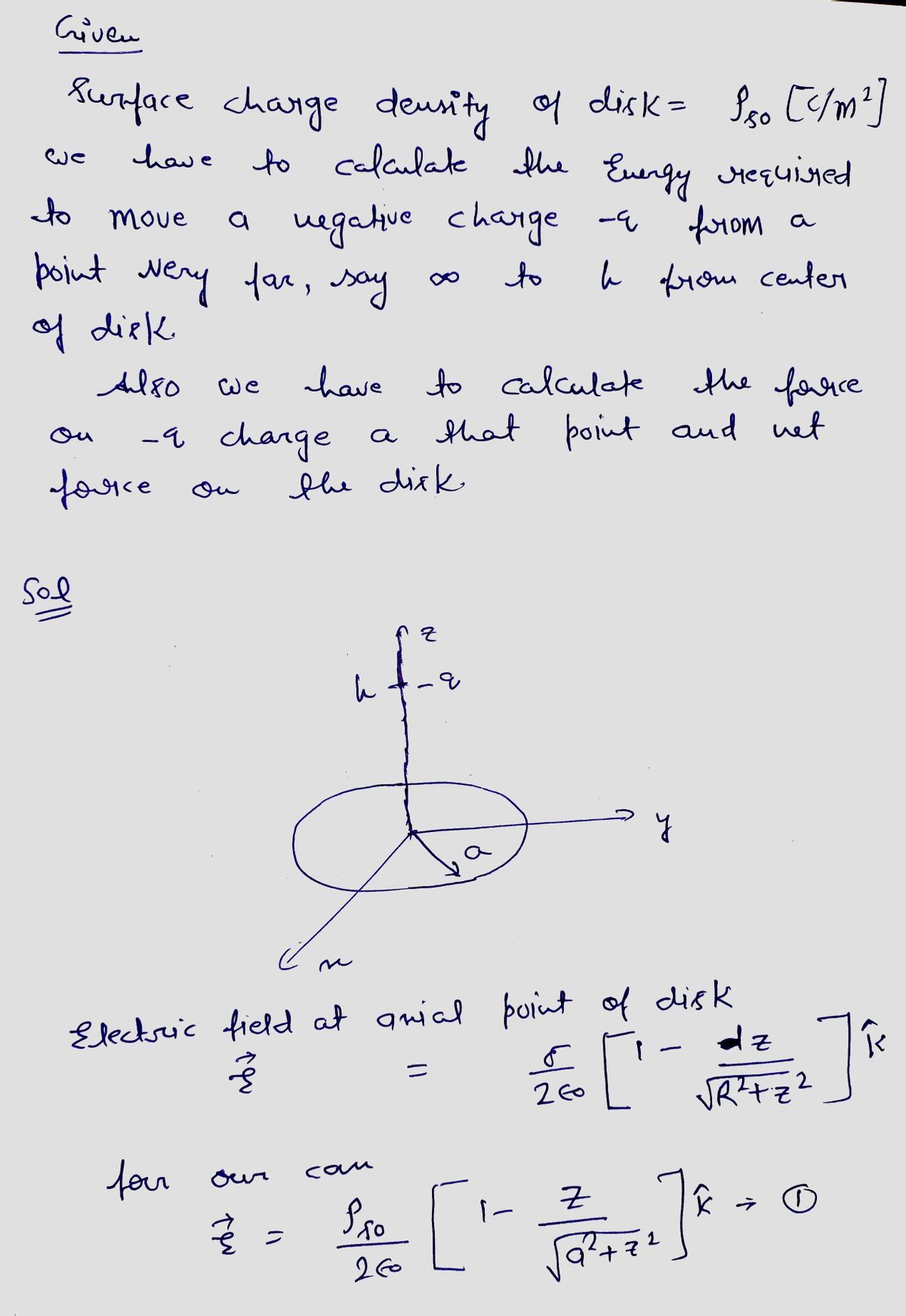
A thin disk of radius a carries a uniform surface charge density po [C/m²]. Find the energy required to move a negative point charge -q from a point very far from the disk (infinity) to a final position along its axis, a distance h from the center of the disk as shown. This can be done two ways using knowledge of the field and potential of a uniformly-charged disk, which is derived in the lecture slides. What is the force on the charge in its final resting position? What is the net force on the disk? X AZ h-e-q a Pso y
A thin disk of radius a carries a uniform surface charge density po [C/m²]. Find the energy required to move a negative point charge -q from a point very far from the disk (infinity) to a final position along its axis, a distance h from the center of the disk as shown. This can be done two ways using knowledge of the field and potential of a uniformly-charged disk, which is derived in the lecture slides. What is the force on the charge in its final resting position? What is the net force on the disk? X AZ h-e-q a Pso y
Related questions
Question
Hand write,more details,as soon as possible.
![A thin disk of radius a carries a uniform surface charge
density po [C/m²]. Find the energy required to move a
negative point charge -q from a point very far from the
disk (infinity) to a final position along its axis, a
distance h from the center of the disk as shown. This
can be done two ways using knowledge of the field and
potential of a uniformly-charged disk, which is derived
in the lecture slides. What is the force on the charge in
its final resting position? What is the net force on
the disk?
X
Z
h--q
a
Pso
y](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F099a9854-7cc3-46b9-a37c-ec0486b4a12a%2F62d8b8fa-9af2-4f49-a3b6-e701c02c339e%2Ffp2qzik_processed.png&w=3840&q=75)
Transcribed Image Text:A thin disk of radius a carries a uniform surface charge
density po [C/m²]. Find the energy required to move a
negative point charge -q from a point very far from the
disk (infinity) to a final position along its axis, a
distance h from the center of the disk as shown. This
can be done two ways using knowledge of the field and
potential of a uniformly-charged disk, which is derived
in the lecture slides. What is the force on the charge in
its final resting position? What is the net force on
the disk?
X
Z
h--q
a
Pso
y
Expert Solution
Step 1
Step by step
Solved in 3 steps with 3 images
