**Bacterial Growth Study**A strain of bacteria is placed into a nutrient broth at 30°C and allowed to grow. The data collected is presented in the table below. The population is measured in grams and the time in hours. Since the population \( P \) depends on time \( t \), and each input corresponds to exactly one output, we say that population is a function of time; so \( P(t) \) represents the population at time \( t \).**Table: Bacterial Growth Over Time**| Time (hr), \( t \) | Population (g), \( P \) ||-------------------|-------------------------|| 0 | 0.02 || 2.5 | 0.18 || 3.5 | 0.23 || 4.5 | 0.35 || 6 | 0.51 |---**Problem:**(a) Find the average rate of change of the population from 0 to 2.5 hours.To calculate the average rate of increase, use the formula:\[\text{Average Rate of Change} = \frac{P(t_2) - P(t_1)}{t_2 - t_1}\]where \( t_1 = 0 \), \( P(t_1) = 0.02 \), \( t_2 = 2.5 \), and \( P(t_2) = 0.18 \).On average, the population is increasing at a rate of \( \_\_\_ \) grams per hour from 0 to 2.5 hours. (Round to 3 decimal places as needed.)

A strain of bacteria is placed into a nutrient broth at 30° Time (hr), t Population (g), P C and allowed to grow. The data shown to the right are collected. The population is measured in grams and the time in hours. Since population P depends on time t and each input corresponds to exactly one output, we say that population is a function of time; so P(t) represents the population at time t. 0 NM 50 50 2.5 3.5 4.5 6 (a) Find the average rate of change of the population from 0 to 2.5 hours. On average, the population is increasing at a rate of (Round to 3 decimal places as needed.) 0.02 0.18 0.23 0.35 0.51 gram per hour from 0 to 2.5 hours.

A strain of bacteria is placed into a nutrient broth at 30° Time (hr), t Population (g), P C and allowed to grow. The data shown to the right are collected. The population is measured in grams and the time in hours. Since population P depends on time t and each input corresponds to exactly one output, we say that population is a function of time; so P(t) represents the population at time t. 0 NM 50 50 2.5 3.5 4.5 6 (a) Find the average rate of change of the population from 0 to 2.5 hours. On average, the population is increasing at a rate of (Round to 3 decimal places as needed.) 0.02 0.18 0.23 0.35 0.51 gram per hour from 0 to 2.5 hours.

Advanced Engineering Mathematics

10th Edition

ISBN:9780470458365

Author:Erwin Kreyszig

Publisher:Erwin Kreyszig

Chapter2: Second-order Linear Odes

Section: Chapter Questions

Problem 1RQ

See similar textbooks

Related questions

Q: Question 10 Evaluate x 2 for the function y = 3/x +2-1 O 5 O 47 O 11 0 7

Q: A particle that moves along a straight line has a velocity V(t) = t e meters per second after t…

Q: A respiratory cycle is defined as the beginning of one breath to the beginning of the next breath.…

Q: Question 8 parts C and D

Q: Suppose a spring has spring constant k = 8. The spring has a mass of 32 units hanging from its end.…

Q: need correct answers

Q: Find energy in kJ of a photon. 2=488 nm. The speed of light is 3.00 x 108 m/s and h = 6.63 x 10-34 J…

A: Energy

Q: The data collected in a research study well either support or refute the -that the study is designed…

Q: uppose that at 12:01 a.m. on January 1 (just after midnight), there is one cell. And suppose it…

Q: QUESTION 4 A horse runs with velocity 15 km/h for 0.05 hours (hrs), 20 km/h for the next 0.1 hours,…

Q: If y is inversely proportional to x, and y= 1/9 when x= 12, find the value of y when x= 8.…

A: given y is inversely proportional to x given y=19 when x=12

Q: In example 4, we investigated Holling’s disk equation. P=(aN)/(1+aTN) We will now consider P as a…

Q: A strain of bacteria is placed into a nutrient broth at 30° Time (hr), t Population (g), P C and…

Q: Define the following: Heaviside step function Unit Step Function The Dirac Delta Function…

Q: The population P of a city is given by P = 80, 000e0.015€ where t represents the year, with t = 0…

A: Given information: Given that t = 0 corresponding to 2000. The population is P = 100,000. The given…

Q: Select all the lines that are needed to find the inverse of y = log, (x + 1) – 2 in the appropriate…

A: Given,y=log3(x+1)-2

Q: (a). Most often, we move items from one environment to another. A Ph.D researcher found that in…

A: Since you have asked multiple question, we will solve the mentioned first question for you. If you…

Question

100%

**Bacterial Growth Study**

A strain of bacteria is placed into a nutrient broth at 30°C and allowed to grow. The data collected is presented in the table below. The population is measured in grams and the time in hours. Since the population \( P \) depends on time \( t \), and each input corresponds to exactly one output, we say that population is a function of time; so \( P(t) \) represents the population at time \( t \).

**Table: Bacterial Growth Over Time**

| Time (hr), \( t \) | Population (g), \( P \) |
|-------------------|-------------------------|
| 0 | 0.02 |
| 2.5 | 0.18 |
| 3.5 | 0.23 |
| 4.5 | 0.35 |
| 6 | 0.51 |

---

**Problem:**

(a) Find the average rate of change of the population from 0 to 2.5 hours.

To calculate the average rate of increase, use the formula:

\[
\text{Average Rate of Change} = \frac{P(t_2) - P(t_1)}{t_2 - t_1}
\]

where \( t_1 = 0 \), \( P(t_1) = 0.02 \), \( t_2 = 2.5 \), and \( P(t_2) = 0.18 \).

On average, the population is increasing at a rate of \( \_\_\_ \) grams per hour from 0 to 2.5 hours. (Round to 3 decimal places as needed.)

Expert Solution

This question has been solved!

Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.

This is a popular solution!

SEE SOLUTION Check out a sample Q&A here

Step 1: Explanation

VIEW

Step 2: Answer

VIEW

Solution

VIEW

Trending now

This is a popular solution!

Step by step

Solved in 3 steps with 1 images

SEE SOLUTION Check out a sample Q&A here

Follow-up Questions

Read through expert solutions to related follow-up questions below.

Follow-up Question

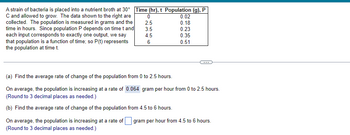
A strain of bacteria is placed into a nutrient broth at 30°C and allowed to grow. The data shown to the right are collected. The population is measured in grams and the time in hours. Since population \( P \) depends on time \( t \) and each input corresponds to exactly one output, we say that population is a function of time; so \( P(t) \) represents the population at time \( t \).

**Table**

- **Time (hr)**, \( t \)
- **Population (g)**, \( P \)

| Time (hr), \( t \) | Population (g), \( P \) |
|------------------|-----------------------|
| 0 | 0.02 |
| 2.5 | 0.18 |
| 3.5 | 0.23 |
| 4.5 | 0.35 |
| 6 | 0.51 |

**Questions:**

(a) Find the average rate of change of the population from 0 to 2.5 hours.

- On average, the population is increasing at a rate of \( 0.064 \) gram per hour from 0 to 2.5 hours. (Round to 3 decimal places as needed.)

(b) Find the average rate of change of the population from 4.5 to 6 hours.

- On average, the population is increasing at a rate of \(\_\_\) gram per hour from 4.5 to 6 hours. (Round to 3 decimal places as needed.)

Solution

by Bartleby Expert

SEE SOLUTION

Similar questions

OM Question 3 A is between Y and Z and YA = 12x, AZ= 42, and YZ = 78. Find YA. %3D YA= Question 4
Coober Pedy is a town in Australia that mines most of the world's opals (precious gemstones). Because of the scorching heat, much of the population lives in underground "dugouts." The Coober Pedy monthly high temperatures are shown in the figure below, with H (n) the temperature in month n, where n = 1 is January. We fit the data with a function, H (n) = A cos(Bn) + C. temperature (°C) 36.7 27.5 18.4 135 C = (a) What does the value of C represent and what is its value? Estimate your answer to one decimal place. i 7 9 11 It represents the n (months) O maximum temperature. O month at which the temperature is equal to the average temperature. O minimum temperature. O average temperature. temperature variation above and below the average.
On wet roads, under certain conditions the front tires of a car will hydroplane, or run along the surface of the water. The critical speed V at which hydroplaning occurs is a function of p, the tire inflation pressure. Suppose the following table shows hypothetical data for p, in pounds per square inch, and V, in miles per hour. Find a formula that models V as a power function of p. Round parameters to the nearest hundredth. Tire inflation pressure p Critical speed for hydroplaning V 25 52.5 30 57.4 35 62.0 40 66.2 a) V = 35.89 x p^0.02 b) V = -41.49 x p^67.11 c) V = 29.82 x p^0.91 d) V = 10.70 x p^0.49
Speedometer readings for a vehicle (in motion) at 5-second intervals are given in the table. t (sec) v (ft/s) 10 15 20 25 30 6. 21 37 47 46 34 Estimate the distance traveled by the vehicle during this 30-second period using Ln. Ra and M.. Lo feet %3D Ro = feet feet %3D
Use the method of undetermined coefficients to determine the form of a particular solution for the given equation. y'"' + 10y" – 11y=xe* + 4x What is the form of the particular solution with undetermined coefficients? Yp(x) = ] (Do not use d, D, e, E, i, or I as arbitrary constants since these letters already have defined meanings.)