A slab of weight 40 N rests on a horizontal floor. A block of weight WB = 80 N rests on top of the slab. The coefficient of friction between the block and the slab is μs = 1/3. The coefficient of friction between the slab and the table is μk = 1/4. A horizontal external force F is applied on the slab. What is the maximum value of F so that there is still no relative motion between the block and slab?
A slab of weight 40 N rests on a horizontal floor. A block of weight WB = 80 N rests on top of the slab. The coefficient of friction between the block and the slab is μs = 1/3. The coefficient of friction between the slab and the table is μk = 1/4. A horizontal external force F is applied on the slab. What is the maximum value of F so that there is still no relative motion between the block and slab? (Show work)
Given:
Weight of slab (Ws) = 40 N.
Weight of block (Wb) = 80 N.
Coefficient of friction between block and slab (µs) = 0.33
Coefficient of friction between slab and table (µk) = 0.25
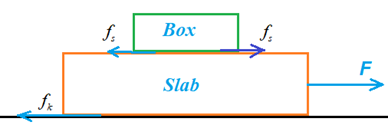
The free body diagram of block and slab is given as,

The equilibrium equation of box,
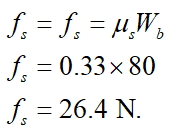
Acceleration of block due to applied force,
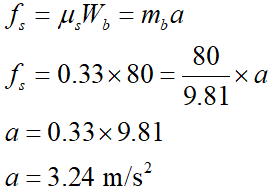
Step by step
Solved in 5 steps with 6 images









