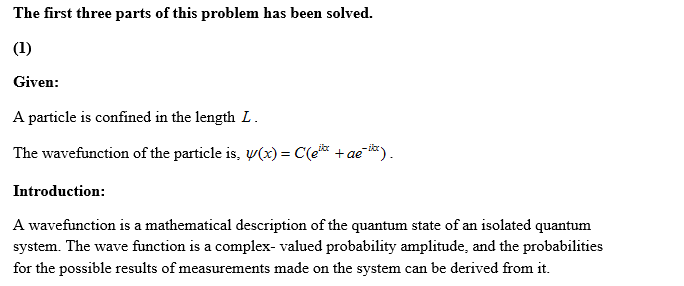
A particle confined in a region of length L has a wave function being a superposition of two counter-propagating de-Broglie waves: (x) = C(ekz + ue¬ikz). Here a = a1+ia2 = ao exp(ip) is a complex constant, where ao = Vaf + a and tan y = a2/a1. Calculate: 1. The normalization constant C. 2. The probability density to find a particle at the position r. 3. The probability current density J(r) as a function of k, ao and p.
A particle confined in a region of length L has a wave function being a superposition of two counter-propagating de-Broglie waves: (x) = C(ekz + ue¬ikz). Here a = a1+ia2 = ao exp(ip) is a complex constant, where ao = Vaf + a and tan y = a2/a1. Calculate: 1. The normalization constant C. 2. The probability density to find a particle at the position r. 3. The probability current density J(r) as a function of k, ao and p.
Related questions
Question

Transcribed Image Text:A particle confined in a region of length L has a wave function
being a superposition of two counter-propagating de-Broglie waves:
(x) = C(ekz + ue¬ikz).
Here a = a1+ia2 = ao exp(ip) is a complex constant, where ao = Vaf + a
and tan y = a2/a1.
Calculate:
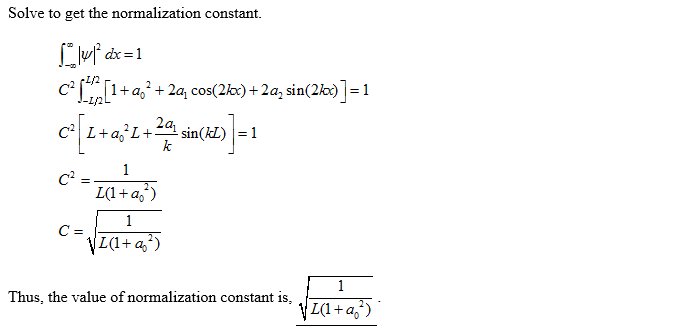
1. The normalization constant C.
2. The probability density to find a particle at the position r.
3. The probability current density J(r) as a function of k, ao and p.
Expert Solution
Step 1
Step 2

Step by step
Solved in 4 steps with 5 images
