A parkour enthusiast attempts a dangerous jump from building to building, which involves a drop of 4.05 m from rooftop to rooftop across a gap of 5.20 m. He can run at a maximum speed of 5.80 m/s. 1. (a) Show that he can safely make the jump. (Assume that his initial velocity is horizontal.) (b) How fast is he moving just before he lands?
A parkour enthusiast attempts a dangerous jump from building to building, which involves a drop of 4.05 m from rooftop to rooftop across a gap of 5.20 m. He can run at a maximum speed of 5.80 m/s. 1. (a) Show that he can safely make the jump. (Assume that his initial velocity is horizontal.) (b) How fast is he moving just before he lands?
Related questions
Question
1. A parkour enthusiast attempts a dangerous jump from building to building, which involves a drop of 4.05 m from rooftop to rooftop across a gap of 5.20 m. He can run at a maximum speed of 5.80 m/s.
a) Show that he can safely make the jump. (Assume that his initial velocity is horizontal. b) How fast is he moving just before he lands?
Transcribed Image Text:A parkour enthusiast attempts a dangerous jump from building to building, which involves a drop
of 4.05 m from rooftop to rooftop across a gap of 5.20 m. He can run at a maximum speed of 5.80 m/s.
1.
(a)
Show that he can safely make the jump. (Assume that his initial velocity is horizontal.)
(b)
How fast is he moving just before he lands?
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
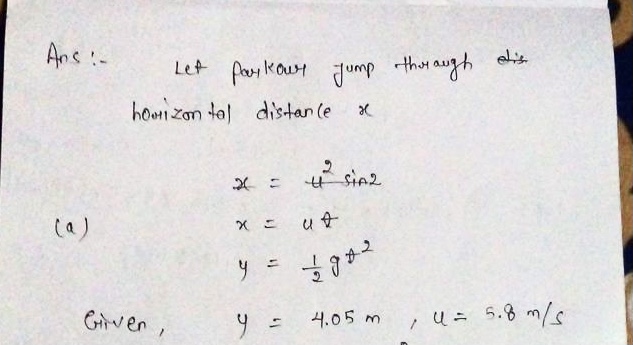
Step by step
Solved in 2 steps with 2 images
