Homework Help is Here – Start Your Trial Now!
Engineering
Electrical Engineering
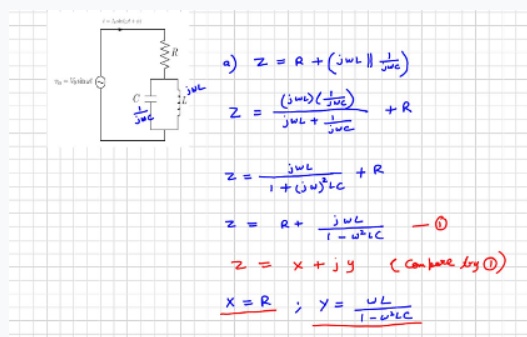
2. Consider the LRC circuit below. Vin Vo sin wt i lo sin(wt + o) C www R L (a) Find an analytic expression for the equivalent impedance of the R, L, and C combination. Remember that Zc = 1/(jwC) and ZL = jwL and that you can combine impedances in series and parallel the same way that you would combine resistances. Give your answer in the form Z = X+jY (i.e. find X and Y). (b) The current in the resistor is given by i = Io sin(wt + o). Find Io and tano in terms of Vo, w, R, L, and C. (c) Plot VR/Vo as a function of frequency. VR represents the amplitude of the voltage across the resistor. Use component values L = 300 μH, C = 15 μF, and R = 1000 2 and plot over a frequency range of f= 2200-2500 Hz. Don't forget that w 2πf. Don't draw your graph by hand, use plotting software. (d) What is the impedance of the parallel LC part of the circuit when w=1/√LC? Compare this to the impedance of a series combination of L and C when w=1/√LC.

2. Consider the LRC circuit below. Vin Vo sin wt i lo sin(wt + o) C www R L (a) Find an analytic expression for the equivalent impedance of the R, L, and C combination. Remember that Zc = 1/(jwC) and ZL = jwL and that you can combine impedances in series and parallel the same way that you would combine resistances. Give your answer in the form Z = X+jY (i.e. find X and Y). (b) The current in the resistor is given by i = Io sin(wt + o). Find Io and tano in terms of Vo, w, R, L, and C. (c) Plot VR/Vo as a function of frequency. VR represents the amplitude of the voltage across the resistor. Use component values L = 300 μH, C = 15 μF, and R = 1000 2 and plot over a frequency range of f= 2200-2500 Hz. Don't forget that w 2πf. Don't draw your graph by hand, use plotting software. (d) What is the impedance of the parallel LC part of the circuit when w=1/√LC? Compare this to the impedance of a series combination of L and C when w=1/√LC.

Introductory Circuit Analysis (13th Edition)
Introductory Circuit Analysis (13th Edition)
13th Edition
ISBN:9780133923605
Author:Robert L. Boylestad
Publisher:Robert L. Boylestad
Chapter1: Introduction
Section: Chapter Questions
Problem 1P: Visit your local library (at school or home) and describe the extent to which it provides literature...
See similar textbooks
icon
Related questions
Question
In class we showed that any complex number Z can be written in either of the following two forms: Z = X+jY Z = |Z| ej To go between these two forms, you can use the following relationships: |Z| = √X² +Y² Y X X = |Z| cos o Y = |Z| sino We also introduced one more quantity called the complex conjugate of Z, which is denoted Z*. To find the complex conjugate of Z, you simply reverse the sign in front of all the j's. So, for example, if: Z = X+jY= |Z| e¹ then: tan o = Z* = X-jY= |Z| e-j The complex conjugate is useful because it can be used to calculate |Z|, the magnitude of Z. Consider, for example, the following product: ZZ* = (x+jY) (X -jY) = x² + y² = |Z|². Alternatively, using the exponential form of Z: ZZ = (|Z|e¹) (|Z| e-jº) = |Z|². In both cases we get that ZZ* = |Z|².
Transcribed Image Text:In class we showed that any complex number Z can be written in either of the following two forms: Z = X+jY Z = |Z| ej To go between these two forms, you can use the following relationships: |Z| = √X² +Y² Y X X = |Z| cos o Y = |Z| sino We also introduced one more quantity called the complex conjugate of Z, which is denoted Z*. To find the complex conjugate of Z, you simply reverse the sign in front of all the j's. So, for example, if: Z = X+jY= |Z| e¹ then: tan o = Z* = X-jY= |Z| e-j The complex conjugate is useful because it can be used to calculate |Z|, the magnitude of Z. Consider, for example, the following product: ZZ* = (x+jY) (X -jY) = x² + y² = |Z|². Alternatively, using the exponential form of Z: ZZ = (|Z|e¹) (|Z| e-jº) = |Z|². In both cases we get that ZZ* = |Z|².
2. Consider the LRC circuit below. Vin = Vo sin wt i = lo sin(wt + o) C R L (a) Find an analytic expression for the equivalent impedance of the R, L, and C combination. Remember that Zc = 1/(jwC) and Zz = jwL and that you can combine impedances in series and parallel the same way that you would combine resistances. Give your answer in the form Z = X+jY (i.e. find X and Y). (b) The current in the resistor is given by i = Io sin(wt + o). Find Io and tan o in terms of Vo, w, R, L, and C. (c) Plot VR/Vo as a function of frequency. VR represents the amplitude of the voltage across the resistor. Use component values L = 300 µH, C = 15 µF, and R = 1000 2 and plot over a frequency range of f = 2200-2500 Hz. Don't forget that w 2πf. Don't draw your graph by hand, use plotting software. = (d) What is the impedance of the parallel LC part of the circuit when w=1/√LC? Compare this to the impedance of a series combination of L and C when w=1/√LC.
Transcribed Image Text:2. Consider the LRC circuit below. Vin = Vo sin wt i = lo sin(wt + o) C R L (a) Find an analytic expression for the equivalent impedance of the R, L, and C combination. Remember that Zc = 1/(jwC) and Zz = jwL and that you can combine impedances in series and parallel the same way that you would combine resistances. Give your answer in the form Z = X+jY (i.e. find X and Y). (b) The current in the resistor is given by i = Io sin(wt + o). Find Io and tan o in terms of Vo, w, R, L, and C. (c) Plot VR/Vo as a function of frequency. VR represents the amplitude of the voltage across the resistor. Use component values L = 300 µH, C = 15 µF, and R = 1000 2 and plot over a frequency range of f = 2200-2500 Hz. Don't forget that w 2πf. Don't draw your graph by hand, use plotting software. = (d) What is the impedance of the parallel LC part of the circuit when w=1/√LC? Compare this to the impedance of a series combination of L and C when w=1/√LC.
Expert Solution
Step 1

Electrical Engineering homework question answer, step 1, image 1

steps

Step by step

Solved in 3 steps with 3 images

SEE SOLUTIONCheck out a sample Q&A here
Blurred answer
Knowledge Booster
Types of Resonance and Different Parameter
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, electrical-engineering and related others by exploring similar questions and additional content below.
Similar questions
Recommended textbooks for you
Introductory Circuit Analysis (13th Edition)
Introductory Circuit Analysis (13th Edition)
Electrical Engineering
ISBN:
9780133923605
Author:
Robert L. Boylestad
Publisher:
PEARSON
Delmar's Standard Textbook Of Electricity
Delmar's Standard Textbook Of Electricity
Electrical Engineering
ISBN:
9781337900348
Author:
Stephen L. Herman
Publisher:
Cengage Learning
Programmable Logic Controllers
Programmable Logic Controllers
Electrical Engineering
ISBN:
9780073373843
Author:
Frank D. Petruzella
Publisher:
McGraw-Hill Education
Fundamentals of Electric Circuits
Fundamentals of Electric Circuits
Electrical Engineering
ISBN:
9780078028229
Author:
Charles K Alexander, Matthew Sadiku
Publisher:
McGraw-Hill Education
Electric Circuits. (11th Edition)
Electric Circuits. (11th Edition)
Electrical Engineering
ISBN:
9780134746968
Author:
James W. Nilsson, Susan Riedel
Publisher:
PEARSON
Engineering Electromagnetics
Engineering Electromagnetics
Electrical Engineering
ISBN:
9780078028151
Author:
Hayt, William H. (william Hart), Jr, BUCK, John A.
Publisher:
Mcgraw-hill Education,
SEE MORE TEXTBOOKS