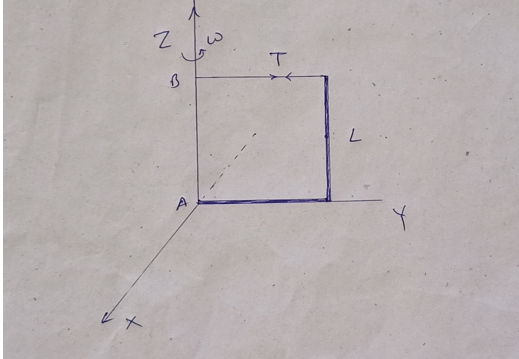
2. An L-bracket is composed of two identical thin sticks each with mass M and length L. The bracket rotates about the vertical at constant angular speed co and is supported by a bearing at the bottom, point A, as well as by a massless cord at the top of the bracket. The cord is parallel to the xy-plane and rotates with the bracket. Determine the tension in the cord.
2. An L-bracket is composed of two identical thin sticks each with mass M and length L. The bracket rotates about the vertical at constant angular speed co and is supported by a bearing at the bottom, point A, as well as by a massless cord at the top of the bracket. The cord is parallel to the xy-plane and rotates with the bracket. Determine the tension in the cord.
College Physics
11th Edition
ISBN:9781305952300
Author:Raymond A. Serway, Chris Vuille
Publisher:Raymond A. Serway, Chris Vuille
Chapter1: Units, Trigonometry. And Vectors
Section: Chapter Questions
Problem 1CQ: Estimate the order of magnitude of the length, in meters, of each of the following; (a) a mouse, (b)...
Related questions
Concept explainers
Angular speed, acceleration and displacement
Angular acceleration is defined as the rate of change in angular velocity with respect to time. It has both magnitude and direction. So, it is a vector quantity.
Angular Position
Before diving into angular position, one should understand the basics of position and its importance along with usage in day-to-day life. When one talks of position, it’s always relative with respect to some other object. For example, position of earth with respect to sun, position of school with respect to house, etc. Angular position is the rotational analogue of linear position.
Question
Transcribed Image Text:**Problem 2: Analysis of an L-Bracket System**
An L-bracket is composed of two identical thin sticks, each with mass \( M \) and length \( L \). The bracket rotates about the vertical axis at a constant angular speed \( \omega \) and is supported by a bearing at the bottom, referred to as point \( A \), as well as by a massless cord at the top of the bracket. The cord remains parallel to the xy-plane and rotates with the bracket. The task is to determine the tension in the cord.
---
**Visual Explanation:**
No specific graphs or diagrams are provided in the text. However, imagine the L-bracket as a system of two sticks forming a right angle. The setup revolves around a vertical axis with a support at the base (point \( A \)) and a supporting cord at the top, keeping the structure parallel to the xy-plane during rotation.
**Key Concepts:**
- **Rotation Dynamics**: Understanding how angular speed \( \omega \) influences the forces on the bracket.
- **Support Forces**: Identifying the role of the bearing and the cord in maintaining structural integrity.
- **Tension Calculation**: Applying principles from physics to find the cord's tension.
**Instructions for Solving the Problem:**
1. **Analyze Forces**: Consider centripetal force due to rotation and how it affects tension in the cord.
2. **Apply Equations of Motion**: Use the principles of rotational dynamics and equilibrium to set up equations.
3. **Simplify and Solve**: Isolate the variable representing tension and calculate its value using known quantities like mass \( M \), length \( L \), and angular speed \( \omega \).
This problem is an excellent exercise for students studying rotational dynamics and forces in physics.
Transcribed Image Text:The diagram illustrates a 3D coordinate system with axes labeled X, Y, and Z. At point A, located at the intersection of the X and Y axes, a structure begins with a vertical line extending upwards parallel to the Z-axis. This vertical line is labeled B at the top, where it connects to a horizontal line or "cord." The horizontal line extends perpendicular to the vertical line towards the Y-axis.
The Z-axis is marked with an arrow indicating a counterclockwise rotational motion, labeled with the angular velocity \(\omega\).
Key elements in the diagram:
- **Axes**: X, Y (horizontal plane), and Z (vertical axis).
- **Vertical Line**: Originating at point A, labeled B at the top end.
- **Cord**: A horizontal line at the top, parallel to the Y-axis.
- **Rotational Arrow**: Indicates the direction of rotation around the Z-axis.
This diagram can represent a mechanical system or a physics problem dealing with rotational motion.
Expert Solution
Step 1
Let ω denote the angular speed, T denote the string's tension, M denote the mass of each rod, L denote the length of each rod.
Step by step
Solved in 2 steps with 2 images
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Recommended textbooks for you
College Physics
Physics
ISBN:
9781305952300
Author:
Raymond A. Serway, Chris Vuille
Publisher:
Cengage Learning
University Physics (14th Edition)
Physics
ISBN:
9780133969290
Author:
Hugh D. Young, Roger A. Freedman
Publisher:
PEARSON
Introduction To Quantum Mechanics
Physics
ISBN:
9781107189638
Author:
Griffiths, David J., Schroeter, Darrell F.
Publisher:
Cambridge University Press
College Physics
Physics
ISBN:
9781305952300
Author:
Raymond A. Serway, Chris Vuille
Publisher:
Cengage Learning
University Physics (14th Edition)
Physics
ISBN:
9780133969290
Author:
Hugh D. Young, Roger A. Freedman
Publisher:
PEARSON
Introduction To Quantum Mechanics
Physics
ISBN:
9781107189638
Author:
Griffiths, David J., Schroeter, Darrell F.
Publisher:
Cambridge University Press
Physics for Scientists and Engineers
Physics
ISBN:
9781337553278
Author:
Raymond A. Serway, John W. Jewett
Publisher:
Cengage Learning
Lecture- Tutorials for Introductory Astronomy
Physics
ISBN:
9780321820464
Author:
Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina Brissenden
Publisher:
Addison-Wesley
College Physics: A Strategic Approach (4th Editio…
Physics
ISBN:
9780134609034
Author:
Randall D. Knight (Professor Emeritus), Brian Jones, Stuart Field
Publisher:
PEARSON