Homework Help is Here – Start Your Trial Now!
Math
Advanced Math
11. If A¹ = [1 27 4 6 12. If A¹ = -1 solve AX = 1 2 0] 3 1 2 4 1 [3] for X. -2 solve AX = 1 for X. 4

11. If A¹ = [1 27 4 6 12. If A¹ = -1 solve AX = 1 2 0] 3 1 2 4 1 [3] for X. -2 solve AX = 1 for X. 4

Algebra & Trigonometry with Analytic Geometry
Algebra & Trigonometry with Analytic Geometry
13th Edition
ISBN:9781133382119
Author:Swokowski
Publisher:Swokowski
Chapter2: Equations And Inequalities
Section2.3: Quadratic Equations
Problem 40E
See similar textbooks
Question

number 11, 12, 14, and 15. 

11. If A¹ = [1 2] 4 6 . solve AX = 3 [³] 1 for X. 1 2 0 -2 12. If A¹ = -1 3 1 solve AX = 1 for X. 2 4 4 4
Transcribed Image Text:11. If A¹ = [1 2] 4 6 . solve AX = 3 [³] 1 for X. 1 2 0 -2 12. If A¹ = -1 3 1 solve AX = 1 for X. 2 4 4 4
In Problems 15-19, use an inverse matrix to find the solution to the systems. x+2y+z=5 (3,4,0) 2x+y-3z=-2 (3x+y+4z=-5 x+3y-6z=7 15. 2x-y+2z=0 16. x+y+2z=-1 (1,0, -1) (x+2y. 17. 2x+3 -x-2
Transcribed Image Text:In Problems 15-19, use an inverse matrix to find the solution to the systems. x+2y+z=5 (3,4,0) 2x+y-3z=-2 (3x+y+4z=-5 x+3y-6z=7 15. 2x-y+2z=0 16. x+y+2z=-1 (1,0, -1) (x+2y. 17. 2x+3 -x-2
Expert Solution
steps

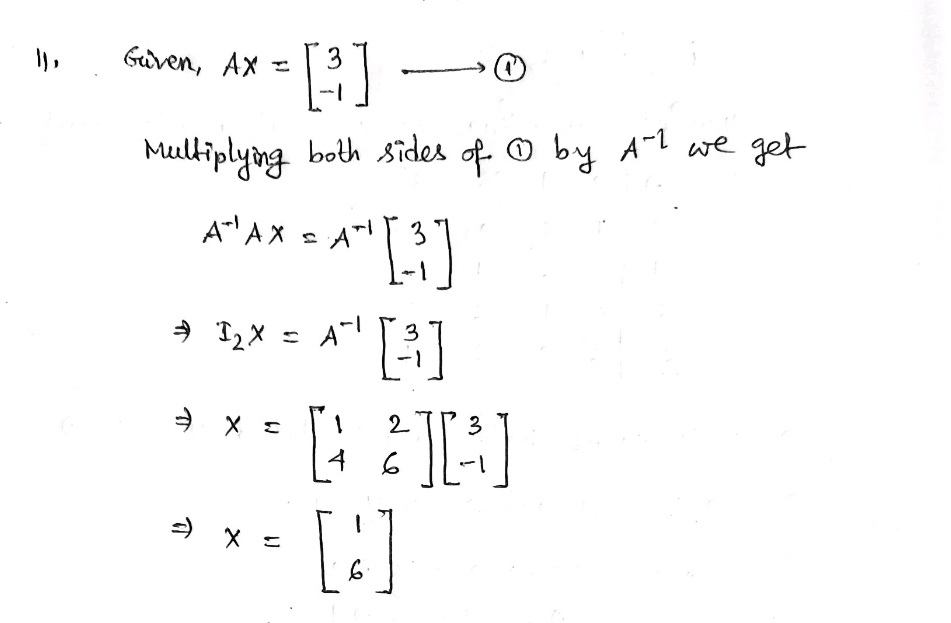
Step by step

Solved in 3 steps with 3 images

SEE SOLUTIONCheck out a sample Q&A here
Blurred answer
Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question

I meant 16 not 14! could you help 

Solution
Bartleby Expert
SEE SOLUTION
Recommended textbooks for you
Algebra & Trigonometry with Analytic Geometry
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage
Algebra: Structure And Method, Book 1
Algebra: Structure And Method, Book 1
Algebra
ISBN:
9780395977224
Author:
Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:
McDougal Littell
College Algebra
College Algebra
Algebra
ISBN:
9781337282291
Author:
Ron Larson
Publisher:
Cengage Learning
College Algebra
College Algebra
Algebra
ISBN:
9781938168383
Author:
Jay Abramson
Publisher:
OpenStax
Elementary Algebra
Elementary Algebra
Algebra
ISBN:
9780998625713
Author:
Lynn Marecek, MaryAnne Anthony-Smith
Publisher:
OpenStax - Rice University
College Algebra (MindTap Course List)
College Algebra (MindTap Course List)
Algebra
ISBN:
9781305652231
Author:
R. David Gustafson, Jeff Hughes
Publisher:
Cengage Learning
SEE MORE TEXTBOOKS