1. Consider the concentration cell below: Identify the anode, cathode, and the electron flow. Calculate the cell potential at 25 C. The [Ag*] in the right-hand beaker is 1.0x10 8 M. I Ag- Ag [Ag ]= 1.0 M
1. Consider the concentration cell below: Identify the anode, cathode, and the electron flow. Calculate the cell potential at 25 C. The [Ag*] in the right-hand beaker is 1.0x10 8 M. I Ag- Ag [Ag ]= 1.0 M
Chemistry
10th Edition
ISBN:9781305957404
Author:Steven S. Zumdahl, Susan A. Zumdahl, Donald J. DeCoste
Publisher:Steven S. Zumdahl, Susan A. Zumdahl, Donald J. DeCoste
Chapter1: Chemical Foundations
Section: Chapter Questions
Problem 1RQ: Define and explain the differences between the following terms. a. law and theory b. theory and...
Related questions
Question
Can another expert solve this and provide me with an answer?
![### Concentration Cell Analysis
#### Problem Statement:
Consider the concentration cell below: Identify the anode, cathode, and the direction of electron flow. Calculate the cell potential at 25°C. The \([Ag^+]\) in the right-hand beaker is \(1.0 \times 10^{-8} \, M\).

#### Diagram Explanation:
The diagram represents a concentration cell involving silver electrodes immersed in different concentrations of \( Ag^+ \) ions:
- **Left-hand Beaker**: Contains \( Ag \) electrode immersed in a silver nitrate solution where \([Ag^+] = 1.0 \, M\).
- **Right-hand Beaker**: Contains \( Ag \) electrode immersed in a silver nitrate solution where \([Ag^+] = 1.0 \times 10^{-8} \, M\).
The two beakers are connected by a salt bridge that allows for the movement of ions to maintain electrical neutrality.
#### Identification:
- **Anode (Oxidation)**: The anode is where oxidation occurs (loss of electrons). In this cell, it corresponds to the electrode in the low concentration solution.
- Therefore, the right-hand beaker (\([Ag^+] = 1.0 \times 10^{-8} \, M\)) is the **anode**.
- **Cathode (Reduction)**: The cathode is where reduction occurs (gain of electrons). It corresponds to the electrode in the high concentration solution.
- Therefore, the left-hand beaker (\([Ag^+] = 1.0 \, M\)) is the **cathode**.
- **Direction of Electron Flow**: Electrons will flow from the anode to the cathode. In this case, from the right-hand beaker to the left-hand beaker.
#### Calculation of Cell Potential:
Using the Nernst equation for a concentration cell:
\[ E_{\text{cell}} = E^\circ_{\text{cell}} - \frac{RT}{nF} \ln \frac{[Ag^+]_{\text{anode}}}{[Ag^+]_{\text{cathode}}} \]
Given:
- \( E^\circ_{\text{cell}} = 0 \) (for identical electrodes)
- \( R = 8.314 \, J \cdot K](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F7c13c2cd-7092-4d54-837b-d1e36936f099%2Fa97d6fbe-00b4-427f-8bdc-d301fc0981f3%2F5m8d4o.jpeg&w=3840&q=75)
Transcribed Image Text:### Concentration Cell Analysis
#### Problem Statement:
Consider the concentration cell below: Identify the anode, cathode, and the direction of electron flow. Calculate the cell potential at 25°C. The \([Ag^+]\) in the right-hand beaker is \(1.0 \times 10^{-8} \, M\).

#### Diagram Explanation:
The diagram represents a concentration cell involving silver electrodes immersed in different concentrations of \( Ag^+ \) ions:
- **Left-hand Beaker**: Contains \( Ag \) electrode immersed in a silver nitrate solution where \([Ag^+] = 1.0 \, M\).
- **Right-hand Beaker**: Contains \( Ag \) electrode immersed in a silver nitrate solution where \([Ag^+] = 1.0 \times 10^{-8} \, M\).
The two beakers are connected by a salt bridge that allows for the movement of ions to maintain electrical neutrality.
#### Identification:
- **Anode (Oxidation)**: The anode is where oxidation occurs (loss of electrons). In this cell, it corresponds to the electrode in the low concentration solution.
- Therefore, the right-hand beaker (\([Ag^+] = 1.0 \times 10^{-8} \, M\)) is the **anode**.
- **Cathode (Reduction)**: The cathode is where reduction occurs (gain of electrons). It corresponds to the electrode in the high concentration solution.
- Therefore, the left-hand beaker (\([Ag^+] = 1.0 \, M\)) is the **cathode**.
- **Direction of Electron Flow**: Electrons will flow from the anode to the cathode. In this case, from the right-hand beaker to the left-hand beaker.
#### Calculation of Cell Potential:
Using the Nernst equation for a concentration cell:
\[ E_{\text{cell}} = E^\circ_{\text{cell}} - \frac{RT}{nF} \ln \frac{[Ag^+]_{\text{anode}}}{[Ag^+]_{\text{cathode}}} \]
Given:
- \( E^\circ_{\text{cell}} = 0 \) (for identical electrodes)
- \( R = 8.314 \, J \cdot K
Expert Solution
Step 1
The Nernst equation is shown below:
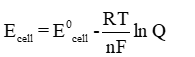
Where;
Ecell = electrode potential at any condition
E0cell = standard electrode potential
R = gas constant
T = temperature
n = no. of electrons transferred
F = Faraday's constant
Q = reaction quotient
Step 2
Given information:
E0Ag+/Ag = 0.80 V
The concentration of Ag+ in right hand compartment = 1.0×10-8 M
The concentration of Ag+ in left hand compartment = 1.0 M
Step 3
In galvanic cell; the left-hand compartment is taken as anode and the right-hand compartment is taken as cathode.
Trending now
This is a popular solution!
Step by step
Solved in 6 steps with 4 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, chemistry and related others by exploring similar questions and additional content below.Recommended textbooks for you

Chemistry
Chemistry
ISBN:
9781305957404
Author:
Steven S. Zumdahl, Susan A. Zumdahl, Donald J. DeCoste
Publisher:
Cengage Learning

Chemistry
Chemistry
ISBN:
9781259911156
Author:
Raymond Chang Dr., Jason Overby Professor
Publisher:
McGraw-Hill Education

Principles of Instrumental Analysis
Chemistry
ISBN:
9781305577213
Author:
Douglas A. Skoog, F. James Holler, Stanley R. Crouch
Publisher:
Cengage Learning

Chemistry
Chemistry
ISBN:
9781305957404
Author:
Steven S. Zumdahl, Susan A. Zumdahl, Donald J. DeCoste
Publisher:
Cengage Learning

Chemistry
Chemistry
ISBN:
9781259911156
Author:
Raymond Chang Dr., Jason Overby Professor
Publisher:
McGraw-Hill Education

Principles of Instrumental Analysis
Chemistry
ISBN:
9781305577213
Author:
Douglas A. Skoog, F. James Holler, Stanley R. Crouch
Publisher:
Cengage Learning

Organic Chemistry
Chemistry
ISBN:
9780078021558
Author:
Janice Gorzynski Smith Dr.
Publisher:
McGraw-Hill Education

Chemistry: Principles and Reactions
Chemistry
ISBN:
9781305079373
Author:
William L. Masterton, Cecile N. Hurley
Publisher:
Cengage Learning

Elementary Principles of Chemical Processes, Bind…
Chemistry
ISBN:
9781118431221
Author:
Richard M. Felder, Ronald W. Rousseau, Lisa G. Bullard
Publisher:
WILEY