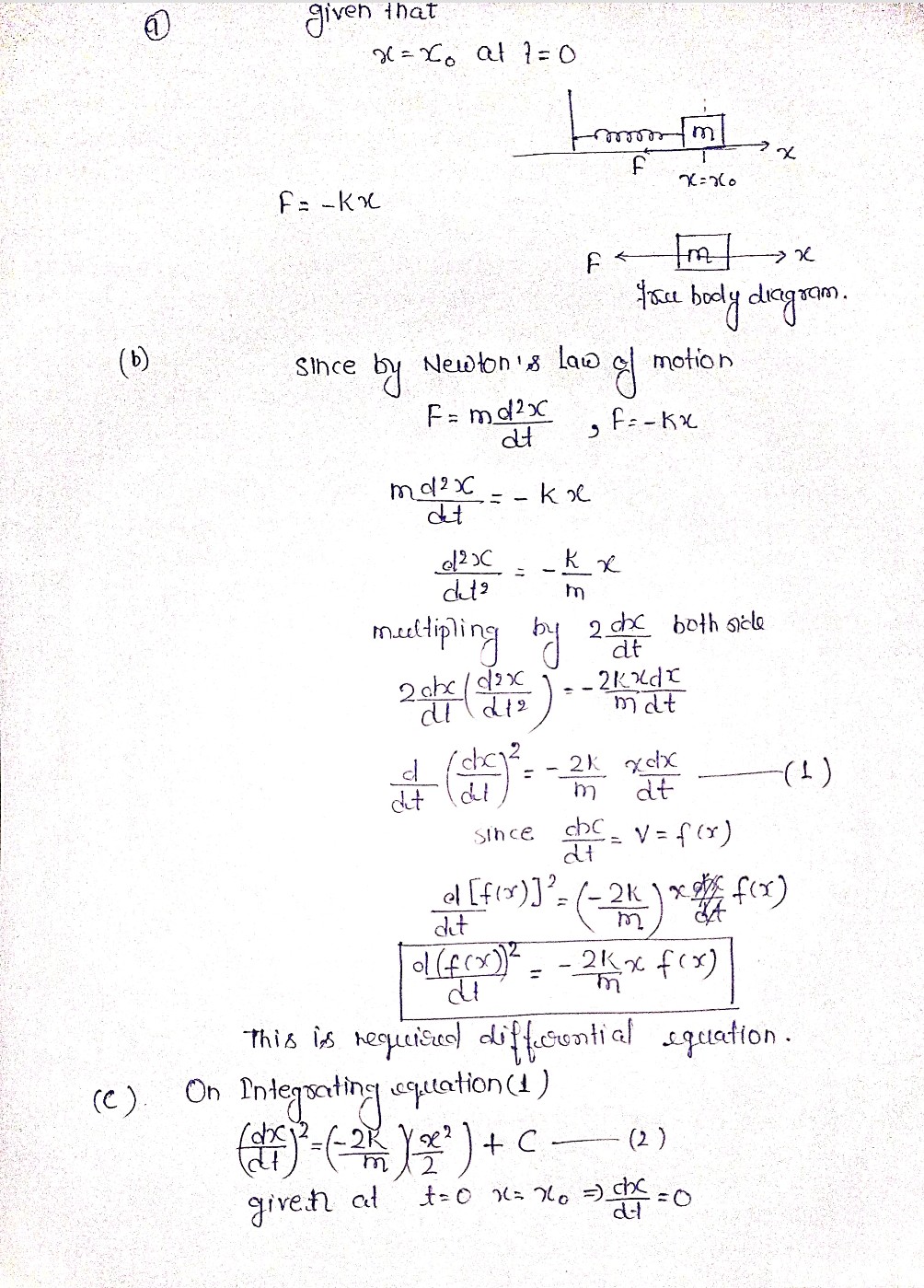
= [1] Consider a mass m constrained to move on the x-axis and subject to a net force F where k is a positive constant. The mass is released from rest at x = x, at time t = 0 s. -kx, (a) Draw a free-body diagram for the mass and indicate a coordinate system. (b) Write down Newton's 2nd Law (N2) for the mass, then convert it to first order differential equation for velocity. Solve for the velocity of the mass as a function of position, v = f(x). (c) Now use the result for velocity above in the form dx/dt = f(x) and solve for the position of the mass.
= [1] Consider a mass m constrained to move on the x-axis and subject to a net force F where k is a positive constant. The mass is released from rest at x = x, at time t = 0 s. -kx, (a) Draw a free-body diagram for the mass and indicate a coordinate system. (b) Write down Newton's 2nd Law (N2) for the mass, then convert it to first order differential equation for velocity. Solve for the velocity of the mass as a function of position, v = f(x). (c) Now use the result for velocity above in the form dx/dt = f(x) and solve for the position of the mass.
Related questions
Question
![=
[1] Consider a mass m constrained to move on the x-axis and subject to a net force F
where k is a positive constant. The mass is released from rest at x = x, at time t = 0 s.
-kx,
(a) Draw a free-body diagram for the mass and indicate a coordinate system.
(b) Write down Newton's 2nd Law (N2) for the mass, then convert it to first order differential
equation for velocity. Solve for the velocity of the mass as a function of position, v = f(x).
(c) Now use the result for velocity above in the form dx/dt = f(x) and solve for the position of
the mass.](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F0c015292-e614-4754-9a92-673e636d111d%2F60c51ed2-a004-4b3c-b98a-a1d20735ede9%2Fp7dk7em_processed.jpeg&w=3840&q=75)
Transcribed Image Text:=
[1] Consider a mass m constrained to move on the x-axis and subject to a net force F
where k is a positive constant. The mass is released from rest at x = x, at time t = 0 s.
-kx,
(a) Draw a free-body diagram for the mass and indicate a coordinate system.
(b) Write down Newton's 2nd Law (N2) for the mass, then convert it to first order differential
equation for velocity. Solve for the velocity of the mass as a function of position, v = f(x).
(c) Now use the result for velocity above in the form dx/dt = f(x) and solve for the position of
the mass.
Expert Solution
Step 1
The required solution is following.
Trending now
This is a popular solution!
Step by step
Solved in 2 steps with 2 images
