What are Z-parameters?

The Z-parameters that are the impedance parameters of a two-port network are defined by expressing the two-port voltages V₁ and V₂ in terms of two-port currents I₁ and I₂. The Z-parameters are calculated by open circuiting one of the two ports at a time henceforth they are called open-circuit impedance parameters.

Determination of Z-parameters

Let us consider a two-port network that has two pairs of terminals, one pair at the input known as an input port, and one pair at the output known as an output port as shown in the figure below. V₁ and V₂ are the terminal input and output voltages whereas I₁ and I₂ are input and output currents respectively.

The port voltages V₁ and V₂ are the dependent variables and port current I₁ and I₂ are independent variables.

$V_1{V}_{2=}f\left(I_1{I}_2\right)$

V₁=Z₁₁I₁+Z₁₂I₂………(1)

V₂=Z₂₁I₁+Z₂₂I₂………..(2)

Z₁₁, Z₁₂, Z₂₁, Z₂₂ are called the impedance parameters of the two-port network and are defined by equations (1) and (2).

These parameters can be represented by matrices. In matrix form, we can write

$\left[\begin{array}{c}{V}_1\\ V_2\end{array}\right]=\left[\begin{array}{cc}{Z}_{11}& Z_{12}\\ Z_{21}& Z_{22}\end{array}\right]\left[\begin{array}{c}{I}_1\\ I_2\end{array}\right]$

Each of the Z-parameters for the given two ports can be defined by making each of the port currents equal to zero that is open circuiting the port.

Case I: When the output port is open-circuited, that is I₂=0

By substituting I₂ =0 in equation (1) we get the Z-parameter matrix,

$Z_{11}=\frac{V_1}{I_1}{I}_{2=0}$

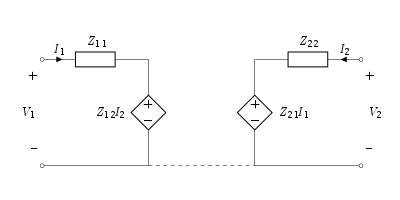
Where Z₁₁ is the driving point impedance at the input port with output port open-circuited. Z₁₁ is the ratio of input port voltage and input port current and is called open circuit input impedance.

Similarly, by substituting I₂=0 in equation (2) we get the z-matrix,

$Z_{21}=\frac{V_2}{I_1}{I}_{2=0}$

Where Z₂₁ is the transfer impedance at the input port with the output port open-circuited. Z₂₁ is the ratio of output port voltage to input port current and is called open-circuit forward transfer impedance.

Case II: When the input port is open-circuited, that is I₁=0

By substituting I₁ =0 in equation (1) we get the z-matrix,

$Z_{12}=\frac{V_2}{I_2}{I}_{1=0}$

Where Z₁₂ is the transfer impedance at the output port with the input port open-circuited. Z₁₂ is the ratio of input port voltage to output port current and is called open-circuit reverse transfer impedance.

Similarly, by substituting I₂=0 in equation (2) we get the z-matrix,

$Z_{22}=\frac{V_2}{I_2}{I}_{1=0}$

where Z₂₂ is the open-circuit driving-point impedance at the output port with the input port open-circuited. Z₂₂ is the ratio of output port voltage to output port current and is called open circuit output impedance.

The equivalent circuit of the two-port network in terms of Z-parameters is shown in the figure below.

Condition for Reciprocity

A two-port network is said to be reciprocal if the ratio of excitation at one port to response at the other port is the same if excitation and response are interchanged. Consequently, we can write,

$\frac{V_2}{I_1}{I}_{2=0}=\frac{V_1}{I_2}{I}_{1=0}$

Z₂₁=Z₁₂

Condition for Symmetry

A network is said to be symmetrical if the voltage to current ratio at one port is the same as the voltage to current ratio at the other port with one of the ports open-circuited. Consequently, we can write

$\frac{V_1}{I_1}{I}_{2=0}=\frac{V_2}{I_2}{I}_{1=0}$

Z₁₁=Z₂₂

The network is symmetrical implies that the network has a mirror-like symmetry about the centerline, that is, a line can be drawn which will divide the network into two symmetrical halves.

Inter-relationships between the parameters

For a particular network to find two or more parameters will be tedious. Conversely, if we find a particular parameter then the other parameters can be found if the interrelationship between them is known. The relation between Z-parameters and other parameters that is Y parameters, ABCD parameters, and hybrid parameters as mentioned below:

Expression of Z-parameters in terms of Y parameters

The equations for Y parameters are given by

I₁=Y₁₁V₁+Y₁₂V₂

I₂ =Y₂₁V₁+Y₂₂V₂

Comparing with the equations of Z parameter we get,

$$\begin{gathered} Z_{11}=\frac{Y_{22}}{∆} \\ {Z}_{12}=\frac{-Y_{12}}{∆} \\ {Z}_{21}=\frac{-Y_{21}}{∆} \\ {Z}_{22}=\frac{Y_{11}}{∆} \end{gathered}$$

Wherein Δ is determinant of Y matrix, $∆=Y_{11}{Y}_{22}-Y_{12}{Y}_{21}$

Expression of Z parameter in terms of ABCD parameters

T-parameters are known as transmission parameters or ABCD parameters. The equations for ABCD parameters are given by

V₁=AV₂-BI₂

I₁=CV₂-DI₂

Rearranging the equation and comparing it with the equation of Z parameter we get,

$$\begin{gathered} Z_{11}=\frac{A}{C} \\ {Z}_{12}=\frac{AD-BC}{C} \\ {Z}_{21}=\frac{1}{C} \\ {Z}_{22}=\frac{D}{C} \end{gathered}$$

Expression of Z-parameters in terms of Hybrid parameters

The equations of Hybrid parameters or h-parameters are given by

V₁=h₁₁I₁+h₁₂V₂

I₂=h₂₁I₁ +h₂₂V₂

Rearranging the equation and comparing with equation of Z parameter we get,

$$\begin{gathered} Z_{11}=\frac{∆h}{h_{22}} \\ {Z}_{12}=\frac{h_{12}}{h_{22}} \\ {Z}_{21}=\frac{-h_{21}}{h_{22}} \\ {Z}_{22}=\frac{1}{h_{22}} \end{gathered}$$

wherein Δh =h₁₁h₂₂-h₁₂h₂₁

Series connection of two-port network

In a two-port network, various types of interconnections such as series, parallel, and cascade are done.

• When two two-ports networks are connected in series, their Z-parameters are added to get the overall Z parameter of the series connection.

• When two two-port networks are connected in parallel, their Y parameters are added to get the overall Y parameter of the parallel connection.

• When two two-ports networks are connected in a cascade, their transmission parameters are multiplied to get the overall transmission parameter.

Let us consider two two-port networks connected in series as shown in Figure below.

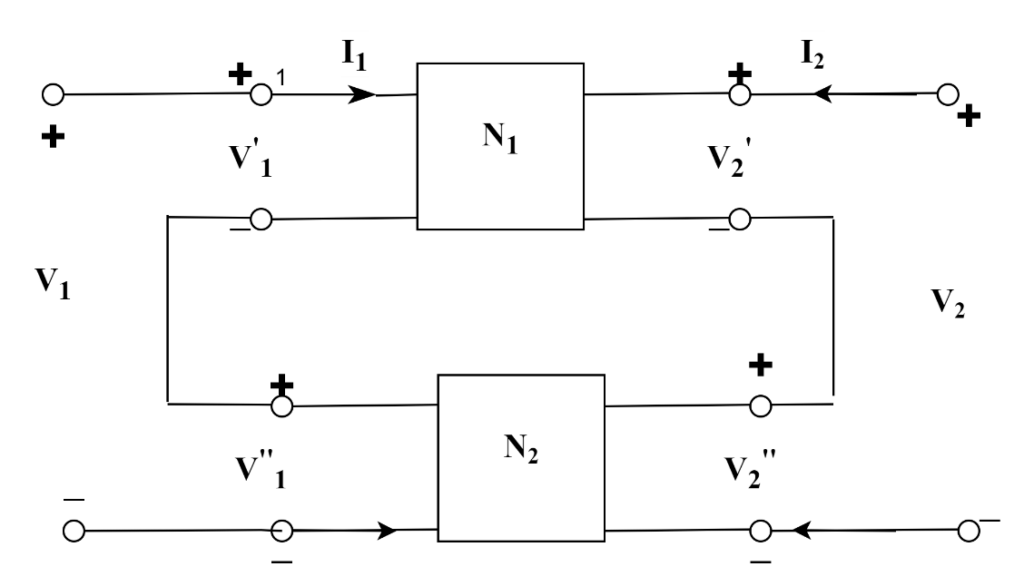
Let the impedance parameter of network N₁ be Z₁₁’, Z₁₂’, Z’₂₁, Z₂₂’ and that of network N₂ be Z₁₁’, Z₁₂’, Z₂₁’’, Z₂₂’’.

For network N₁

V'₁=Z'₁₁+Z'₁₂I₂

V'₂=Z'₂₁I₁+Z'₂₂I₂

For network N₂

V''₁=Z''₁₁+Z''₁₂I₂

V''₂=Z''₂₁I₁+Z''₂₂I₂

For a combined network V₁=V₁’+V₁’’and V₂=V₂’+V₂’’

Consequently, the resultant Z-parameters will be the sum of the Z parameter of each individual two-port network connected.

Z₁₁=Z₁₁’+Z₁₁’’

Z₁₂=Z₁₂’+Z₁₂’’

Z₂₁=Z₂₁’+Z₂₁’’

Z₂₂=Z₂₂’+Z₂₂’’

Reflection Coefficient

In a transmission medium, when a wave is reflected because of discontinuity in impedance, we use the reflection coefficient to find the magnitude of reflection. It is a ratio of the amplitude of the reflected wave to the incident wave.

Context and Applications

Impedance parameter is the widely used concept in design and analysis of electrical network which is an essential topic for all competitive exams and studies related to:

• Bachelors in Technology (Electrical)
• Bachelors in Technology (Electronics)
• Masters in Technology (Electrical)

Practice Problems

1. Which are the parameters obtained by multiplying the individual parameters of a two two-port network that are connected in cascade?

1. Hybrid parameters 

2. Admittance parameters

3. Transmission parameters

4. ABCD parameters

Correct option- c

Explanation: When two two-port are connected in a cascade, their transmission parameters are multiplied to get the overall transmission parameter.  

2. A two-port network is defined by the following two equations I₁=2V₁+V₂ and I₂=V₁+V₂

Its impedance parameters (Z₁₁, Z₁₂, Z₂₁, Z₂₂) are given by :

1. 0.5, 1, 1, 1
2. 1, 1, 1, 2
3. 1, -1, -1, 2
4. 2, -1, -1, 1

Correct option- c

Explanation: Here Y₁₁= 2, Y₁₂ = 1, Y₂₁=1, Y₂₂=1

Δ=Y₁₁Y₂₂-Y₁₂Y₂₁ = 2*1-1*1 =1

$$\begin{gathered} Z_{11}=\frac{Y_{22}}{∆}=\frac{1}{1}=1 \\ {Z}_{12}=\frac{-Y_{12}}{∆}=\frac{-1}{1}=-1 \\ {Z}_{21}=\frac{-Y_{21}}{∆}=\frac{-1}{1}=-1 \\ {Z}_{22}=\frac{Y_{11}}{∆}=\frac{2}{1}=2 \end{gathered}$$

3. Which of the following is known as transfer impedance at the input port? 

1. Z₁₂
2. Z₂₁
3. Z₂₂
4. Both a and b 

Correct option: b

Explanation: Z₂₁ is the transfer impedance at the input port with the output port open-circuited.

4.The impedance matrices of two two-port networks are given by [Z_x ]=$\left[\begin{array}{cc}3& -2\\ 4& 8\end{array}\right]$ and [Z_y]=$\left[\begin{array}{cc}13& -2\\ 8& 10\end{array}\right]$

If these two networks are connected in series, which of the following correctly represents the impedance matrix of the resulting two-port network?

1. $\left[\begin{array}{cc}39& 4\\ 32& 80\end{array}\right]$
2. $\left[\begin{array}{cc}16& 0\\ 12& 18\end{array}\right]$
3. $\left[\begin{array}{cc}16& -4\\ 12& 18\end{array}\right]$
4. $\left[\begin{array}{cc}-10& -4\\ -4& -2\end{array}\right]$

Correct option: b 

Explanation: When the two ports are connected in series, their Z-parameters can be added to get the overall Z parameter of the series connection. 

5. The Z-parameters of a two-port network are $\left[\begin{array}{cc}10& -2\\ -2& 10\end{array}\right]$. The two-port network is:

1. Symmetrical
2. Reciprocal
3. Both symmetrical and reciprocal
4. Can't be determined

Explanation: For a network to be symmetrical Z₁₁= Z₂₂, here Z₁₁ =Z₂₂=10, also for a network to be reciprocal Z₁₂=Z_21, here Z₁₂=Z₂₁=-2

Related Concepts

• Y parameters of two-port network
• Smith chart
• N-port parameters
• T and π representations
• Terminated two-port network