
Concept explainers
Solve by graphing:
To calculate: The solution of the inequality
Answer to Problem 1DE
Solution:
The solution of the inequality
Explanation of Solution
Given Information:
The provided inequality is
Formula used:
Steps to solve the inequality by graphing.
1. Replace the inequality sign by equal sign.
2. Find the leading coefficient in the inequality.
If the leading coefficient in the inequality is negative, then the graph opens down and if the leading coefficient is positive, then the graph opens up.
3. Set the polynomial equal to zero to find the x-intercept and also find y-intercept.
4. Plot the graph of the equation.
Zero product rule is such that if
Calculation:
Consider the inequality,
Now, the inequality
So, the equation becomes
Put the equation equals to zero to find the x-intercepts,
Use the zero product rule and simplify the above equation as,
The x-intercepts are
The leading coefficient in the polynomial
Now, find the point where the graph intersects y-axis.
Substitute
So, the y-intercept is
The table provided below shows the values of y for different values of x,
The graph of the equation
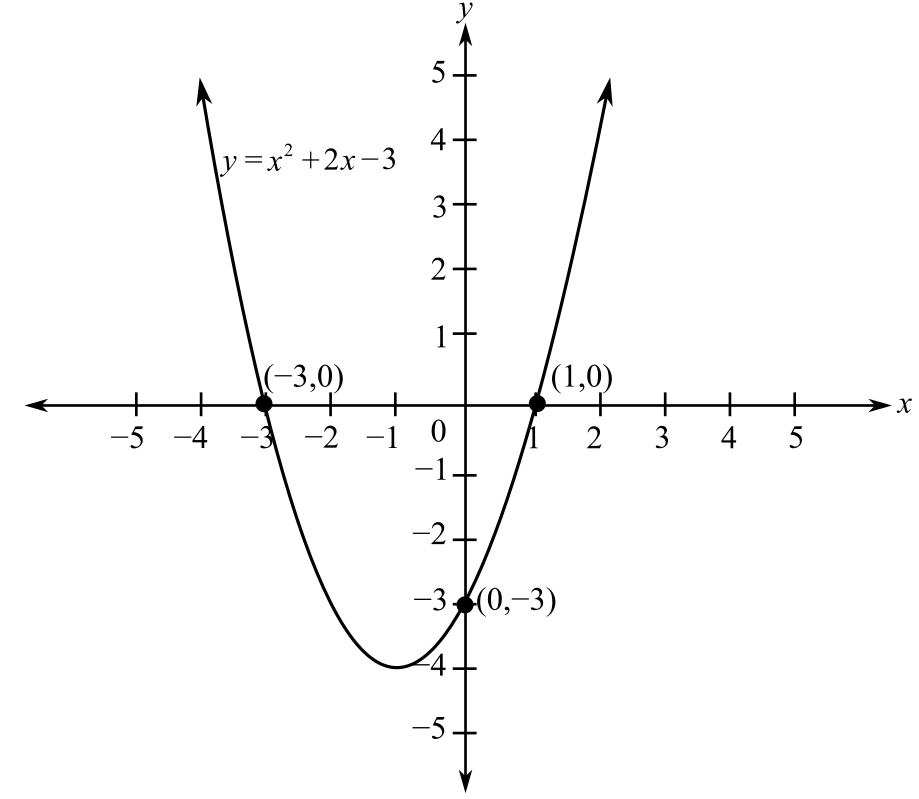
From the observation of the graph, the values of y is positive when
So, the values for which y be positive are
Therefore, the solution of the inequality
Want to see more full solutions like this?
Chapter L Solutions
Developmental Mathematics (9th Edition)
- 5 (Moments of GARCH) For the GARCH(2,2) model rt = 0.2+0.25u1+0.05u-2 +0.30% / -1 +0.20% -2, find cov(rt). 0.0035 ut, ut = στει,στ =arrow_forward(14 points) Let S = {(x, y, z) | z = e−(x²+y²), x² + y² ≤ 1}. The surface is the graph of ze(+2) sitting over the unit disk.arrow_forwardDoor 87.5in to 47 living 44.75 Closet 96in Window ISS.Sin 48in Train Table 96in 48in 132:2 Windowarrow_forward
- Classify the singularities for the following functions at the given point. at a = (a) f(z) = 1 (2 sin z-1)² (b) f(z) = exp(4)-1 at 0 and at a = (c) f(z) = 1-cosh z at a=0 2 In the case of a pole, indicate the order of the pole and its residue.arrow_forwardDefinition of null hypothesis from the textbook Definition of alternative hypothesis from the textbook Imagine this: you suspect your beloved Chicken McNugget is shrinking. Inflation is hitting everything else, so why not the humble nugget too, right? But your sibling thinks you’re just being dramatic—maybe you’re just extra hungry today. Determined to prove them wrong, you take matters (and nuggets) into your own hands. You march into McDonald’s, get two 20-piece boxes, and head home like a scientist on a mission. Now, before you start weighing each nugget like they’re precious gold nuggets, let’s talk hypotheses. The average weight of nuggets as mentioned on the box is 16 g each. Develop your null and alternative hypotheses separately. Next, you weigh each nugget with the precision of a jeweler and find they average out to 15.5 grams. You also conduct a statistical analysis, and the p-value turns out to be 0.01. Based on this information, answer the following questions. (Remember,…arrow_forwardBusiness Discussarrow_forward
- Determine all functions f analytic in the open unit disc || < 1 which satisfy in addition f(0) = 1 and |f(z)|≥ 1 whenever || < 1. Justify your answer.arrow_forwardDeduce the Laurent expansion for f(z) = 22(2-3)2 in the annulus 0 < |z3|< 3.arrow_forwardWhat can you conclude about a complex-valued function f(z) that satisfies 1. f is complex differentiable everywhere 2. ƒ(z+1) = ƒ(z) for all z 3. For a fixed complex number a with nonzero imaginary part, f(z+a) = f(z) for all z ? Justify your answer. (Hint: Use Liouville's theorem.)arrow_forward
- 6. Solve the system of differential equations using Laplace Transforms: x(t) = 3x₁ (t) + 4x2(t) x(t) = -4x₁(t) + 3x2(t) x₁(0) = 1,x2(0) = 0arrow_forward3. Determine the Laplace Transform for the following functions. Show all of your work: 1-t, 0 ≤t<3 a. e(t) = t2, 3≤t<5 4, t≥ 5 b. f(t) = f(tt)e-3(-) cos 4τ drarrow_forward4. Find the inverse Laplace Transform Show all of your work: a. F(s) = = 2s-3 (s²-10s+61)(5-3) se-2s b. G(s) = (s+2)²arrow_forward
 Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
 Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
 Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell





