Chapter B, Problem 10RP

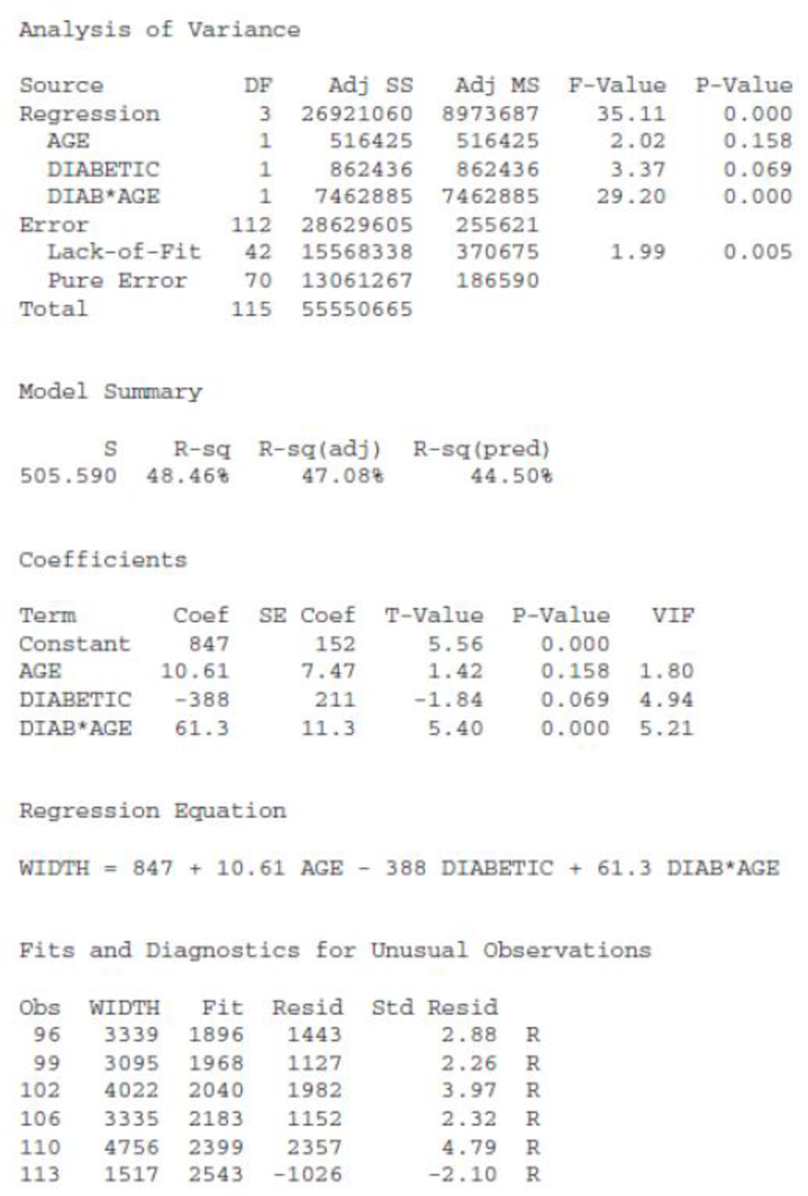
OUTPUT B.95 Output for Problem 10

Regression Analysis: WIDTH versus AGE, DIABETIC, DIAB*AGE

R Large residual

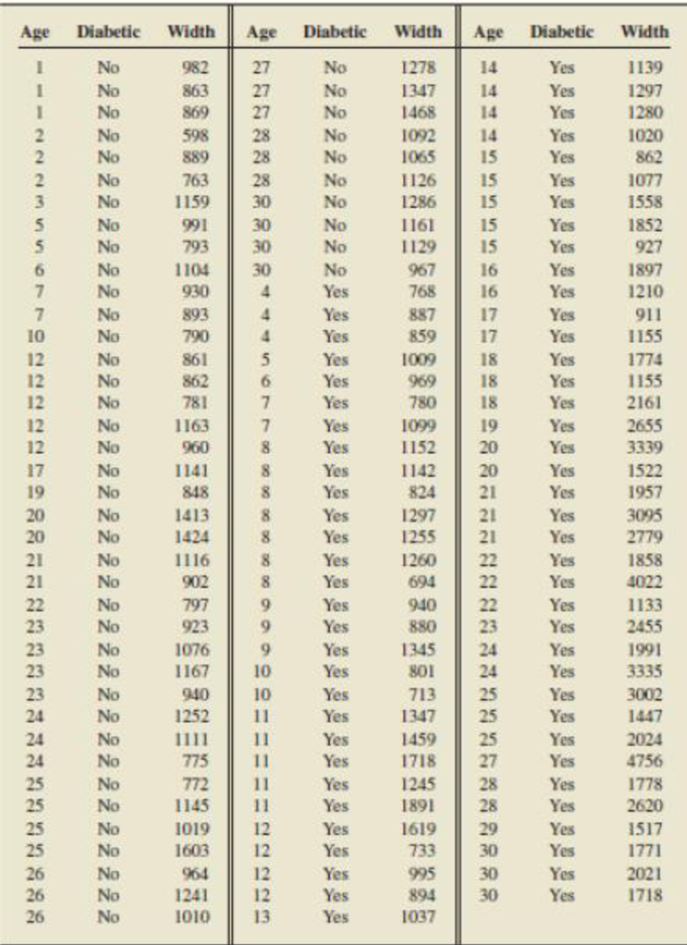
10. Diabetes. In the research article “Capillary Basement Membrane Width in Diabetic Children" (American Journal of Medicine, 58. pp. 365–372), P. Raskin et al. obtained data on age and on width of the quadriceps muscle capillary basement membrane of individuals with and without diabetes. The membrane width can be used to diagnose the presence of diabetic microangiopathy. The table below provides the data obtained by the researchers. We want to predict membrane width based on age and whether the person is a diabetic. We introduce the indicator variable diabetic defined by

$diabetic=\{\begin{array}{c}1,\text{if}\text{person}\text{has}\text{diabetes};\\ 0,\text{if}\text{person}\text{doesnot}\text{have}\text{diabetes}.\end{array}$

1. a. Output B .91 on page B-170 shows a plot of width versus age, with the plot symbol being a solid black circle for diabetics and an open circle for non-diabetics. Based on this plot does it appear that diabetic is a useful predictor variable? Explain your answer.
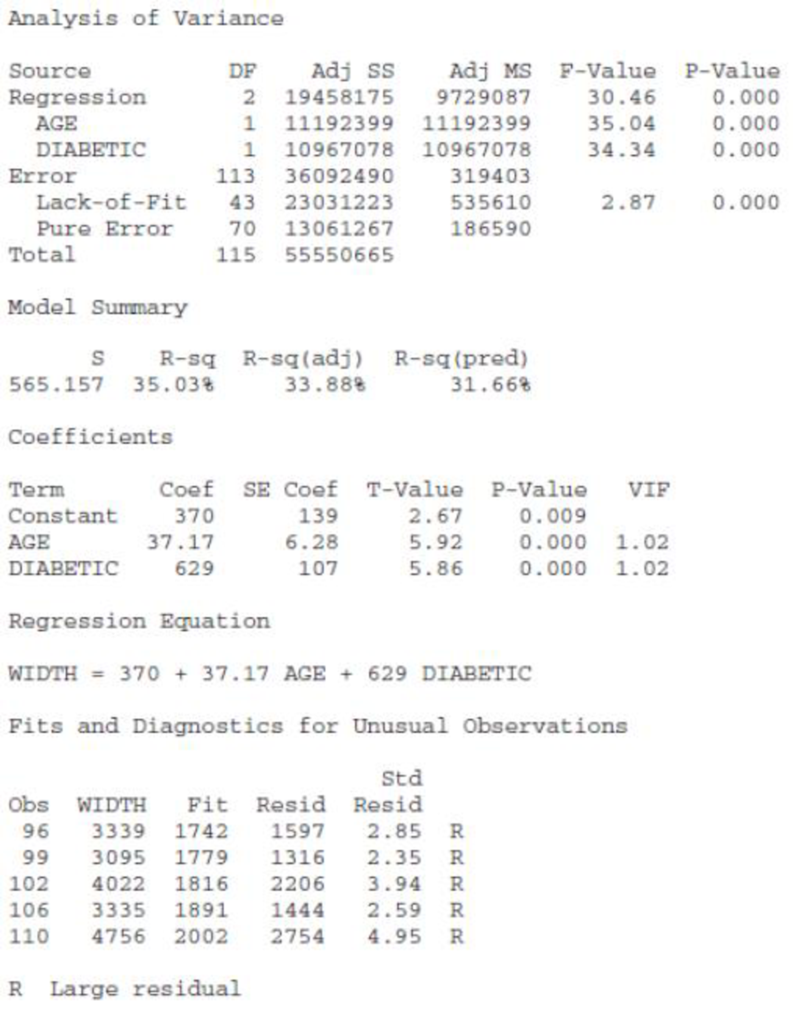
2. b. We obtained the regression analysis of width on age and diabetic shown in Output B.92 on page B-170. Conduct the t-tests for the individual utility of each of the two predictor variables. Use a 5% level of significance and interpret your results.
3. c. Based on Output B.92, obtain the regression equations relating width to age for diabetics and non-diabetics, separately.
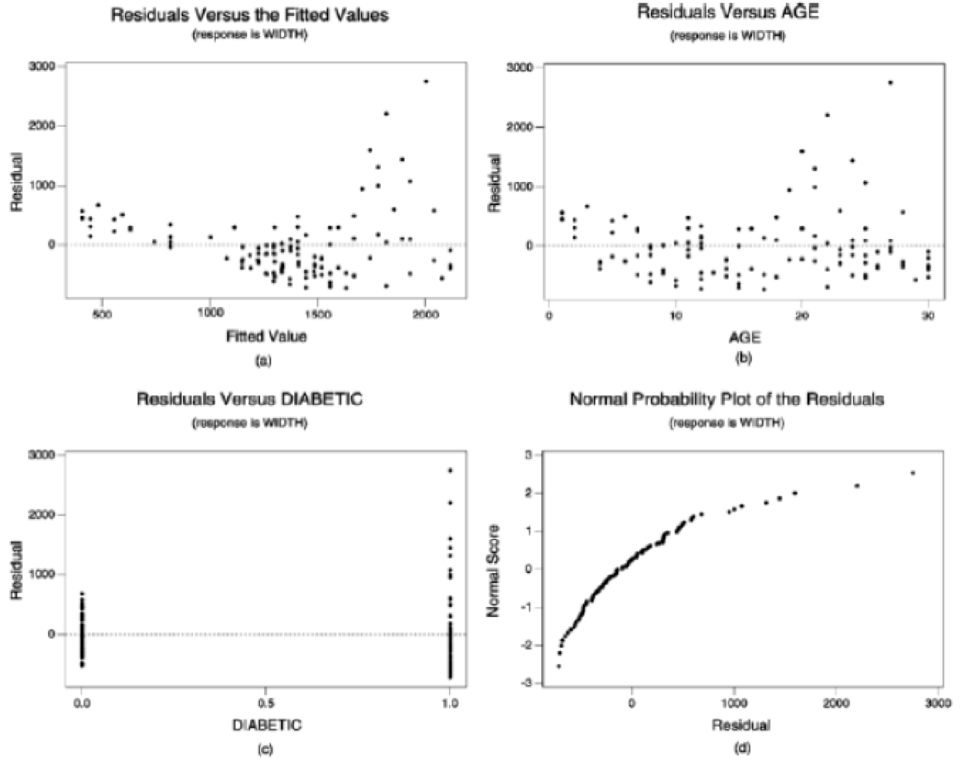
4. d. Outputs B.93(a), (b), (c), and (d), given on page B-171, provide, respectively, plots of residuals versus fitted values, residuals versus age, residuals versus diabetic, and a normal probability plot of the residuals. Perform a residual analysis to assess the appropriateness of the regression equation, constancy of the conditional standard deviations, and normality of the conditional distributions. Check for outliers and influential observations.

Table for Problem 10

OUTPUT B.91 Scatterplot for Problem 10

OUTPUT B.92 Output for Problem 10

Regression Analysis: WIDTH versus AGE, DIABETIC

OUTPUT B.93 Residual plots for Problem 10

1. e. Output B.94 provides a plot of width versus age with regression lines for diabetics and non-diabetics. Based on this output and your residual analysis in part (d), do you feel that the model fits the data well? Explain your answer.
2. f. To check for interaction between the two predictor variables, we obtained the regression analysis of width on age, diabetic, and diabetic-age. The output is given in Output B.95 on page B-172. Is there an interaction between age and diabetic? Use α = 0.05.
3. g. What other analyses should be performed on these data? Explain your answer.