
College Algebra (10th Edition)
10th Edition
ISBN: 9780321979476
Author: Michael Sullivan
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Textbook Question
Chapter A.1, Problem 4E
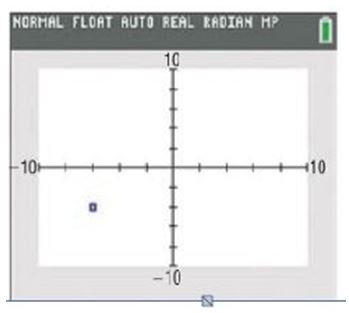
In problems 1-4, determine the coordinates of the points shown. Tell in which quadrant each point lies.
Assume that the coordinates are integers.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
A research study in the year 2009 found that there were 2760 coyotes
in a given region. The coyote population declined at a rate of 5.8%
each year.
How many fewer coyotes were there in 2024 than in 2015?
Explain in at least one sentence how you solved the problem. Show
your work. Round your answer to the nearest whole number.
Answer the following questions related to the following matrix
A =
3
³).
Explain the following terms
Chapter A Solutions
College Algebra (10th Edition)
Ch. A.1 - In problems 1-4, determine the coordinates of the...Ch. A.1 - In problems 1-4, determine the coordinates of the...Ch. A.1 - In problems 1-4, determine the coordinates of the...Ch. A.1 - In problems 1-4, determine the coordinates of the...Ch. A.1 - In Problems 5-10, determine the viewing window...Ch. A.1 - In Problems 5-10, determine the viewing window...Ch. A.1 - In Problems 5-10, determine the viewing window...Ch. A.1 - In Problems 5-10, determine the viewing window...Ch. A.1 - In Problems 5-10, determine the viewing window...Ch. A.1 - In Problems 5-10, determine the viewing window...
Ch. A.1 - In Problems 11-16, select a setting so that each...Ch. A.1 - In Problems 11-16, select a setting so that each...Ch. A.1 - In Problems 11-16, select a setting so that each...Ch. A.1 - In Problems 11-16, select a setting so that each...Ch. A.1 - In Problems 11-16, select a setting so that each...Ch. A.1 - In Problems 11-16, select a setting so that each...Ch. A.2 - In Problems 1-16, graph each equation using the...Ch. A.2 - In Problems 1-16, graph each equation using the...Ch. A.2 - In Problems 1-16, graph each equation using the...Ch. A.2 - In Problems 1-16, graph each equation using the...Ch. A.2 - In Problems 1-16, graph each equation using the...Ch. A.2 - In Problems 1-16, graph each equation using the...Ch. A.2 - In Problems 1-16, graph each equation using the...Ch. A.2 - In Problems 1-16, graph each equation using the...Ch. A.2 - In Problems 1-16, graph each equation using the...Ch. A.2 - In Problems 1-16, graph each equation using the...Ch. A.2 - In Problems 1-16, graph each equation using the...Ch. A.2 - In Problems 1-16, graph each equation using the...Ch. A.2 - In Problems 1-16, graph each equation using the...Ch. A.2 - In Problems 1-16, graph each equation using the...Ch. A.2 - In Problems 1-16, graph each equation using the...Ch. A.2 - In Problems 1-16, graph each equation using the...Ch. A.2 - For each of the above equations, create a table,...Ch. A.2 - For each of the above equations, create a table,...Ch. A.2 - For each of the above equations, create a table,...Ch. A.2 - For each of the above equations, create a table,...Ch. A.2 - For each of the above equations, create a table,...Ch. A.2 - For each of the above equations, create a table,...Ch. A.2 - For each of the above equations, create a table,...Ch. A.2 - For each of the above equations, create a table,...Ch. A.2 - For each of the above equations, create a table,...Ch. A.2 - For each of the above equations, create a table,...Ch. A.2 - For each of the above equations, create a table,...Ch. A.2 - For each of the above equations, create a table,...Ch. A.2 - For each of the above equations, create a table,...Ch. A.2 - For each of the above equations, create a table,...Ch. A.2 - For each of the above equations, create a table,...Ch. A.2 - For each of the above equations, create a table,...Ch. A.3 - In Problems 1-6, use ZERO (or ROOT) to approximate...Ch. A.3 - In Problems 1-6, use ZERO (or ROOT) to approximate...Ch. A.3 - In Problems 1-6, use ZERO (or ROOT) to approximate...Ch. A.3 - In Problems 1-6, use ZERO (or ROOT) to approximate...Ch. A.3 - In Problems 1-6, use ZERO (or ROOT) to approximate...Ch. A.3 - In Problems 7-12, use ZERO (or ROOT) to...Ch. A.3 - In Problems 7-12, use ZERO (or ROOT) to...Ch. A.3 - In Problems 7-12, use ZERO (or ROOT) to...Ch. A.3 - In Problems 7-12, use ZERO (or ROOT) to...Ch. A.3 - In Problems 7-12, use ZERO (or ROOT) to...Ch. A.3 - In Problems 7-12, use ZERO (or ROOT) to...Ch. A.3 - In Problems 7-12, use ZERO (or ROOT) to...Ch. A.5 - In Problems 1-8, determine which of the given...Ch. A.5 - In Problems 1-8, determine which of the given...Ch. A.5 - In Problems 1-8, determine which of the given...Ch. A.5 - In Problems 1-8, determine which of the given...Ch. A.5 - In Problems 1-8, determine which of the given...Ch. A.5 - In Problems 1-8, determine which of the given...Ch. A.5 - In Problems 1-8, determine which of the given...Ch. A.5 - In Problems 1-8, determine which of the given...Ch. A.5 - If Xmin=4 , Xmax=12 , and Xscl=1 , how should...Ch. A.5 - If Xmin=6 , Xmax=10 , and Xscl=2 , how should...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, algebra and related others by exploring similar questions and additional content below.Similar questions
- Solve questions by Course Name (Ordinary Differential Equations II 2)arrow_forwardplease Solve questions by Course Name( Ordinary Differential Equations II 2)arrow_forwardInThe Northern Lights are bright flashes of colored light between 50 and 200 miles above Earth. Suppose a flash occurs 150 miles above Earth. What is the measure of arc BD, the portion of Earth from which the flash is visible? (Earth’s radius is approximately 4000 miles.)arrow_forward
- e). n! (n - 1)!arrow_forwardSuppose you flip a fair two-sided coin four times and record the result. a). List the sample space of this experiment. That is, list all possible outcomes that could occur when flipping a fair two-sided coin four total times. Assume the two sides of the coin are Heads (H) and Tails (T).arrow_forwarde). n! (n - 1)!arrow_forward
- Evaluate the following expression and show your work to support your calculations. a). 6! b). 4! 3!0! 7! c). 5!2! d). 5!2! e). n! (n - 1)!arrow_forwardAmy and Samiha have a hat that contains two playing cards, one ace and one king. They are playing a game where they randomly pick a card out of the hat four times, with replacement. Amy thinks that the probability of getting exactly two aces in four picks is equal to the probability of not getting exactly two aces in four picks. Samiha disagrees. She thinks that the probability of not getting exactly two aces is greater. The sample space of possible outcomes is listed below. A represents an ace, and K represents a king. Who is correct?arrow_forwardConsider the exponential function f(x) = 12x. Complete the sentences about the key features of the graph. The domain is all real numbers. The range is y> 0. The equation of the asymptote is y = 0 The y-intercept is 1arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
 Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage, Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill College AlgebraAlgebraISBN:9781305115545Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
College AlgebraAlgebraISBN:9781305115545Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage Learning

Elementary Geometry For College Students, 7e
Geometry
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Cengage,

Holt Mcdougal Larson Pre-algebra: Student Edition...
Algebra
ISBN:9780547587776
Author:HOLT MCDOUGAL
Publisher:HOLT MCDOUGAL

Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill

College Algebra
Algebra
ISBN:9781305115545
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning

Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell

Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781305652224
Author:Charles P. McKeague, Mark D. Turner
Publisher:Cengage Learning
What are the Different Types of Triangles? | Don't Memorise; Author: Don't Memorise;https://www.youtube.com/watch?v=1k0G-Y41jRA;License: Standard YouTube License, CC-BY
Law of Sines AAS, ASA, SSA Ambiguous Case; Author: Mario's Math Tutoring;https://www.youtube.com/watch?v=FPVGb-yWj3s;License: Standard YouTube License, CC-BY
Introduction to Statistics..What are they? And, How Do I Know Which One to Choose?; Author: The Doctoral Journey;https://www.youtube.com/watch?v=HpyRybBEDQ0;License: Standard YouTube License, CC-BY
Triangles | Mathematics Grade 5 | Periwinkle; Author: Periwinkle;https://www.youtube.com/watch?v=zneP1Q7IjgQ;License: Standard YouTube License, CC-BY
What Are Descriptive Statistics And Inferential Statistics?; Author: Amour Learning;https://www.youtube.com/watch?v=MUyUaouisZE;License: Standard Youtube License