
To calculate: The sine function that model the curve.
Answer to Problem 1RE
Solution:
The sine function of the curve is
Explanation of Solution
Given Information:
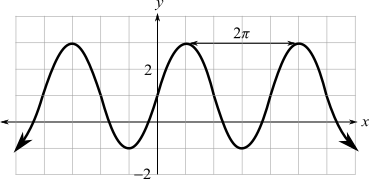
The provided curve is:

Formula Used:
The value of Amplitude in the sine function is the height of peak above the baseline and named it as A.
Then the vertical offset is the height of the baseline and it is named as C.
The angular frequency is the number of cycles in every interval of length
The period or wavelength is the length of each cycle which is given by
Then
The sine function is given by
Calculation:
Consider the provided curve.
Determine the value of Amplitude which is the height of peak above the baseline.
Then determine the vertical offset which is the height of the baseline.
Where h is the total height of the peak and A is Amplitude.
Then determine the period or wavelength which is the length of each cycle.
Then determine the angular frequency which is the number of cycles in every interval of length
Then determine the phase shift which is the distance to which the function is shifted horizontally.
Then substitute the values in the sine function given by
Hence, the sine function of the curve is
Want to see more full solutions like this?
Chapter 9 Solutions
Bundle: Applied Calculus, Loose-leaf Version, 7th + Webassign Printed Access Card For Waner/costenoble's Applied Calculus, 7th Edition, Single-term
- Calculus lll May I please have the solution for the following exercise? Thank you so mucharrow_forwardCalculus lll May I please have the solution for the following exercise? Thank you so much,arrow_forwardCalculus lll May I please have the solution for the following exercise? Thank you so mucharrow_forward
- Calculus lll May I please have the solution for the following example? Thank youarrow_forwardCalculus lll May I please have the statement completed for the following box? Thank you so much,arrow_forwardCalculus lll May I please have the solution for the following exercise? Thank you so mucharrow_forward
- Use a graphing calculator to find where the curves intersect and to find the area between the curves. y=ex, y=-x²-4x a. The left point of intersection is (Type integers or decimals rounded to the nearest thousandth as needed. Type an ordered pair.)arrow_forwardFind the area between the curves. x= -5, x=3, y=2x² +9, y=0 The area between the curves is (Round to the nearest whole number as needed.)arrow_forwardcan you solve these questions with step by step with clear explaination pleasearrow_forward
- Find the area between the following curves. x=-1, x=3, y=x-1, and y=0 The area between the curves is (Simplify your answer.)arrow_forwardFind the area between the curves. x= − 2, x= 3, y=5x, y=x? - 6 6 The area between the curves is (Simplify your answer.) ...arrow_forwardplease question 9arrow_forward
- Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
 Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning, Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning  Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage Learning





