
Concept explainers
Determine the centroid
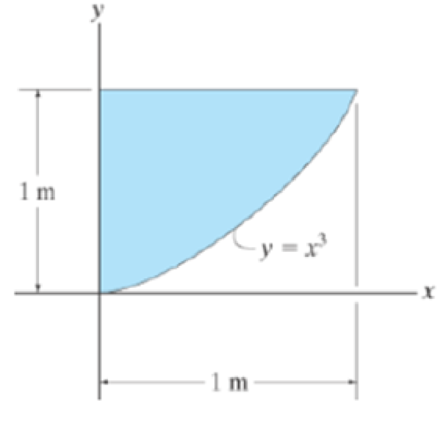
Prob. F9-1
(a)
The centroid
Answer to Problem 1FP
The centroid
Explanation of Solution
Given:
The length of the shaded area is 1 m.
The height of the shaded area is 1 m.
Show the area of the differential element as in Figure 1.
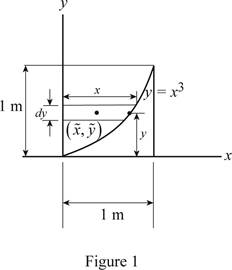
Using Figure 1,
Express the parabolic value.
Compute the area of the element.
Substitute
Compute the centroid of the differential element along the x-axis using the formula.
Substitute
Determine the location of the centre of gravity of the homogeneous rod along the x-axis
Conclusion:
Apply the limits from 0 to 1 m, substitute
Calculate the numerator part from Equation (III).
Calculate the denominator part from Equation (III).
Substitute
Thus, the centroid
(b)
The centroid
Answer to Problem 1FP
The centroid
Explanation of Solution
Given:
The length of the shaded area is 1 m.
The height of the shaded area is 1 m.
Show the area of the differential element as in Figure 2.
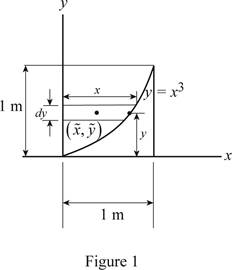
Using Figure 2,
Express the parabolic value.
Compute the area of the element.
Substitute
Compute the centroid of the differential element along the y-axis using the formula.
Determine the location of the centre of gravity of the homogeneous rod along the y-axis
Conclusion:
Apply the limits from 0 to 1 m, substitute
Calculate the numerator part from Equation (V).
Calculate the denominator part from Equation (V).
Substitute
Thus, the centroid
Want to see more full solutions like this?
Chapter 9 Solutions
PEARSON ETEXT ENGINEERING MECH & STATS
- Auto Controls Hand sketch the root Focus of the following transfer function How many asymptotes are there ?what are the angles of the asymptotes?Does the system remain stable for all values of K NO COPIED SOLUTIONSarrow_forward-400" 150" in Datum 80" 90" -280"arrow_forwardUsing hand drawing both of themarrow_forward
- A 10-kg box is pulled along P,Na rough surface by a force P, as shown in thefigure. The pulling force linearly increaseswith time, while the particle is motionless att = 0s untilit reaches a maximum force of100 Nattimet = 4s. If the ground has staticand kinetic friction coefficients of u, = 0.6 andHU, = 0.4 respectively, determine the velocityof the A 1 0 - kg box is pulled along P , N a rough surface by a force P , as shown in the figure. The pulling force linearly increases with time, while the particle is motionless at t = 0 s untilit reaches a maximum force of 1 0 0 Nattimet = 4 s . If the ground has static and kinetic friction coefficients of u , = 0 . 6 and HU , = 0 . 4 respectively, determine the velocity of the particle att = 4 s .arrow_forwardCalculate the speed of the driven member with the following conditions: Diameter of the motor pulley: 4 in Diameter of the driven pulley: 12 in Speed of the motor pulley: 1800 rpmarrow_forward4. In the figure, shaft A made of AISI 1010 hot-rolled steel, is welded to a fixed support and is subjected to loading by equal and opposite Forces F via shaft B. Stress concentration factors K₁ (1.7) and Kts (1.6) are induced by the 3mm fillet. Notch sensitivities are q₁=0.9 and qts=1. The length of shaft A from the fixed support to the connection at shaft B is 1m. The load F cycles from 0.5 to 2kN and a static load P is 100N. For shaft A, find the factor of safety (for infinite life) using the modified Goodman fatigue failure criterion. 3 mm fillet Shaft A 20 mm 25 mm Shaft B 25 mmarrow_forward
 International Edition---engineering Mechanics: St...Mechanical EngineeringISBN:9781305501607Author:Andrew Pytel And Jaan KiusalaasPublisher:CENGAGE L
International Edition---engineering Mechanics: St...Mechanical EngineeringISBN:9781305501607Author:Andrew Pytel And Jaan KiusalaasPublisher:CENGAGE L
