
Concept explainers
| Year | Cash Flow (A) | Cash Flow (B) |
| 0 | $43,500 | $43,500 |
| 1 | 21,400 | 6,400 |
| 2 | 18,500 | 14,700 |
| 3 | 13,800 | 22,800 |
| 4 | 7,600 | 25,200 |
a. What is the IRR for each of these projects? Using the IRR decision rule, which project should the company accept? Is this decision necessarily correct?
b. If the required return is 11 percent, what is the NPV for each of these projects? Which project will the company choose if it applies the NPV decision rule?
c. Over what range of discount rates would the company choose project A? Project B? At what discount rate would the company be indifferent between these two projects? Explain.
a)
To calculate: The IRR (Internal rate of return) for the proposed projects of Company G, the project that the company must accept and discuss whether the decision made is appropriate.
Introduction:
The IRR (Internal rate of return) is a rate of discount, which makes the predictable investment’s NPV equal to zero.
The NPV (Net present value) is a capital budgeting technique, which is used to assess the investment, and it is utilized to identify the profitability in a proposed investment.
Answer to Problem 12QP
As IRR is higher in Project A when compared to Project B, then Project A can be accepted. However, it is not the appropriate decision because the criterion of IRR has a problem in ranking of mutually exclusive projects.
Explanation of Solution
Given information:
Company G has identified two mutually exclusive projects where the cash flows of Project A are $21,400, $18,500, $13,800, and $7,600 for the years 1, 2, 3, and 4, respectively. The cash flows of Project B are $6,400, $14,700, $22,800, and $25,200 for the years 1, 2, 3, and 4, respectively. The initial costs for both the project are $43,500, respectively.
Note:
- NPV is the difference between the present values of the cash inflows from the present value of cash outflows.
- The IRR is the rate of interest, which makes the project’s NPV equal to zero. Hence, using the available information, assume that the NPV is equal to zero and form an equation to compute the IRR.
Equation of NPV to compute IRR assuming that NPV is equal to zero:
Compute IRR for Project A using a spreadsheet:
Step 1:
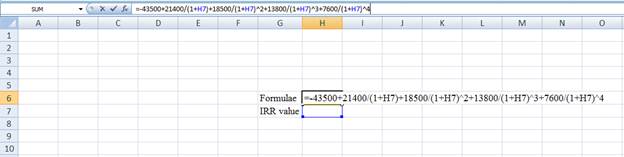
- Type the equation of NPV in H6 in the spreadsheet and consider the IRR value as H7.
Step 2:
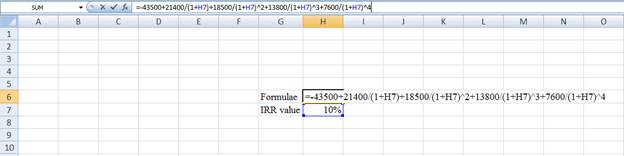
- Assume the IRR value as 0.10%.
Step 3:
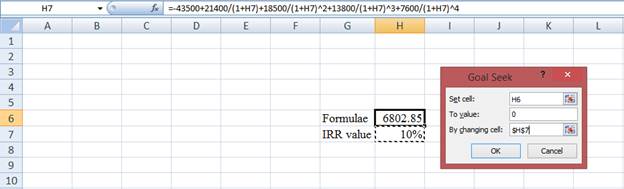
- In the spreadsheet, go to the data and select the what-if analysis.
- In what-if analysis, select goal seek.
- In “Set cell”, select H6 (the formula).
- The “To value” is considered as 0 (the assumption value for NPV).
- The H7 cell is selected for the “By changing cell”.
Step 4:
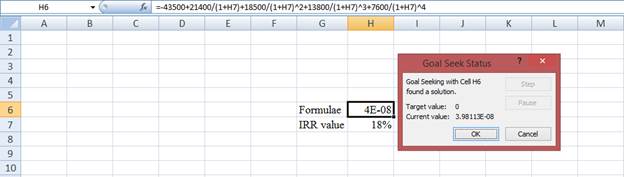
- Following the previous step, click OK in the goal seek. The goal seek status appears with the IRR value.
Step 5:
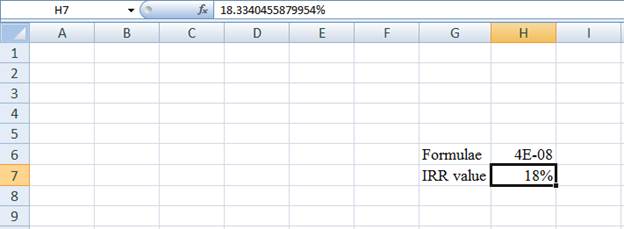
- The value appears to be 18.3340455879954%.
Hence, the IRR value is 18.33%.
Compute IRR for Project B using a spreadsheet:
Step 1:
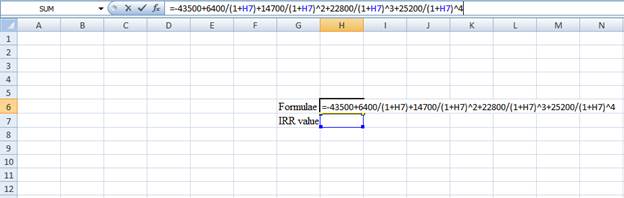
- Type the equation of NPV in H6 in the spreadsheet and consider the IRR value as H7.
Step 2:
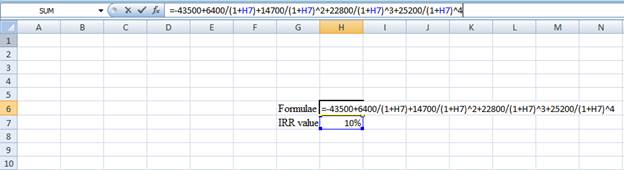
- Assume the IRR value as 0.10%.
Step 3:
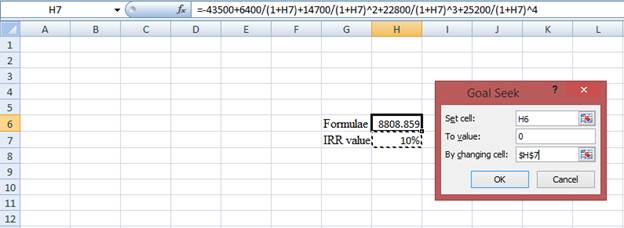
- In the spreadsheet, go to the data and select the what-if analysis.
- In what-if analysis, select goal seek.
- In “Set cell”, select H6 (the formula).
- The “To value” is considered as 0 (the assumption value for NPV).
- The H7 cell is selected for the “By changing cell”.
Step 4:
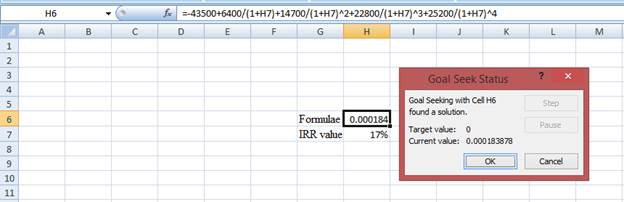
- Following the previous step, click OK in the goal seek. The goal seek status appears with the IRR value.
Step 5:
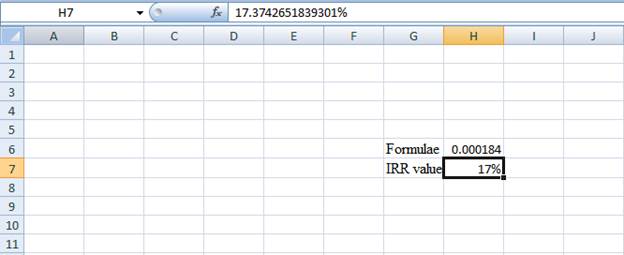
- The value appears to be 17.3742651839301%.
Hence, the IRR value is 17.37%.
b)
To calculate: The NPV for the projects to choose the best project for the company.
Introduction:
The IRR (Internal rate of return) is a rate of discount, which makes the predictable investment’s NPV equal to zero.
The NPV (Net present value) is a capital budgeting technique, which is used to assess the investment, and it is utilized to identify the profitability in a proposed investment.
Answer to Problem 12QP
As NPV is greater in Project B, the company must accept Project B.
Explanation of Solution
Given information:
Company G has identified two mutually exclusive projects where the cash flows of Project A are $21,400, $18,500, $13,800, and $7,600 for the years 1, 2, 3, and 4, respectively. The cash flows of Project B are $6,400, $14,700, $22,800, and $25,200 for the years 1, 2, 3, and 4, respectively. The initial costs for both the project are $43,500, respectively.
Note:
- NPV is the difference between the present values of the cash inflows from the present value of cash outflows.
- The IRR is the rate of interest, which makes the project’s NPV equal to zero. Hence, using the available information, assume that the NPV is equal to zero and form an equation to compute the IRR.
Formula to calculate the NPV:
Compute NPV for Project A:
Hence, the NPV for Project A is $5,891.09.
Compute NPV for Project B:
Note: The rate is given at 11%.
Hence, the NPV for project B is $7,467.80.
c)
To calculate: The rates of discount at which the company will select Project A and Project B, and the discount rate in which the company will be indifferent in selecting between the two projects.
Introduction:
The IRR (Internal rate of return) is a rate of discount, which makes the predictable investment’s NPV equal to zero.
The NPV (Net present value) is a capital budgeting technique, which is used to assess the investment, and it is utilized to identify the profitability in a proposed investment.
Answer to Problem 12QP
At the rate of discount above 15.19%, the company must choose Project A and for the rate below 15.19%, the company should choose Project B. Hence, it is indifferent at the discount rate of 15.19%.
Explanation of Solution
Given information: Company G has identified two mutually exclusive projects where the cash flows of Project A are $21,400, $18,500, $13,800, and $7,600 for the years 1, 2, 3, and 4, respectively. The cash flows of Project B are $6,400, $14,700, $22,800, and $25,200 for the years 1, 2, 3, and 4, respectively. The initial costs for both the project are $43,500, respectively.
Note:
- NPV is the difference between the present values of the cash inflows from the present value of cash outflows.
- The IRR is the rate of interest, which makes the project’s NPV equal to zero. Hence, using the available information, assume that the NPV is equal to zero and form an equation to compute the IRR.
Equation to calculate the crossover rates:
Compute the discount rate using spreadsheet:
Step 1:
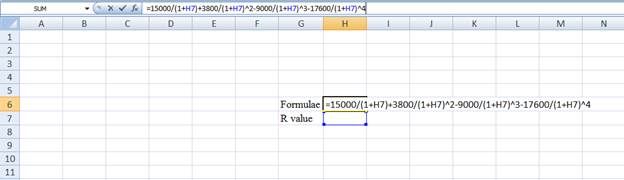
- Type the equation of NPV in H6 in the spreadsheet and consider the R value as H7.
Step 2:
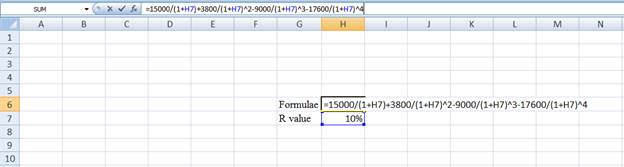
- Assume the R value as 10%.
Step 3:
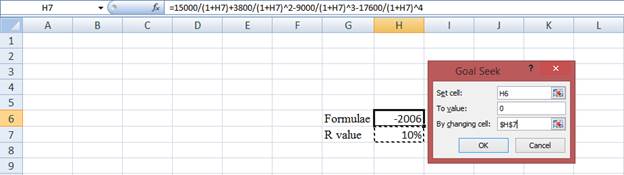
- In the spreadsheet, go to the data and select the what-if analysis.
- In what-if analysis, select goal seek.
- In “Set cell”, select H6 (the formula).
- The “To value” is considered as 0 (the assumption value for NPV).
- The H7 cell is selected for the “By changing cell”.
Step 4:
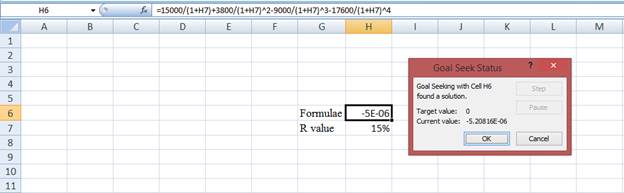
- Following the previous step, click OK in the goal seek. The goal seek status appears with the R value.
Step 5:
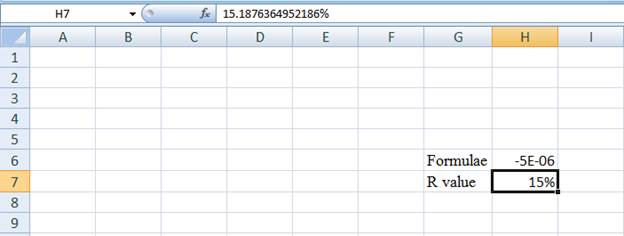
- The value appears to be 15.1876364952186%.
Hence, the R value is 15.19%.
Want to see more full solutions like this?
Chapter 9 Solutions
Fundamentals of Corporate Finance (Special Edition for Rutgers Business School)
 Intermediate Financial Management (MindTap Course...FinanceISBN:9781337395083Author:Eugene F. Brigham, Phillip R. DavesPublisher:Cengage Learning
Intermediate Financial Management (MindTap Course...FinanceISBN:9781337395083Author:Eugene F. Brigham, Phillip R. DavesPublisher:Cengage Learning
