MATHEMATICS A PRACTICAL ODYSSEY W/ACCESS
8th Edition
ISBN: 9780357537343
Author: Johnson
Publisher: CENGAGE L
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 8.1, Problem 24E
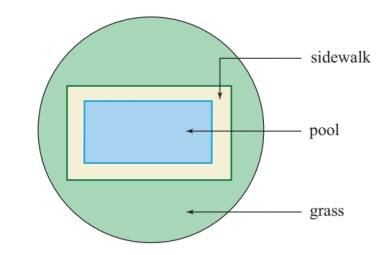
A rectangular swimming pool
a. Find the surface area of the water.
b. Find the area of the concrete sidewalk.
c. Find the area of the grass.
Expert Solution & Answer
Trending nowThis is a popular solution!
Students have asked these similar questions
An airplane flies due west at an airspeed of 428 mph. The wind blows in the direction of 41° south of west
at 50 mph. What is the ground speed of the airplane? What is the bearing of the airplane?
428 mph
41°
50 mph
a. The ground speed of the airplane is
b. The bearing of the airplane is
mph.
south of west.
Rylee's car is stuck in the mud. Roman and Shanice come along in a truck to help pull her out. They attach
one end of a tow strap to the front of the car and the other end to the truck's trailer hitch, and the truck
starts to pull. Meanwhile, Roman and Shanice get behind the car and push. The truck generates a
horizontal force of 377 lb on the car. Roman and Shanice are pushing at a slight upward angle and generate
a force of 119 lb on the car. These forces can be represented by vectors, as shown in the figure below. The
angle between these vectors is 20.2°. Find the resultant force (the vector sum), then give its magnitude
and its direction angle from the positive x-axis.
119 lb
20.2°
377 lb
a. The resultant force is
(Tip: omit degree notations from your answers; e.g. enter cos(45) instead of cos(45°))
b. It's magnitude is
lb.
c. It's angle from the positive x-axis is
Complete the table below. For solutions, round to the nearest whole
number.
Chapter 8 Solutions
MATHEMATICS A PRACTICAL ODYSSEY W/ACCESS
Ch. 8.1 - When necessary, round off answers to one decimal...Ch. 8.1 - When necessary, round off answers to one decimal...Ch. 8.1 - When necessary, round off answers to one decimal...Ch. 8.1 - Prob. 4ECh. 8.1 - When necessary, round off answers to one decimal...Ch. 8.1 - When necessary, round off answers to one decimal...Ch. 8.1 - When necessary, round off answers to one decimal...Ch. 8.1 - When necessary, round off answers to one decimal...Ch. 8.1 - In Exercise 9 and 10, find a the area and b the...Ch. 8.1 - Prob. 10E
Ch. 8.1 - Prob. 11ECh. 8.1 - In Exercises 1120, find (a) the area and (b) the...Ch. 8.1 - In Exercises 1120, find (a) the area and (b) the...Ch. 8.1 - In Exercises 1120 find(a) the area and (b) the...Ch. 8.1 - In Exercises 1120, find (a) the area and (b) the...Ch. 8.1 - In Exercises 1120, find (a) the area and (b) the...Ch. 8.1 - Prob. 17ECh. 8.1 - In Exercises 1120 find a the area and b the...Ch. 8.1 - In Exercises 1120 find a the area and b the...Ch. 8.1 - In Exercises 1120 find a the area and b the...Ch. 8.1 - In Exercises 21 and 22, find (a) the area and (b)...Ch. 8.1 - Prob. 22ECh. 8.1 - A circular swimming pool has diameter 50ft and is...Ch. 8.1 - A rectangular swimming pool 30feet by 15feet is...Ch. 8.1 - Prob. 25ECh. 8.1 - Prob. 26ECh. 8.1 - Prob. 27ECh. 8.1 - Prob. 28ECh. 8.1 - You jog 34 mile due north, then 112 miles due...Ch. 8.1 - You walk 100 yards due south, then 120 yards due...Ch. 8.1 - A 10-foot ladder leans against a wall. If the base...Ch. 8.1 - Prob. 32ECh. 8.1 - Prob. 33ECh. 8.1 - Prob. 34ECh. 8.1 - The length of the edges of a triangular canvas are...Ch. 8.1 - Jim Mulios wants to fertilize his backyard. The...Ch. 8.1 - Reddies Pizza Bash is famous for its Loads-o-Meat...Ch. 8.1 - Prob. 38ECh. 8.1 - Prob. 39ECh. 8.1 - Prob. 40ECh. 8.1 - Prob. 41ECh. 8.1 - In Exercise3944, use the following information: in...Ch. 8.1 - Prob. 43ECh. 8.1 - Prob. 44ECh. 8.1 - Prob. 45ECh. 8.1 - Prob. 46ECh. 8.1 - Prob. 47ECh. 8.1 - Prob. 48ECh. 8.1 - Prob. 49ECh. 8.1 - Prob. 50ECh. 8.1 - Prob. 51ECh. 8.1 - Prob. 52ECh. 8.1 - Prob. 53ECh. 8.1 - Prob. 54ECh. 8.1 - Prob. 55ECh. 8.1 - Prob. 56ECh. 8.1 - Prob. 57ECh. 8.1 - Prob. 58ECh. 8.1 - Prob. 59ECh. 8.2 - When necessary, round off answers to two decimal...Ch. 8.2 - When necessary, round off answers to two decimal...Ch. 8.2 - When necessary, round off answers to two decimal...Ch. 8.2 - When necessary, round off answers to two decimal...Ch. 8.2 - Prob. 5ECh. 8.2 - When necessary, round off answers to two decimal...Ch. 8.2 - Prob. 7ECh. 8.2 - In Exercises714, find the volume of each figure....Ch. 8.2 - In Exercises714, find the volume of each figure....Ch. 8.2 - In Exercises714, find the volume of each figure....Ch. 8.2 - In Exercises714, find the volume of each figure....Ch. 8.2 - In Exercises714, find the volume of each figure....Ch. 8.2 - Prob. 13ECh. 8.2 - Prob. 14ECh. 8.2 - From a 10-inch-by-16-inch piece of cardboard,...Ch. 8.2 - From a 24-inch-square piece of cardboard, square...Ch. 8.2 - A grain silo consists of a cylinder with a...Ch. 8.2 - A propane gas tank consists of a cylinder with a...Ch. 8.2 - A regulation baseball hardball has a circumference...Ch. 8.2 - A regulation tennis ball has a diameter of 212...Ch. 8.2 - The diameter of the earth is approximately 7, 920...Ch. 8.2 - The diameter of the earth is approximately 7, 920...Ch. 8.2 - Prob. 23ECh. 8.2 - Prob. 24ECh. 8.2 - In Exercises 25 and 26, use the following...Ch. 8.2 - Prob. 26ECh. 8.2 - Prob. 27ECh. 8.2 - Golf balls are packaged three to a rectangular...Ch. 8.2 - Prob. 29ECh. 8.2 - Marcus Robinson bought an older house and wants to...Ch. 8.2 - Prob. 31ECh. 8.2 - The student union at the University of Utopia U2...Ch. 8.2 - A water storage tank is an upside-down cone, as...Ch. 8.2 - The diameter of a conical paper cup is 3.5inches....Ch. 8.2 - Prob. 35ECh. 8.2 - Prob. 36ECh. 8.2 - Prob. 37ECh. 8.2 - Prob. 38ECh. 8.2 - Prob. 39ECh. 8.2 - Prob. 40ECh. 8.2 - Prob. 41ECh. 8.2 - Prob. 42ECh. 8.2 - Prob. 43ECh. 8.3 - In Exercises 1-4. determine whether each...Ch. 8.3 - Prob. 2ECh. 8.3 - Prob. 3ECh. 8.3 - Prob. 4ECh. 8.3 - Prob. 5ECh. 8.3 - Prob. 6ECh. 8.3 - Prob. 7ECh. 8.3 - Prob. 8ECh. 8.3 - Following the method of Problem 48 of the Rhind...Ch. 8.3 - Prob. 10ECh. 8.3 - Prob. 11ECh. 8.3 - Prob. 12ECh. 8.3 - Prob. 13ECh. 8.3 - Prob. 14ECh. 8.3 - Prob. 15ECh. 8.3 - Prob. 16ECh. 8.3 - Find the perimeter of a triangle having sides with...Ch. 8.3 - Prob. 18ECh. 8.3 - Prob. 19ECh. 8.3 - Prob. 20ECh. 8.3 - Prob. 21ECh. 8.3 - Prob. 22ECh. 8.3 - Prob. 23ECh. 8.3 - Prob. 24ECh. 8.3 - Problem 41 of the Rhind Papyrus pertains to...Ch. 8.3 - Prob. 26ECh. 8.3 - Prob. 27ECh. 8.3 - Prob. 28ECh. 8.3 - Prob. 29ECh. 8.3 - Prob. 30ECh. 8.4 - In Exercises 1-4, the given triangles are similar....Ch. 8.4 - In Exercises 1-4, the given triangles are similar....Ch. 8.4 - In Exercises 1-4, the given triangles are similar....Ch. 8.4 - In Exercises 1-4, the given triangles are similar....Ch. 8.4 - A 6-foot-tall man casts a shadow of 3.5 feet at...Ch. 8.4 - Prob. 6ECh. 8.4 - Prob. 7ECh. 8.4 - Use Figure 8.67 and the method of rearrangement to...Ch. 8.4 - Find the length of the longest object that will...Ch. 8.4 - Find the length of the longest object that will...Ch. 8.4 - Find the length of the longest object that will...Ch. 8.4 - Find the length of the longest object that will...Ch. 8.4 - Prob. 13ECh. 8.4 - Prob. 14ECh. 8.4 - In Exercises 1318, use the two column method to...Ch. 8.4 - Prob. 16ECh. 8.4 - Prob. 17ECh. 8.4 - Prob. 18ECh. 8.4 - Prob. 19ECh. 8.4 - Prob. 20ECh. 8.4 - Prob. 21ECh. 8.4 - Prob. 22ECh. 8.4 - If a sphere is inscribed in a cylinder, the...Ch. 8.4 - Prob. 24ECh. 8.4 - Prob. 25ECh. 8.4 - Prob. 26ECh. 8.4 - Prob. 27ECh. 8.4 - Prob. 28ECh. 8.4 - Prob. 29ECh. 8.4 - The purpose of this project is to calculate the...Ch. 8.4 - Prob. 31ECh. 8.4 - Prob. 32ECh. 8.5 - In Exercises 18, use trigonometric ratios to find...Ch. 8.5 - In Exercises 18, use trigonometric ratios to find...Ch. 8.5 - In Exercises 18, use trigonometric ratios to find...Ch. 8.5 - In Exercises 18, use trigonometric ratios to find...Ch. 8.5 - In Exercises 18, use trigonometric ratios to find...Ch. 8.5 - In Exercises 18, use trigonometric ratios to find...Ch. 8.5 - In Exercises 18, use trigonometric ratios to find...Ch. 8.5 - Prob. 8ECh. 8.5 - In Exercises 926. use the given information to...Ch. 8.5 - Prob. 10ECh. 8.5 - Prob. 11ECh. 8.5 - Prob. 12ECh. 8.5 - Prob. 13ECh. 8.5 - Prob. 14ECh. 8.5 - Prob. 15ECh. 8.5 - Prob. 16ECh. 8.5 - Prob. 17ECh. 8.5 - Prob. 18ECh. 8.5 - Prob. 19ECh. 8.5 - Prob. 20ECh. 8.5 - Prob. 21ECh. 8.5 - Prob. 22ECh. 8.5 - Prob. 23ECh. 8.5 - Prob. 24ECh. 8.5 - Prob. 25ECh. 8.5 - Prob. 26ECh. 8.5 - A cable runs from the top of a building to a point...Ch. 8.5 - A support cable runs from the top of a telephone...Ch. 8.5 - Prob. 29ECh. 8.5 - Prob. 30ECh. 8.5 - Prob. 31ECh. 8.5 - Prob. 32ECh. 8.5 - Prob. 33ECh. 8.5 - Prob. 34ECh. 8.5 - A billboard is on top of a building. You are 125.0...Ch. 8.5 - Prob. 36ECh. 8.5 - You are hiking along a river and see a tall tree...Ch. 8.5 - Prob. 38ECh. 8.5 - Prob. 39ECh. 8.5 - Prob. 40ECh. 8.5 - Prob. 41ECh. 8.5 - Prob. 42ECh. 8.5 - Prob. 43ECh. 8.5 - Two observers are 500feet apart. Each measures the...Ch. 8.5 - Prob. 45ECh. 8.5 - Prob. 46ECh. 8.5 - Prob. 47ECh. 8.5 - Prob. 48ECh. 8.5 - Prob. 49ECh. 8.5 - Prob. 50ECh. 8.5 - Prob. 51ECh. 8.5 - Prob. 52ECh. 8.5 - Prob. 53ECh. 8.6 - Prob. 1ECh. 8.6 - Prob. 2ECh. 8.6 - Prob. 3ECh. 8.6 - In Exercise 1-4, answer the following questions....Ch. 8.6 - Prob. 5ECh. 8.6 - Prob. 6ECh. 8.6 - Prob. 7ECh. 8.6 - Prob. 8ECh. 8.6 - Prob. 9ECh. 8.6 - Prob. 10ECh. 8.6 - In Exercises 11-20, answer the following...Ch. 8.6 - Prob. 12ECh. 8.6 - Prob. 13ECh. 8.6 - Prob. 14ECh. 8.6 - Prob. 15ECh. 8.6 - Prob. 16ECh. 8.6 - Prob. 17ECh. 8.6 - Prob. 18ECh. 8.6 - Prob. 19ECh. 8.6 - In Exercises 11-20, answer the following...Ch. 8.6 - Prob. 21ECh. 8.6 - Prob. 22ECh. 8.6 - Prob. 23ECh. 8.6 - Prob. 24ECh. 8.6 - Prob. 25ECh. 8.7 - Prob. 1ECh. 8.7 - Prob. 2ECh. 8.7 - Prob. 3ECh. 8.7 - Prob. 4ECh. 8.7 - Prob. 5ECh. 8.7 - Prob. 6ECh. 8.7 - Prob. 7ECh. 8.7 - Prob. 8ECh. 8.7 - Prob. 9ECh. 8.7 - Prob. 10ECh. 8.7 - Prob. 11ECh. 8.7 - Prob. 12ECh. 8.7 - Prob. 13ECh. 8.7 - A satellite dish antenna has a parabolic reflector...Ch. 8.7 - Prob. 15ECh. 8.7 - Prob. 16ECh. 8.7 - Prob. 17ECh. 8.7 - In Exercises 17-24, sketch the graph and find the...Ch. 8.7 - Prob. 19ECh. 8.7 - Prob. 20ECh. 8.7 - Prob. 21ECh. 8.7 - Prob. 22ECh. 8.7 - Prob. 23ECh. 8.7 - Prob. 24ECh. 8.7 - Prob. 25ECh. 8.7 - Prob. 26ECh. 8.7 - Prob. 27ECh. 8.7 - Prob. 28ECh. 8.7 - Prob. 29ECh. 8.7 - In Exercises 29-36, sketch the graph and find the...Ch. 8.7 - Prob. 31ECh. 8.7 - Prob. 32ECh. 8.7 - Prob. 33ECh. 8.7 - Prob. 34ECh. 8.7 - Prob. 35ECh. 8.7 - Prob. 36ECh. 8.7 - Prob. 37ECh. 8.7 - Prob. 38ECh. 8.8 - Prob. 1ECh. 8.8 - Prob. 2ECh. 8.8 - Prob. 3ECh. 8.8 - Prob. 4ECh. 8.8 - Prob. 5ECh. 8.8 - Prob. 6ECh. 8.8 - Prob. 7ECh. 8.8 - Prob. 8ECh. 8.8 - In Exercises 7-12, answer the questions for the...Ch. 8.8 - Prob. 10ECh. 8.8 - Prob. 11ECh. 8.8 - Prob. 12ECh. 8.8 - Prob. 13ECh. 8.8 - Prob. 14ECh. 8.8 - Prob. 15ECh. 8.8 - Prob. 16ECh. 8.8 - Prob. 17ECh. 8.8 - Prob. 18ECh. 8.8 - Prob. 19ECh. 8.8 - What was the scandal of elementary geometry?Ch. 8.8 - Prob. 21ECh. 8.8 - Who was the first mathematician to affirm the...Ch. 8.8 - Prob. 23ECh. 8.8 - Prob. 24ECh. 8.8 - Prob. 25ECh. 8.8 - Prob. 26ECh. 8.8 - Prob. 27ECh. 8.9 - HANDS ON The Sierpinski gasket Using a...Ch. 8.9 - The Sierpinski carpet. The Sierpinski carpet is...Ch. 8.9 - The Mitsubishi gasket. Carefully and accurately...Ch. 8.9 - The square snowflake. Carefully and accurately...Ch. 8.9 - Prob. 5ECh. 8.9 - Prob. 6ECh. 8.9 - Prob. 7ECh. 8.9 - Prob. 8ECh. 8.9 - Prob. 9ECh. 8.9 - Find the approximate dimension of the Sierpinski...Ch. 8.9 - Find the approximate dimension of the Mitsubishi...Ch. 8.9 - Prob. 12ECh. 8.9 - Find the approximate dimension of the square...Ch. 8.9 - Prob. 14ECh. 8.9 - Prob. 15ECh. 8.9 - Prob. 16ECh. 8.9 - Prob. 17ECh. 8.9 - Prob. 18ECh. 8.9 - Prob. 19ECh. 8.9 - Prob. 20ECh. 8.9 - Prob. 21ECh. 8.9 - 22. Describe the self-similarity of the given...Ch. 8.9 - Prob. 23ECh. 8.9 - Prob. 24ECh. 8.9 - Prob. 25ECh. 8.9 - Name three naturally occurring objects other than...Ch. 8.9 - Prob. 27ECh. 8.9 - Prob. 28ECh. 8.9 - Prob. 29ECh. 8.9 - Prob. 30ECh. 8.9 - Prob. 31ECh. 8.9 - Prob. 32ECh. 8.9 - Prob. 33ECh. 8.9 - Prob. 35ECh. 8.9 - Prob. 36ECh. 8.10 - Prob. 1ECh. 8.10 - Prob. 2ECh. 8.10 - Finding the perimeter of a Sierpinski carpet. See...Ch. 8.10 - Finding the area of a Sierpinski carpet. See...Ch. 8.10 - Prob. 5ECh. 8.10 - A square snowflake. See Exercise 4 in Section 8.9...Ch. 8.10 - Prob. 7ECh. 8.10 - Prob. 8ECh. 8.10 - Describe an application of fractal area,...Ch. 8.10 - Design your own fractal. Discuss its...Ch. 8.CR - Prob. 1CRCh. 8.CR - Prob. 2CRCh. 8.CR - Prob. 3CRCh. 8.CR - Prob. 4CRCh. 8.CR - Prob. 5CRCh. 8.CR - Saturn the ringed planet revolves around the sun...Ch. 8.CR - Prob. 7CRCh. 8.CR - Prob. 8CRCh. 8.CR - Prob. 9CRCh. 8.CR - Prob. 10CRCh. 8.CR - Find the volume and surface area of Figure 8.194.Ch. 8.CR - Prob. 12CRCh. 8.CR - Prob. 13CRCh. 8.CR - An oval athletic field is the combination of a...Ch. 8.CR - From a 12inch-by-18inch piece of cardboard,...Ch. 8.CR - A regulation baseball hardball has a circumference...Ch. 8.CR - Prob. 17CRCh. 8.CR - Prob. 18CRCh. 8.CR - Prob. 19CRCh. 8.CR - Prob. 20CRCh. 8.CR - Prob. 21CRCh. 8.CR - Prob. 22CRCh. 8.CR - Prob. 23CRCh. 8.CR - Find the length of the longest object that will...Ch. 8.CR - Prob. 25CRCh. 8.CR - Prob. 26CRCh. 8.CR - In Exercise 26 and 27, use the two-column method...Ch. 8.CR - Prob. 28CRCh. 8.CR - A regular dodecagon 12-sided polygon is inscribed...Ch. 8.CR - Prob. 30CRCh. 8.CR - Prob. 31CRCh. 8.CR - Prob. 32CRCh. 8.CR - Prob. 33CRCh. 8.CR - Prob. 34CRCh. 8.CR - Prob. 35CRCh. 8.CR - Prob. 36CRCh. 8.CR - Prob. 37CRCh. 8.CR - Prob. 38CRCh. 8.CR - Prob. 39CRCh. 8.CR - Prob. 40CRCh. 8.CR - Prob. 41CRCh. 8.CR - Prob. 42CRCh. 8.CR - Prob. 43CRCh. 8.CR - Prob. 44CRCh. 8.CR - The box fractal. To create this shape, use the...Ch. 8.CR - Prob. 46CRCh. 8.CR - Prob. 47CRCh. 8.CR - Prob. 48CRCh. 8.CR - Draw a building in two-point perspective. viewed...Ch. 8.CR - Prob. 50CRCh. 8.CR - Prob. 51CRCh. 8.CR - Prob. 52CRCh. 8.CR - Prob. 53CR
Additional Math Textbook Solutions
Find more solutions based on key concepts
The largest polynomial that divides evenly into a list of polynomials is called the _______.
Elementary & Intermediate Algebra
1. How much money is Joe earning when he’s 30?
Pathways To Math Literacy (looseleaf)
Is there a relationship between wine consumption and deaths from heart disease? The table gives data from 19 de...
College Algebra Essentials (5th Edition)
NOTE: Write your answers using interval notation when appropriate.
CHECKING ANALYTIC SKILLS Fill in each blank ...
Graphical Approach To College Algebra
For Problems 23-28, write in simpler form, as in Example 4. logbFG
Finite Mathematics for Business, Economics, Life Sciences and Social Sciences
1. How is a sample related to a population?
Elementary Statistics: Picturing the World (7th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- A biologist is investigating the effect of potential plant hormones by treating 20 stem segments. At the end of the observation period he computes the following length averages: Compound X = 1.18 Compound Y = 1.17 Based on these mean values he concludes that there are no treatment differences. 1) Are you satisfied with his conclusion? Why or why not? 2) If he asked you for help in analyzing these data, what statistical method would you suggest that he use to come to a meaningful conclusion about his data and why? 3) Are there any other questions you would ask him regarding his experiment, data collection, and analysis methods?arrow_forwardBusinessarrow_forwardAnswer first questionarrow_forward
- Let the universal set be whole numbers 1 through 20 inclusive. That is, U = {1, 2, 3, 4, . . ., 19, 20}. Let A, B, and C be subsets of U. Let A be the set of all prime numbers: A = {2, 3, 5, 7, 11, 13, 17, 19} Let B be the set of all odd numbers: B = {1,3,5,7, . . ., 17, 19} Let C be the set of all square numbers: C = {1,4,9,16}arrow_forwardA research team consists of 4 senior researchers and 10 research assistants. The team needs to select 2 senior researchers and 2 research assistants to attend a conference. How many different ways can the group being sent to the conference be formed?arrow_forwardThere are 25 different varieties of flowering plants found in a natural habitat you are studying. You are asked to randomly select 5 of these flowering plant varieties to bring back to your laboratory for further study. How many different combinations of are possible? That is, how many possible 5 plant subgroups can be formed out of the 25 total plants found?arrow_forward
- A person is tossing a fair, two-sided coin three times and recording the results (either a Heads, H, or a Tails, T). Let E be the event that exactly two heads are tossed. Which of the following sets represent the event E? Group of answer choices {HHT, HTH, THH} {HHT, THH} {HHH, HHT, HTH, THH, TTT, TTH, THT, HTT} {HH}arrow_forwardTake Quiz 54m Exit Let the universal set be whole numbers 1 through 20 inclusive. That is, U = {1, 2, 3, 4, . . ., 19, 20}. Let A, B, and C be subsets of U. Let A be the set of all prime numbers: A = {2, 3, 5, 7, 11, 13, 17, 19} Let B be the set of all odd numbers: B = {1,3,5,7, • • , 17, 19} Let C be the set of all square numbers: C = {1,4,9,16} ☐ Question 2 3 pts Which of the following statement(s) is true? Select all that apply. (1) АСВ (2) A and C are disjoint (mutually exclusive) sets. (3) |B| = n(B) = 10 (4) All of the elements in AC are even numbers. ☐ Statement 1 is true. Statement 2 is true. Statement 3 is true. Statement 4 is true.arrow_forward☐ Question 1 2 pts Let G be the set that represents all whole numbers between 5 and 12 exclusive. Which of the following is set G in standard set notation. (Roster Method)? O G = [5, 12] G = {5, 6, 7, 8, 9, 10, 11, 12} O G = (5, 12) OG = {6, 7, 8, 9, 10, 11}arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Holt Mcdougal Larson Pre-algebra: Student Edition...
Algebra
ISBN:9780547587776
Author:HOLT MCDOUGAL
Publisher:HOLT MCDOUGAL
Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning
Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
Elementary Geometry For College Students, 7e
Geometry
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Cengage,
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,
Problems on Area and Circumference of Circle| Basics of Circle| Questions on Circle||BrainPanthers; Author: Brain Panthers;https://www.youtube.com/watch?v=RcNEL9OzcC0;License: Standard YouTube License, CC-BY