
(a)
To find: Use the graph to get the domain, range and zeros
(a)
Answer to Problem 4CT
Explanation of Solution
Given information: The graph of the function with some coordinates
Concept used:
The domain is the set of x values for which the function is defined and the value is real.
Range is the set of all y values for which the function is defined.
Calculation:
In the graph there is no restriction for x . The curve is for all value of x. So, domain is set of all real numbers
The graph starts at 8 on y and it goes down
x intercepts are the values where the graph crosses x- axis
(b)
To find: Use the graph to get the standard form of the function
(b)
Answer to Problem 4CT
Explanation of Solution
Given information: The graph of the function with some coordinates
Formula used:
Calculation:
Substitute the given vertex in general form
To find the value of ‘ a’ use the ‘x’ intercept
Putting value of a back in the equation we get,
Conclusion: The equation of the graph is
(c )
To compare: The graph of f(x) with the function
(c )
Answer to Problem 4CT
f(x) is upside down, f(x) is vertically stretched by 2 units, f(x) shifted up by 8 units and right by 5 units
Explanation of Solution
Given information: The equation obtained from part (b)
Calculation:
Compare both equations f(x) and
For -2 , the graph is stretched vertically and parabola is upside down
5 is subtracted from x , the graph of f(x) is shifted 5 units right
8 is subtracted from x , the graph of f(x) is shifted 8 units up
(d)
To graph: Graph the function
(d)
Answer to Problem 4CT
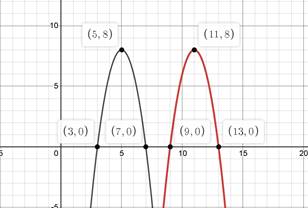
Explanation of Solution
Given information:
Calculation:
6 is subtracted from x in f(x). So the graph of h(x) will be shifted 6 units to the right
Move all the points on the graph of f(x) , 6 units to the right
Chapter 8 Solutions
BIG IDEAS MATH Algebra 1: Common Core Student Edition 2015
- Solve questionsarrow_forwardPatterns in Floor Tiling A square floor is to be tiled with square tiles as shown. There are blue tiles on the main diagonals and red tiles everywhere else. In all cases, both blue and red tiles must be used. and the two diagonals must have a common blue tile at the center of the floor. If 81 blue tiles will be used, how many red tiles will be needed?arrow_forwardFind the values of n, if the points (n + 1, 2n), (3n, 2n + 3) and (5n + 1,5n) are collinear. Find the value of k that the four points (4,1,2), (5, k, 6), (5,1,-1) and (7,4,0) are coplanar. Find the value of r if the area of the triangle is formed by the points (-3,6),(4,4) and (r,-2) is 12 sq units. Find the volume of tetrahedron whose vertices are A(1,1,0), B(-4,3,6), C(-1,0,3) and D(2,4,-5).arrow_forward
- - Consider the following system of linear equations in the variables a,b,c,d: 5a-3b 7c - 2d = 2 2ab 2c+ 5d = -3 → (*) 4a 3b 5d = 3 6a b+2c+ 7d = −7 (a) Solve the system (*) by using Gauss elimination method. (b) Solve the system (*) by using Cramer's rule method.arrow_forwardSolve for a 25 55 30 a=?arrow_forward9:41 … 93 Applying an Exponential Function to Newton's Law of Cooling 60. Water in a water heater is originally Aa ← 122°F. The water heater is shut off and the water cools to the temperature of the surrounding air, which is 60°F. The water cools slowly because of the insulation inside the heater, and the value of k is measured as 0.00351. a. Write a function that models the temperature T (t) (in °F) of the water t hours after the water heater is shut off. b. What is the temperature of the water 12 hr after the heater is shut off? Round to the nearest degree. c. Dominic does not like to shower with water less than 115°F. If Dominic waits 24 hr. will the water still be warm enough for a shower? Mixed Exercises ger-ui.prod.mheducation.comarrow_forward
- Please use the infinite series formula and specify how you did each step. Thank you.arrow_forward8) Solve the given system using the Gaussian Elimination process. 2x8y = 3 (-6x+24y = −6arrow_forward7) Solve the given system using the Gaussian Elimination process. (5x-4y = 34 (2x - 2y = 14arrow_forward
- 33 (a) (b) Let A(t) = = et 0 0 0 cos(t) sin(t) 0-sin(t) cos(t)) For any fixed tЄR, find det(A(t)). Show that the matrix A(t) is invertible for any tЄ R, and find the inverse (A(t))¹.arrow_forwardUse the infinite geometric sum to convert .258 (the 58 is recurring, so there is a bar over it) to a ratio of two integers. Please go over the full problem, specifying how you found r. Thank you.arrow_forwardH.w: Find the Eigen vectors for the largest Eigen value of the system X1+ +2x3=0 3x1-2x2+x3=0 4x1+ +3x3=0arrow_forward
 Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON
Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning
Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON
Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press
Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education
College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education





