Chapter 8, Problem 48P

Determining the velocity of particles settling through fluids is of great importance of many areas of engineering and science. Such calculations depend on the flow regime as represented by the dimensionless Reynolds number,

$\mathrm{Re}=\frac{\rho dv}{\mu }$ (P8.48.1)

where $\rho =$ the fluid's density $\left({\text{kg/m}}^{\text{3}}\right),d=$ the particle diameter $\left(\text{m}\right),y=$ the particle's settling velocity $\left(\text{m/s}\right),\text{ and }\mu =$ the fluid's dynamic viscosity $\left({\text{N s/m}}^{\text{2}}\right)$. Under laminar conditions $\left(\text{Re}<0.1\right)$, the settling velocity of a spherical particle can be computed with the following formula based on Stokes law,

$v=\frac{g}{18}\left(\frac{{\rho }_s-\rho }{\mu }\right)d^2$ (P8.48.2)

where $g=$ the gravitational constant $\left(=9.81{\text{ m/s}}^{\text{2}}\right),\text{ and }{\rho }_s=$ the particle's density $\left({\text{kg/m}}^{\text{3}}\right)$. For turbulent conditions (i.e., higher Reynolds numbers), an alternative approach can be used based on the following formula:

$v=\sqrt{\frac{4g\left({\rho }_s-\rho \right)d}{3C_D\rho }}$ (P8.48.3)

where $C_D=$ the drag coefficient, which depends on the Reynolds number as in

$C_D=\frac{24}{\mathrm{Re}}+\frac{3}{\sqrt{\mathrm{Re}}}+0.34$ (P8.48.4)

(a) Combine Eqs. (P8.48.2), (P8.48.3), and (P8.48.4) to express the determination of v as a roots of equations problem. That is, express the combined formula in the format $f\left(v\right)=0$.

(b) Use the modified secant method with $\delta ={10}^{23}\text{ and }{\epsilon }_S=0.05\%$ to determine v for a spherical iron particle settling in water, where $d=200\mu \text{m},\rho =1{\text{g/cm}}^{\text{3}}$, ${\rho }_s=7.874{\text{g/cm}}^{\text{3}},\text{and }\mu =0.014\text{ g/}\left(\text{cm}\cdot \text{s}\right)$. Employ Eq. (P8.48.2) to generate your initial guess.

(c) Based on the result of (b), compute the Reynolds number and the drag coefficient, and use the latter to confirm that the flow regime is not laminar.

(d) Develop a fixed-point iteration solution for the conditions outlined in (b).

(e) Use a graphical approach to illustrate that the formulation developed in (d) will converge for any positive guess.

To determine

To calculate: The equation for the velocity of the particles that settle inside fluids in the form $f\left(v\right)=0$.

Answer to Problem 48P

Solution: The desired equation for the velocity of the settling particles is $f\left(v\right)=\sqrt{\frac{4g\left({\rho }_s-\rho \right)d}{3\left(\frac{24\mu }{\rho dv}+\frac{3\sqrt{\mu }}{\sqrt{\rho dv}}+0.34\right)\rho }}-v$.

Explanation of Solution

Given Information:

The relation between the fluid’s velocity in $\text{m}/\text{s}$, v and the dimensionless Reynolds number, Re, is:

$\mathrm{Re}=\frac{\rho dv}{\mu }$

Here, d is the diameter of the object in m, $\rho$ is the density of the object in $\text{kg}/{\text{m}}^3$ and $\mu$ is the fluids viscosity in $\text{Ns}/{\text{m}}^2$.

Formula Used:

When the Reynolds number is less than 0.1, the Stokes law states that:

$v=\frac{g}{18}\left(\frac{{\rho }_s-\rho }{\mu }\right)d^2$

Here, g is the gravitational constant and ${\rho }_s$ is the particle’s density in $\text{kg}/{\text{m}}^3$.

When the Reynolds number is higher,

$v=\sqrt{\frac{4g\left({\rho }_s-\rho \right)d}{3C_D\rho }}$

Here $C_D$ is the drag coefficient whose value is given by:

$C_D=\frac{24}{\mathrm{Re}}+\frac{3}{\sqrt{\mathrm{Re}}}+0.34$

Calculation:

Consider the formula for the Reynolds’ number:

$\mathrm{Re}=\frac{\rho dv}{\mu }$

Substitute the value of the Reynolds number in the formula for the drag coefficient.

$C_D=\frac{24\mu }{\rho dv}+\frac{3\sqrt{\mu }}{\sqrt{\rho dv}}+0.34$ ……$\left(1\right)$

Now replace the value of the drag coefficient from equation $\left(1\right)$ into the formula for the velocity of the particle when the Reynolds number is greater than 0.1 to obtain:

$\begin{array}{c}v=\sqrt{\frac{4g\left({\rho }_s-\rho \right)d}{3C_D\rho }}\\ v=\sqrt{\frac{4g\left({\rho }_s-\rho \right)d}{3\left(\frac{24\mu }{\rho dv}+\frac{3\sqrt{\mu }}{\sqrt{\rho dv}}+0.34\right)\rho }}\end{array}$

Now create a function whose zero is same as the root of this equation:

$f\left(v\right)=\sqrt{\frac{4g\left({\rho }_s-\rho \right)d}{3\left(\frac{24\mu }{\rho dv}+\frac{3\sqrt{\mu }}{\sqrt{\rho dv}}+0.34\right)\rho }}-v$

This is the desired equation.

To determine

To calculate: The zero of the function obtained in part (a) using the modified secant method where $\delta$ equals ${10}^{-3}$ and ${\epsilon }_s$ is 0.05% and obtain the velocity of an iron particle settling in water.

Answer to Problem 48P

Solution: The velocity of the iron particle settling in water is $0.0697075\text{ m}/\text{s}$.

Explanation of Solution

Given Information:

The diameter of the iron particle is $200\mu m$, the density of the iron particle ${\rho }_s$ is $7.874\text{ g}/{\text{cm}}^3$, the viscosity of water $\mu$ is $0.014\text{g}/\text{cm}\cdot \text{s}$.

Formula Used:

The iterative scheme for the modified secant method is of the form:

$x_{i+1}=x_i-\frac{f\left(x_i\right)}{f\text{'}\left(x_i\right)}$

where $f\text{'}\left(x_i\right)$ can be approximated by:

$f\text{'}\left(x_i\right)=\frac{f\left(x_i+\delta \right)-f\left(x_i\right)}{\delta }$

Calculation:

Note that the dimensions of the diameter, particle density, water density, and the viscosity of water are different to what can be used in the equation obtained in part (a).

Convert the dimensions as follows:

$\begin{array}{c}d=200\mu \text{m}\\ =200\mu \text{m}\cdot \frac{\text{m}}{{10}^6\mu \text{m}}\\ =0.0002\text{m}\end{array}$

And,

$\begin{array}{c}\rho =1\text{g}/{\text{cm}}^3\\ =1\text{g}/{\text{cm}}^3\cdot \frac{{10}^6{\text{cm}}^3}{{\text{m}}^3}\cdot \frac{\text{kg}}{{10}^3\text{g}}\\ =1000\text{ kg}/{\text{m}}^3\end{array}$

And,

$\begin{array}{c}\mu =0.014\frac{g}{\text{cm s}}\\ =0.014\frac{g}{\text{cms}}\cdot \frac{100\text{cm}}{\text{m}}\cdot \frac{\text{kg}}{{\text{10}}^3\text{g}}\\ =0.0014\frac{\text{kg}}{\text{m s}}\end{array}$

And,

$\begin{array}{c}{\rho }_s=7.874\text{g}/{\text{cm}}^3\\ =7.874\text{g}/{\text{cm}}^3\cdot \frac{{10}^6{\text{cm}}^3}{{\text{m}}^3}\cdot \frac{\text{kg}}{{10}^3\text{g}}\\ =7874\text{ kg}/{\text{m}}^3\end{array}$

The modified secant method requires an initial guess. This can be obtained using Stoke’s law.

$\begin{array}{c}v=\frac{g}{18}\left(\frac{{\rho }_s-\rho }{\mu }\right)d^2\\ =\frac{9.81}{18}\left(\frac{7.874-1}{0.0014}\right){0.0002}^2\\ =0.107038\end{array}$

Now the modified secant method can be started. This will be done using MATLAB.

Code:

The mod_secant.m file

% A function that uses the modified secant method with maximum iterations N, a tolerance of tol

% an initial value of x_0 and delta as delx

functionmod_secant(f,delx,x_0,N,tol)

clc;

format long g

% Initialize the solution as the initial value

sol(1)=x_0;

% Counter

k=1;

% This needs to be grater than the tolerance specified

app_err=100000;

% The code will run till the err<tol and the maximum iterations have not been reached

while(k<N &app_err>tol)

% The formula for the modified secant method

% Re(k)=sig*sol(k)*d/u;

fdiff(k)=(f(sol(k)+delx)-f(sol(k)))/delx;

sol(k+1)=sol(k)-f(sol(k))/fdiff(k);

if k>=1

% Calculate the relative approximate error

app_err(k+1)=abs((sol(k+1)-sol(k))/sol(k+1))*100;

end

k=k+1;

end

% Store the results in new column matrices

Iteration=[0;1;2;3];

Velocity=sol';

Error=app_err';

% Replace the starting entry for app_err as 100000 by 0 as the absolute

% relative error can only be calculated from the first iteration

Error=Error(2:end);

Error=[0;Error];

% Display the results in a table

T=table(Iteration,Velocity,Error)

end

The func.m file

function y=func(v);

d=0.0002;sig=1000;u=0.0014;sig_s=7874;g=9.81;

a=4*g*(sig_s-sig)*d;

b=(24*u)./(sig*d*v)+(3*sqrt(u))./(sqrt(sig*d*v))+0.34;

y=sqrt(a./(3*b*sig))-v;

end

Output:

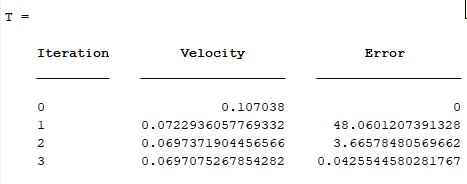
Note that in the third iteration the absolute relative percentage error falls below 0.05%.

Hence the desired velocity can be approximated as $0.0697075\text{ m}/\text{s}$.

To determine

To calculate: The Reynolds number and the drag coefficient for the iron particles that settle in water and then determine whether the flow in laminar or not.

Answer to Problem 48P

Solution: The Reynolds number is 9.95821429 and the drag coefficient is 3.70074225. This implies that the flow is not laminar.

Explanation of Solution

Given Information:

The velocity of the iron particle settling in water is $0.0697075\text{ m}/\text{s}$. The diameter of the iron particle is $0.0002\text{ m}$, the density of the iron particle ${\rho }_s$ is $7874\text{ kg}/{\text{m}}^3$, the viscosity of water $\mu$ is $0.0014\text{ kg}/\text{m}\cdot \text{s}$ and the density $\rho$ of water is $1000\text{ kg}/{\text{m}}^3$.

Formula Used:

The relation between the fluid’s velocity in $\text{m}/\text{s}$, v and the dimensionless Reynolds number, Re, is:

$\mathrm{Re}=\frac{\rho dv}{\mu }$

Here, d is the diameter of the object in m, $\rho$ is the density of the object in $\text{kg}/{\text{m}}^3$ and $\mu$ is the fluids viscosity in $\text{Ns}/{\text{m}}^2$.

$C_D$ is the drag coefficient of a particle whose value is given by:

$C_D=\frac{24}{\mathrm{Re}}+\frac{3}{\sqrt{\mathrm{Re}}}+0.34$

Calculation:

Substitute 0.0697075 for the v, 0.0002 for d, 1000 for $\rho$ and0.0014 for $\mu$ in the formula of the Reynolds number to obtain:

$\begin{array}{c}\mathrm{Re}=\frac{\rho dv}{\mu }\\ =\frac{\left(1000\right)\left(0.0002\right)0.0697075}{0.0014}\\ =9.95821429\end{array}$

Hence, the Reynolds number is 9.95821429. As this is greater than 0.1, the flow is non-laminar.

Now substitute 9.95821429 for Re in the formula for the drag-coefficient to obtain:

$\begin{array}{c}{C}_D=\frac{24}{\mathrm{Re}}+\frac{3}{\sqrt{\mathrm{Re}}}+0.34\\ =\frac{24}{9.95821429}+\frac{3}{\sqrt{9.95821429}}+0.34\\ =3.70074225\end{array}$

Thus, the drag coefficient is 3.70074225.

To determine

To calculate: The zero of the function obtained in part (a) using the fixed point method where ${\epsilon }_s$ is 0.05% and obtain the velocity of an iron particle settling in water.

Answer to Problem 48P

Solution: The velocity of the iron particle settling in water is $0.0697226\text{ m}/\text{s}$.

Explanation of Solution

Given Information:

The diameter of the iron particle is $200\mu m$, the density of the iron particle ${\rho }_s$ is $7.874\text{ g}/{\text{cm}}^3$, the viscosity of water $\mu$ is $0.014\text{g}/\text{cm}\cdot \text{s}$.

Formula Used:

The iterative scheme for the fixed point method is of the form:

$x_{i+1}=f\left(x_i\right)$

Calculation:

Note that the dimensions of the diameter, particle density, water density, and the viscosity of water are different to what can be used in the equation obtained in part (a).

Convert the dimensions as follows:

$\begin{array}{c}d=200\mu \text{m}\\ =200\mu \text{m}\cdot \frac{\text{m}}{{10}^6\mu \text{m}}\\ =0.0002\text{m}\end{array}$

And,

$\begin{array}{c}\rho =1\text{g}/{\text{cm}}^3\\ =1\text{g}/{\text{cm}}^3\cdot \frac{{10}^6{\text{cm}}^3}{{\text{m}}^3}\cdot \frac{\text{kg}}{{10}^3\text{g}}\\ =1000\text{ kg}/{\text{m}}^3\end{array}$

And,

$\begin{array}{c}\mu =0.014\frac{g}{\text{cm s}}\\ =0.014\frac{g}{\text{cms}}\cdot \frac{100\text{cm}}{\text{m}}\cdot \frac{\text{kg}}{{\text{10}}^3\text{g}}\\ =0.0014\frac{\text{kg}}{\text{m s}}\end{array}$

And,

$\begin{array}{c}{\rho }_s=7.874\text{g}/{\text{cm}}^3\\ =7.874\text{g}/{\text{cm}}^3\cdot \frac{{10}^6{\text{cm}}^3}{{\text{m}}^3}\cdot \frac{\text{kg}}{{10}^3\text{g}}\\ =7874\text{ kg}/{\text{m}}^3\end{array}$

The fixed point method requires an initial guess. This can be obtained using Stoke’s law.

$\begin{array}{c}v=\frac{g}{18}\left(\frac{{\rho }_s-\rho }{\mu }\right)d^2\\ =\frac{9.81}{18}\left(\frac{7.874-1}{0.0014}\right){0.0002}^2\\ =0.107038\end{array}$

Consider the equation whose root needs to obtain as velocity:

$g\left(v\right)=\sqrt{\frac{4g\left({\rho }_s-\rho \right)d}{3\left(\frac{24\mu }{\rho dv}+\frac{3\sqrt{\mu }}{\sqrt{\rho dv}}+0.34\right)\rho }}$

Now proceed with the fixed-point method using MATLAB.

Code:

The fixed_point.m file

% A function that uses the fixed point method with maximum iterations N, a tolerance of tol

% an initial value of x_0

functionfixed_point(f,x_0,N,tol)

clc;

format long g

% Initialize the solution as the initial value

sol(1)=x_0;

% Counter

k=1;

% This needs to be grater than the tolerance specified

app_err=100000;

% The code will run till the err<tol and the maximum iterations have not been reached

while(k<N &app_err>tol)

sol(k+1)=f(sol(k));

if k>=1

% Calculate the relative approximate error

app_err(k+1)=abs((sol(k+1)-sol(k))/sol(k+1))*100;

end

k=k+1;

end

% Store the results in new column matrices

Iteration=1:length(sol);

Iteration=Iteration';

Velocity=sol';

Error=app_err';

% Replace the starting entry for app_err as 100000 by 0 as the absolute

% relative error can only be calculated from the first iteration

Error=Error(2:end);

Error=[0;Error];

% Display the results in a table

T=table(Iteration,Velocity,Error)

end

The g.m file

function y=g(v);

d=0.0002;sig=1000;u=0.0014;sig_s=7874;g=9.81;

a=4*g*(sig_s-sig)*d;

b=(24*u)./(sig*d*v)+(3*sqrt(u))./(sqrt(sig*d*v))+0.34;

y=sqrt(a./(3*b*sig));

end

Output:

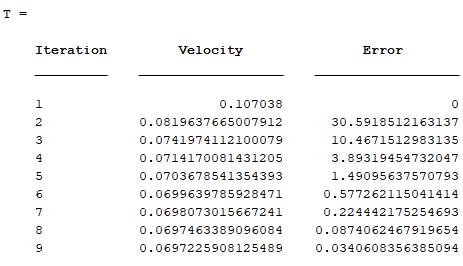
This shows that after the 9^th iteration, the approximate relative percentage error reaches below 0.05%.

Hence the desired velocity can be approximated as $0.0697226\text{ m}/\text{s}$.

To determine

To prove: The function whose root was computed in part (d) would converge using the fixed point iteration irrespective of a positive initial guess.

Explanation of Solution

Given Information:

The diameter of the iron particle is $0.0002\text{ m}$, the density of the iron particle ${\rho }_s$ is $7874\text{ kg}/{\text{m}}^3$, the viscosity of water $\mu$ is $0.0014\text{ kg}/\text{m}\cdot \text{s}$ and the density $\rho$ of water is $1000\text{ kg}/{\text{m}}^3$.

Formula Used:

The function in terms of the velocity whose zero or root was computed in part (d) was

$g\left(v\right)=\sqrt{\frac{4g\left({\rho }_s-\rho \right)d}{3\left(\frac{24\mu }{\rho dv}+\frac{3\sqrt{\mu }}{\sqrt{\rho dv}}+0.34\right)\rho }}$

Proof:

The fixed point iteration for a function $f\left(x\right)$ works based on the following equation:

$f\left(x\right)=x$ ……$\left(1\right)$

This is iteratively rewritten as:

$x_{i+1}=f\left(x_i\right)$

Use equation $\left(1\right)$ to set up a similar equation for the provided problem as:

$\begin{array}{c}g\left(v\right)=v\\ \sqrt{\frac{4g\left({\rho }_s-\rho \right)d}{3\left(\frac{24\mu }{\rho dv}+\frac{3\sqrt{\mu }}{\sqrt{\rho dv}}+0.34\right)\rho }}=v\end{array}$

Now graph the functions on the right and on the left of the provided equation.

This can be done using MATLAB.

Code:

clc; clear all;

% Create a discrete domain for v

v=0:0.001:0.16;

% Denote the first function

y1=v;

% Now denote the second function using the provided value of the constants

d=0.0002;sig=1000;u=0.0014;sig_s=7874;g=9.81;

a=4*g*(sig_s-sig)*d;

b=(24*u)./(sig*d*v)+(3*sqrt(u))./(sqrt(sig*d*v))+0.34;

y2=sqrt(a./(3*b*sig));

% Now plot the two functions

plot(v,y1,v,y2)

legend('v','g(v)')

Graph:

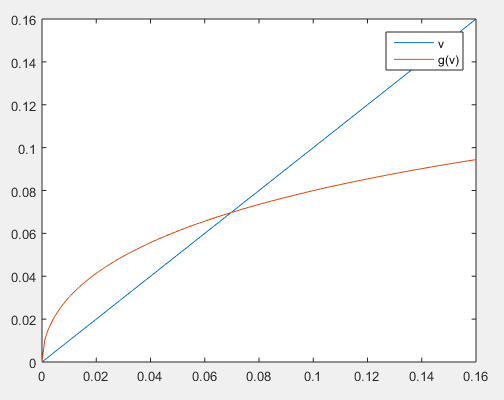
Interpretation:

Note that the graph is divided into two parts. The first part where the function $g\left(v\right)$ lies above v and the second part where $g\left(v\right)$ lies below v.

For both the parts, it is clear that any positive guess say $x_0$ would give have a corresponding value $f\left(x_0\right)$.

A line drawn horizontal from $\left(x_0,f\left(x_0\right)\right)$ would intersect with the curve $y=v$ at a point say $x_1$.

Now proceeding the same way as before, the iterative method would approach the true root. Note that this would occur irrespective of the positive value of $x_0$.

This can be illustrated as:

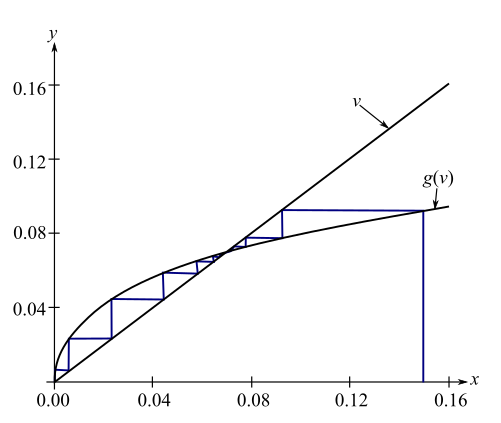
Hence, the fixed point method would converge irrespective of the positive initial guess $x_0$.