Chapter 7.3, Problem 55E

(a)

To determine

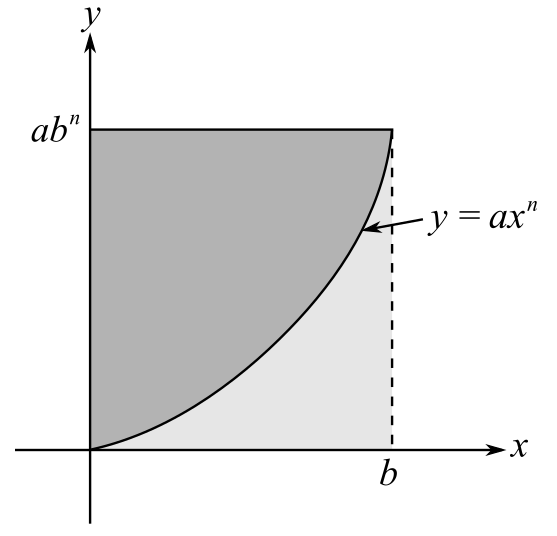
To calculate: The ratio $R_1\left(n\right)$ of the area of the region bounded by the graphs of $y=a{x}^n$, $y=a{b}^n$ and $x=0$ to the area of the circumscribed rectangle using the graph as shown below.

(b)

To determine

To calculate: The limit $\underset{n\to \infty }{\lim }{R}_1\left(n\right)$ and compare it with the area of circumscribed rectangle using the graph as shown in the figure below:

(c)

To determine

To calculate: The volume of the solid of revolution formed by revolving the region about the $y$-axis and find the ratio $R_2\left(n\right)$ of this volume to the volume of circumscribed right circular cylinder using the graph as shown in the figure below:

(d)

To determine

To calculate: The limit $\underset{n\to \infty }{\lim }{R}_2\left(n\right)$ and compare it with the volume of circumscribed cylinder using the graph as shown in the figure below:

(e)

To determine

A conjecture about the shape of the graph of $y=a{x}^n$, $0\le x\le b$ as $n\to \infty$ from the result of part (b) and part (d).