Chapter 72, Problem 42A

To determine

The distance $x$.

Answer to Problem 42A

The distance $x$ is $1.041\text{in}$.

Explanation of Solution

The below figure represent the schematic diagram of the system.

  

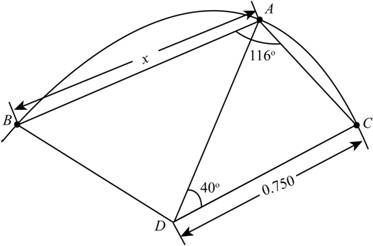
       Figure -(1)

Write the expression of cosine rule in $\Delta ADC$ for the angle $\angle ADC$.

 $\cos \angle ADC=\frac{A{C}^2-A{D}^2-D{C}^2}{2\left(AD\right)\left(DC\right)}$ ......... (I)

Here, the sides of $\Delta ADC$ is $AD,DC\text{and}AC$ respectively.

Write the expression for the relation between angles in $\Delta ADC$.

 $\angle ACD=\angle CAD$ ...... (II)

Write the expression of sum of angles in $\Delta ADC$ .

 $\begin{array}{l}\angle ACD+\angle CAD+\angle ADC=180°\\ \angle CAD+\angle CAD+\angle ADC=180°\\ 2\angle CAD+\angle ADC=180°\end{array}$ ...... (III)

Write the expression of $\angle DAB$.

 $\angle DAB=\angle BAC-\angle CAD$ ...... (IV)

Write the expression for the relation between angles in $\Delta ABD$.

 $\angle DAB=\angle DBA$ ...... (V)

Write the expression of $\angle ADB$.

 $\begin{array}{c}\angle ADB=180°-\angle DAB-\angle DBA\\ =180°-\angle DAB-\angle DAB\\ =180°-2\angle DAB\end{array}$ ...... (VI)

Write the expression of cosine rule in $\Delta ADB$.

 $A{B}^2=A{D}^2+A{B}^2-2\left(AD\right)\left(AB\right)\cos \angle ADB$ ......... (VII)

Calculation:

Substitute $40°$ for $\angle ADC$, $0.750\text{in}$ for $AD$, $0.750\text{in}$ for $DC$ in Equation (I).

 $\begin{array}{l}\cos 40°=\frac{A{C}^2-{\left(0.750\text{in}\right)}^2-{\left(0.750\text{in}\right)}^2}{2\left(0.750\text{in}\right)\left(0.750\text{in}\right)}\\ A{C}^2-{\left(0.750\text{in}\right)}^2-{\left(0.750\text{in}\right)}^2=\left(0.8618{\text{in}}^2\right)\\ A{C}^2=0.2632{\text{in}}^2\\ AC=0.513\text{in}\end{array}$

Substitute $40°$ for $\angle ADC$ in Equation (III).

 $\begin{array}{l}2\angle CAD+40°=180°\\ 2\angle CAD=180°-40°\\ \angle CAD=\frac{140°}{2}\\ \angle CAD=70°\end{array}$

Substitute $116°$ for $\angle BAC$ and $70°$ for $\angle CAD$ in Equation (IV).

 $\begin{array}{c}\angle DAB=116°-70°\\ =46°\end{array}$

Substitute $46°$ for $\angle DAB$ in Equation (VI).

 $\begin{array}{c}\angle ADB=180°-2\left(46°\right)\\ =180°-92°\\ =88°\end{array}$

Substitute $x$ for $AB$, $88°$ for $\angle DAB$, $0.750\text{in}$ for $AD$, $0.750\text{in}$ for $AB$ in Equation (VII).

 $\begin{array}{l}{x}^2={\left(0.750\text{in}\right)}^2+{\left(0.750\text{in}\right)}^2-2\left(0.750\text{in}\right)\left(0.750\text{in}\right)\cos 88°\\ x^2=1.0857{\text{in}}^2\\ x=\sqrt{1.0857{\text{in}}^2}\\ x=1.041\text{in}\end{array}$

Conclusion:

The distance $x$ is $1.041\text{in}$.