Concept explainers
Videos
To sketch:
A right triangle for the given angle and the sides of the triangle are to be labelled as positive or negative.
Label the sides of the triangle as positive or negative.
To find: The reference angles and functions of the angles.
The value of sine, cosine, tangent, cotangent, cosecant, secant for a given angle, up to 4 decimals.
Answer to Problem 13A
Reference angle is 28°50'.
Sin 208°50' is -0.4822. It is a negative function.
Cos 208°50' is -0.876. It is a negative function.
Tan 208°50' is 0.5504. It is a positive function.
Cot 208°50' is 1.8167. It is a positive function.
Sec 208°50' is -1.1414. It is a negative function.
Cosec 208°50' is -2.073. It is a negative function.
Explanation of Solution
Given Information:
θ= 208°50'
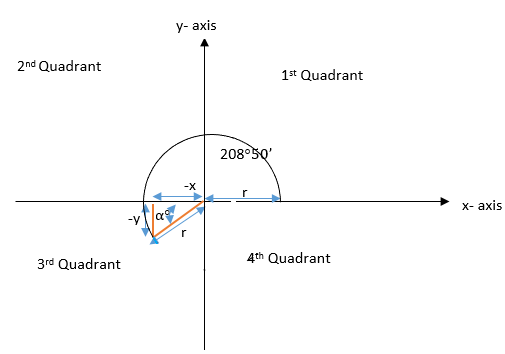
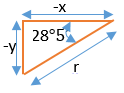
In Quadrant 3, y is negative and x is negative.
In the above triangle, r is the hypotenuse, the adjacent side x is negative, the opposite side y is negative.
Conclusion:
Reference angle is 28°50'.
Sin 208°50' is -0.4822. It is a negative function.
Cos 208°50' is -0.876. It is a negative function.
Tan 208°50' is 0.5504. It is a positive function.
Cot 208°50' is 1.8167. It is a positive function.
Sec 208°50' is -1.1414. It is a negative function.
Cosec 208°50' is -2.073. It is a negative function.
Want to see more full solutions like this?
Chapter 71 Solutions
Mathematics For Machine Technology
- (b) 313 dy dx -y= 10 sin(2x)y; y(x) = ex-5 cos(2x)arrow_forward5 сл Use vectors to prove the following theorems from geometry: (a) The diagonals of a parallelogram bisect each other. (b) The median to the base of an isosceles triangle is perpendicular to the base.arrow_forward5 сл Use vectors to prove the following theorems from geometry: (a) The diagonals of a parallelogram bisect each other. (b) The median to the base of an isosceles triangle is perpendicular to the base.arrow_forward
- List the possible values of a for which square root is between 6 and 7 and an element of natural numberarrow_forwardQuestion 25 Jasmine bought a house for $225 000. She already knows that for the first $200 000, the land transfer tax will cost $1650. Calculate the total land transfer tax. (2 marks) Land Transfer Tax Table Value of Property Rate On the first $30 000 0% On the next $60 000 0.5% (i.e., $30 001 to $90 000) On the next $60 000 1.0% (i.e., $90 001 to $150 000) On the next $50 000 1.5% (i.e., $150 001 to $200 000) On amounts in excess of $200 000 2.0% 225000–200 000 = 825000 25000.002 × 25000 1= 8500 16 50+ 500 2 marksarrow_forwardTaxable income (R) 1-216 200 216 201-337 800 337 801-467 500 2021/2022 TAX YEAR Rates of tax (R) 18% of taxable income 38 916 +26% of taxable income above 216 200 70 532 + 31% of taxable income above 337 800 110 739 + 36% of taxable income above 467 500 467 501-613 600 613 601-782 200 163 335 + 39% of taxable income above 613 600 782 201-1656 600 229 089 +41% of taxable income above 782 200 4 1 656 601 and above 587 593 + 45% of taxable income above 1 656 600 TAX REBATES • A special relief/discount given to taxpayers. It is subtracted after the income tax has been calculated. • Every person qualifies for primary rebate. Older people are granted additional rebate i.e. If you are older than 65 years of age you will receive Primary and secondary rebate; If you are over 75 years you will receive primary, secondary, and tertiary rebate. Tax rebate type Primary (age below 65) Secondary (65 to 74) Tertiary (75 and older) 2021 R14 958 2022 R15 714 R8 199 R8 613 R2 736 R2 871 • TAX THRESHOLD…arrow_forward
- 25 Topology: Properties of Compact Spaces Task: Refer to Question 25 in the provided document. Link: https://drive.google.com/file/d/1wKSrun-GlxirS3IZ9qoHazb9tC44OAZF/view?usp=sharingarrow_forward4 6 Spectral Theory: Eigenfunctions and Eigenvalues Task: Refer to Question 46 in the provided document. Link: https://drive.google.com/file/d/1wKSrun-GlxirS3IZ9qoHazb9tC440AZF/view?usp=sharing 47 Category Theory: Functors and Natural Transformations Task: Refer to Question 47 in the provided document. Link: https://drive.google.com/file/d/1wKSrun-GlxirS3IZ9qoHazb9tC440AZF/view?usp=sharingarrow_forward= Consider the vector space R² with the standard basis {e1 {v1 = (1, 1), V2 = = (1, 0), e2 = (0, 1)} and a new basis new basis. (1,-1)}. a) Find the change of basis matrix from the standard basis to the b) Express the vector w = (2, 3) in the new basis {V1, V2}.arrow_forward
- Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning 
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage Learning