
Concept explainers
(a)
To calculate: The positive real root of
(a)
Answer to Problem 3P
Solution:
The positive real root of the given equation
Explanation of Solution
Given Information:
The given equation is,
Use Müller’s method.
Formula used:
The expression for the new roots is,
The expression for the error is,
Calculation:
Recall the equation mentioned in the problem,
Draw the plot of the equation.
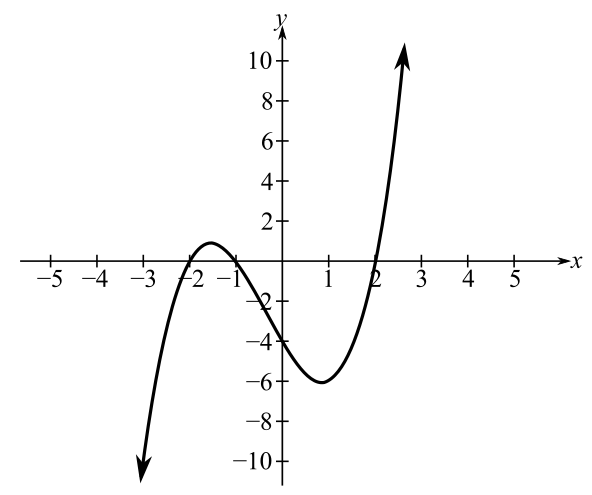
From the above plot it is clear that one root is at about
Consider the initial guess be
, and
Thus, the values of
Now calculate the
Substituting the value of
, and
Now calculate the
Substitute the above calculated values.
And,
Calculate the value of constants
For b,
For c,
Thus, the new root is calculated as follows.
Substitute the all values.
Calculate the error estimate.
Because the error is large, so required new guesses for
,
, and
Therefore, for the new iteration,
,
,
Thus, the values of
Now calculate the
Substituting the value of
, and
Now calculate the
Substitute the above calculated values.
And,
Calculate the value of constants
For b,
For c,
Thus, the new root is calculated as follows.
Substitute the all values.
Calculate the error estimate.
Because the error is near about 1, so required new guesses for
,
, and
. And repeat the same process will get the root is
| error | ||
| 0 | 1.979384 | 26.03 |
| 1 | 1.8 | |
| 2 | 2 | 0.00241 |
Similarly repeat for all other roots.
To find the exact positive real root use MATLAB.
Write the following code in command window.
Therefore, the only positive real root of the given equation
(b)
To calculate: The positive real root of
(b)
Answer to Problem 3P
Solution:
The only positive real root of the given equation
Explanation of Solution
Given Information:
The given equation is,
Use Müller’s method.
Formula used:
The expression for the new roots is,
The expression for the error is,
Calculation:
Recall the equation mentioned in the problem,
Draw the plot of the equation.
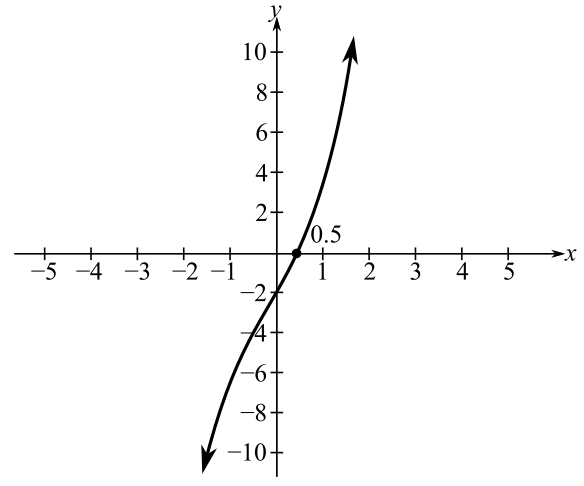
From the above plot it is clear that one root is at about
Consider the initial guess be
, and
Thus, the values of
Now calculate the
Substituting the value of
, and
Now calculate the
Substitute the above calculated values.
And,
Calculate the value of constants
For b,
For c,
Thus, the new root is calculated as follows.
Substitute the all values.
Calculate the error estimate.
Because the error is large, so required new guesses for
,
, and
Therefore, for the new iteration,
,
,
Thus, the values of
Now calculate the
Substituting the value of
,
,
Now calculate the
Substitute the above calculated values.
And,
Calculate the value of constants
For b,
For c,
Thus, the new root is calculated as follows.
Substitute the all values.
Calculate the error estimate.
Because the error is zero, the root is
| error | ||
| 0 | 200% | |
| 1 | 0% |
Similarly repeat for all other roots.
To find the exact positive real root use MATLAB.
Write the following code in command window.
Therefore, the only positive real root of the given equation
Want to see more full solutions like this?
Chapter 7 Solutions
EBK NUMERICAL METHODS FOR ENGINEERS
- Theorem 1: A number n ∈ N is divisible by 3 if and only if when n is writtenin base 10 the sum of its digits is divisible by 3. As an example, 132 is divisible by 3 and 1 + 3 + 2 is divisible by 3.1. Prove Theorem 1 2. Using Theorem 1 construct an NFA over the alphabet Σ = {0, 1, 2, 3, 4, 5, 6, 7, 8, 9}which recognizes the language {w ∈ Σ^(∗)| w = 3k, k ∈ N}.arrow_forwardRecall the RSA encryption/decryption system. The following questions are based on RSA. Suppose n (=15) is the product of the two prime numbers 3 and 5.1. Find an encryption key e for for the pair (e, n)2. Find a decryption key d for for the pair (d, n)3. Given the plaintext message x = 3, find the ciphertext y = x^(e) (where x^e is the message x encoded with encryption key e)4. Given the ciphertext message y (which you found in previous part), Show that the original message x = 3 can be recovered using (d, n)arrow_forwardFind the sum of products expansion of the function F(x, y, z) = ¯x · y + x · z in two ways: (i) using a table; and (ii) using Boolean identities.arrow_forward
- Give both a machine-level description (i.e., step-by-step description in words) and a state-diagram for a Turing machine that accepts all words over the alphabet {a, b} where the number of a’s is greater than or equal to the number of b’s.arrow_forwardCompute (7^ (25)) mod 11 via the algorithm for modular exponentiation.arrow_forwardProve that the sum of the degrees in the interior angles of any convex polygon with n ≥ 3 sides is (n − 2) · 180. For the base case, you must prove that a triangle has angles summing to 180 degrees. You are permitted to use thefact when two parallel lines are cut by a transversal that corresponding angles are equal.arrow_forward
- Answer the following questions about rational and irrational numbers.1. Prove or disprove: If a and b are rational numbers then a^b is rational.2. Prove or disprove: If a and b are irrational numbers then a^b is irrational.arrow_forwardProve the following using structural induction: For any rooted binary tree T the number of vertices |T| in T satisfies the inequality |T| ≤ (2^ (height(T)+1)) − 1.arrow_forward(a) Prove that if p is a prime number and p|k^2 for some integer k then p|k.(b) Using Part (a), prove or disprove: √3 ∈ Q.arrow_forward
- Provide a context-free grammar for the language {a^ (i) b^ (j) c^ (k) | i, j, k ∈ N, i = j or i = k}. Briefly explain (no formal proof needed) why your context-free grammar is correct and show that it produces the word aaabbccc.arrow_forwardDo College Students With Part-Time Jobs Sleep Less? College students were surveyed about the number of hours they sleep each night.Group A = With part-time jobs | Group B = Without jobs Group A: 6, 5, 7, 6, 5Group B: 8, 7, 9, 8, 7 Instructions: State your hypothesis and perform a two-sample t-test with all formulas. Create histograms for each group. Label axes and add titles. Comment on the distribution shape (e.g., normal, skewed, etc.).Solve on pen and paperarrow_forwardThis is advanced mathematics question that need detailed solutionsarrow_forward
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,






