
1-4 Modeling Periodic Data A set of data is given.
(a) Make a
(b) Find a cosine function of the form
(c) Graph the function you found in part (b) together with the scatter plot. How well does the curve fit the data?
(d) Use a graphing calculator to find the sine function that best fits the data, as in Example 2.
(e) Compare the functions you found in parts (b) and (d). [Use the reduction formula
| t | y |
| 0 | 2.1 |
| 2 | 1.1 |
| 4 |
|
| 6 |
|
| 8 |
|
| 10 | 0.6 |
| 12 | 1.9 |
| 14 | 1.5 |
(a)
To find:
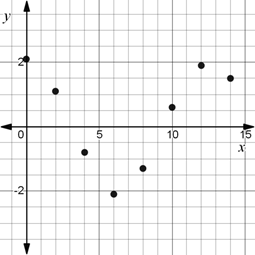
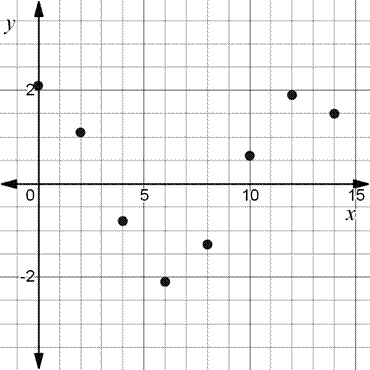
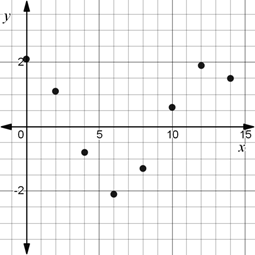
A scatter plot of the data.
Answer to Problem 1P
Solution:
Explanation of Solution
Calculation:
The scatter plot for the given data is given by
Final statement:
(b)
To find:
A cosine function of the form
Answer to Problem 1P
Solution:
Explanation of Solution
Calculation:
Here, the maximum value is 2.1 and the minimum value is -2.1.
The maximum value occurs at time 0 and the minimum value occurs at time 6.
The vertical shift
The amplitude
The time between consecutive maximum and minimum values is half of one period.
That is,
Since the maximum value of the data occurs at
There is no horizontal shift.
Apply the values in the function
Since cosine is an even function,
Final statement:
(c)
To find:
The graph of the function from part (b).
Answer to Problem 1P
Solution:
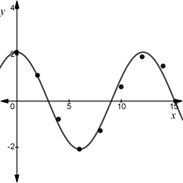
Explanation of Solution
Calculation:
The graph of the function
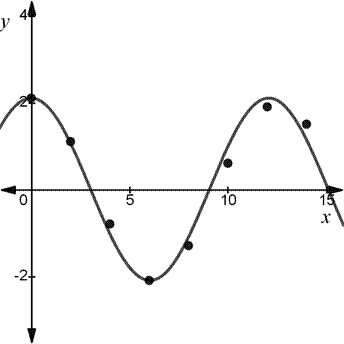
Final statement:
(d)
To find:
The sine function that fits the data.
Answer to Problem 1P
Solution:
Explanation of Solution
Calculation:
Using the given data and the SinReg command on the T1-83 calculator, we get a function of the form
Here,
Apply the values in the function
Final statement:
(e)
To find:
The comparison between the functions from part (b) and (d).
Answer to Problem 1P
Solution:
It is same as (b), corrected to one decimal.
Explanation of Solution
Calculation:
From part (d),
Apply the reduction formula
So,
It is same as (b), corrected to one decimal.
Final statement:
It is same as (b), corrected to one decimal.
Want to see more full solutions like this?
Chapter 6 Solutions
ALGEBRA AND TRIGONOMETRY-WEBASSIGN
Additional Math Textbook Solutions
Elementary & Intermediate Algebra
College Algebra (Collegiate Math)
Elementary Statistics Using The Ti-83/84 Plus Calculator, Books A La Carte Edition (5th Edition)
University Calculus
Elementary Statistics ( 3rd International Edition ) Isbn:9781260092561
- 3. A scientist recorded the movement of a pendulum for 10 s. The scientist began recording when the pendulum was at its resting position. The pendulum then moved right (positive displacement) and left (negative displacement) several times. The pendulum took 4 s to swing to the right and the left and then return to its resting position. The pendulum's furthest distance to either side was 6 in. Graph the function that represents the pendulum's displacement as a function of time. Answer: f(t) (a) Write an equation to represent the displacement of the pendulum as a function of time. (b) Graph the function. 10 9 8 7 6 5 4 3 2 1 0 t 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 -1 -5. -6 -7 -8 -9 -10-arrow_forwardA power outage occurs 6 min after the ride started. Passengers must wait for their cage to be manually cranked into the lowest position in order to exit the ride. Sine function model: h = −82.5 cos (3πt) + 97.5 where h is the height of the last passenger above the ground measured in feet and t is the time of operation of the ride in minutes. (a) What is the height of the last passenger at the moment of the power outage? Verify your answer by evaluating the sine function model. (b) Will the last passenger to board the ride need to wait in order to exit the ride? Explain.arrow_forwardThe Colossus Ferris wheel debuted at the 1984 New Orleans World's Fair. The ride is 180 ft tall, and passengers board the ride at an initial height of 15 ft above the ground. The height above ground, h, of a passenger on the ride is a periodic function of time, t. The graph displays the height above ground of the last passenger to board over the course of the 15 min ride. Height of Passenger in Ferris Wheel 180 160 140- €120 Height, h (ft) 100 80 60 40 20 0 ך 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 Time of operation, t (min) Sine function model: h = −82.5 cos (3πt) + 97.5 where h is the height of the passenger above the ground measured in feet and t is the time of operation of the ride in minutes. What is the period of the sine function model? Interpret the period you found in the context of the operation of the Ferris wheel. Answer:arrow_forward
- 1. Graph the function f(x)=sin(x) −2¸ Answer: y -2π 一元 1 −1 -2 -3 -4+ 元 2πarrow_forward3. Graph the function f(x) = −(x-2)²+4 Answer: f(x) 6 5 4 3 2+ 1 -6-5 -4-3-2-1 × 1 2 3 4 5 6 -1 -2+ ရာ -3+ -4+ -5 -6arrow_forward2. Graph the function f(x) = cos(2x)+1 Answer: -2π 一元 y 3 2- 1 -1 -2+ ရာ -3- Π 2πarrow_forward
- 2. Graph the function f(x) = |x+1+2 Answer: -6-5-4-3-2-1 f(x) 6 5 4 3 2 1 1 2 3 4 5 6 -1 -2 -3 -4 -5 -6arrow_forward1. The table shows values of a function f(x). What is the average rate of change of f(x) over the interval from x = 5 to x = 9? Show your work. X 4 f(x) LO 5 6 7 8 9 10 -2 8 10 11 14 18arrow_forward• Find a real-world situation that can be represented by a sinusoidal function. You may find something online that represents a sinusoidal graph or you can create a sinusoidal graph yourself with a measuring tape and a rope. • Provide a graph complete with labels and units for the x- and y-axes. • Describe the amplitude, period, and vertical shift in terms of the real-world situation.arrow_forward
- f(x) = 4x²+6x 2. Given g(x) = 2x² +13x+15 and find 41 (4)(x) Show your work.arrow_forwardf(x) = x² − 6x + 8 3. Given and g(x) = x -2 solve f(x) = g(x) using a table of values. Show your work.arrow_forward1. Graph the function f(x) = 3√x-2 Answer: -6-5 -4-3-2 -1 6 LO 5 f(x) 4 3 2+ 1 1 2 3 4 5 6 -1 -2+ -3 -4 -5 -6- 56arrow_forward
- Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
