
Algebra and Trigonometry
1st Edition
ISBN: 9781938168376
Author: Jay Abramson
Publisher: OpenStax
expand_more
expand_more
format_list_bulleted
Concept explainers
Textbook Question
Chapter 6.8, Problem 51SE
For the following exercises, refer to Table 12.
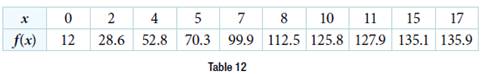
Use a graphing calculator to create a
Expert Solution & Answer
Trending nowThis is a popular solution!

Students have asked these similar questions
Write the equation of the trigonometric
function shown in the graph.
LO
5
4
3
2
1
y
-5
-5
4
8
8
500
-1
-2
-3
-4
-5
x
5
15л
5л
25л
15л
35π
5л
4
8
2
8
4
8
2. If log2 (sin x) + log₂ (cos x) = -2 and log2 (sin x + cos x) = (-2 + log2 n), find n.
If cscx- cotx = -4, find cscx + cotx.
Chapter 6 Solutions
Algebra and Trigonometry
Ch. 6.1 - Which of the following equations represent...Ch. 6.1 - Let f(x)=8(1.2)x5. Evaluate f(3) using a...Ch. 6.1 - The population of China was about 1.39 billion in...Ch. 6.1 - A wolf population is growing exponentially. In...Ch. 6.1 - Given the two points (1,3) and (2,4.5) , find the...Ch. 6.1 - Find an equation for the exponential function...Ch. 6.1 - Use a graphing calculator to find the exponential...Ch. 6.1 - An initial investment of 100,000 at 12 interest is...Ch. 6.1 - Refer to Example 9. To the nearest dollar, how...Ch. 6.1 - Use a calculator to find e0.5. Round to five...
Ch. 6.1 - A person invests 100,000 at a nominal 12 interest...Ch. 6.1 - Using the data in Example 12, how much radon-222...Ch. 6.1 - Explain why the values of an increasing...Ch. 6.1 - Given a formula for an exponential function, is...Ch. 6.1 - The Oxford Dictionary defines the word nominal asa...Ch. 6.1 - For the following exercises, identify whether the...Ch. 6.1 - For the following exercises, identify whether the...Ch. 6.1 - For the following exercises, identify whether the...Ch. 6.1 - For the following exercises, identify whether the...Ch. 6.1 - For the following exercises, identify whether the...Ch. 6.1 - For the following exercises, consider this...Ch. 6.1 - For the following exercises, consider this...Ch. 6.1 - For the following exercises, consider this...Ch. 6.1 - For the following exercises, consider this...Ch. 6.1 - For the following exercises, consider this...Ch. 6.1 - For the following exercises, determine whether the...Ch. 6.1 - For the following exercises, determine whether the...Ch. 6.1 - For the following exercises, determine whether the...Ch. 6.1 - For the following exercises, determine whether the...Ch. 6.1 - For the following exercises, find the formula for...Ch. 6.1 - For the following exercises, find the formula for...Ch. 6.1 - For the following exercises, find the formula for...Ch. 6.1 - For the following exercises, find the formula for...Ch. 6.1 - For the following exercises, find the formula for...Ch. 6.1 - For the following exercises, determine whether the...Ch. 6.1 - For the following exercises, determine whether the...Ch. 6.1 - For the following exercises, determine whether the...Ch. 6.1 - For the following exercises, determine whether the...Ch. 6.1 - For the following exercises, determine whether the...Ch. 6.1 - For the following exercises, use the compound...Ch. 6.1 - For the following exercises, use the compound...Ch. 6.1 - For the following exercises, use the compound...Ch. 6.1 - For the following exercises, use the compound...Ch. 6.1 - For the following exercises, use the compound...Ch. 6.1 - For the following exercises, use the compound...Ch. 6.1 - For the following exercises, use the compound...Ch. 6.1 - For the following exercises, use the compound...Ch. 6.1 - For the following exercises, use the compound...Ch. 6.1 - For the following exercises, use the compound...Ch. 6.1 - For the following exercises, use the compound...Ch. 6.1 - For the following exercises, determine whether the...Ch. 6.1 - For the following exercises, determine whether the...Ch. 6.1 - For the following exercises, determine whether the...Ch. 6.1 - For the following exercises, determine whether the...Ch. 6.1 - For the following exercises, determine whether the...Ch. 6.1 - For the following exercises, evaluate each...Ch. 6.1 - For the following exercises, evaluate each...Ch. 6.1 - For the following exercises, evaluate each...Ch. 6.1 - For the following exercises, evaluate each...Ch. 6.1 - For the following exercises, evaluate each...Ch. 6.1 - For the following exercises, evaluate each...Ch. 6.1 - For the following exercises, evaluate each...Ch. 6.1 - For the following exercises, use a graphing...Ch. 6.1 - For the following exercises, use a graphing...Ch. 6.1 - For the following exercises, use a graphing...Ch. 6.1 - For the following exercises, use a graphing...Ch. 6.1 - For the following exercises, use a graphing...Ch. 6.1 - The annual percentage yield (APY) of an...Ch. 6.1 - Repeat the previous exercise to find the formula...Ch. 6.1 - Recall that an exponential function is any...Ch. 6.1 - In an exponential decay function, the base of...Ch. 6.1 - The formula for the amount A in an...Ch. 6.1 - The fox population in a certain region has an...Ch. 6.1 - A scientist begins with 100 milligrams of...Ch. 6.1 - In the year 1985, a house was valued at 110,000....Ch. 6.1 - A car was valued at 38,000 in the year 2007. By...Ch. 6.1 - Jamal wants to save 54,000 for a down paymenton a...Ch. 6.1 - Kyoko has 10,000 that she wants to invest. Her...Ch. 6.1 - Alyssa opened a retirement account with 7.25 APRin...Ch. 6.1 - An investment account with an annual interest...Ch. 6.2 - Sketch the graph of f(x)=4x. State the domain,...Ch. 6.2 - Try it # 2 Graph f(x)=2x1+3. State domain, range,...Ch. 6.2 - Solve 4=7.85(1.15)x2.27 graphically. Round to the...Ch. 6.2 - Sketch the graph of f(x)=12(4)x. State the domain,...Ch. 6.2 - Find and graph the equation for a function, g(x) ,...Ch. 6.2 - Write the equation for function described below....Ch. 6.2 - What role does the horizontal asymptote of...Ch. 6.2 - What is the advantage of knowing how to...Ch. 6.2 - The graph of f(x)=3x is reflected about the...Ch. 6.2 - The graph of f(x)=(12)x is reflected about...Ch. 6.2 - The graph of f(x)=10x is reflected about the...Ch. 6.2 - The graph of f(x)=(1.68)x is shifted right 3...Ch. 6.2 - The graph of f(x)=2(14)x20 is shifted downward 4...Ch. 6.2 - For the following exercises, graph the function...Ch. 6.2 - For the following exercises, graph the function...Ch. 6.2 - For the following exercises, graph the function...Ch. 6.2 - For the following exercises, graph each set of...Ch. 6.2 - For the following exercises, graph each set of...Ch. 6.2 - For the following exercises, match each function...Ch. 6.2 - For the following exercises, match each function...Ch. 6.2 - For the following exercises, match each function...Ch. 6.2 - For the following exercises, match each function...Ch. 6.2 - For the following exercises, match each function...Ch. 6.2 - For the following exercises, match each function...Ch. 6.2 - For the following exercises, use the graphs shown...Ch. 6.2 - For the following exercises, use the graphs shown...Ch. 6.2 - For the following exercises, use the graphs shown...Ch. 6.2 - For the following exercises, use the graphs shown...Ch. 6.2 - For the following exercises, graph the function...Ch. 6.2 - For the following exercises, graph the function...Ch. 6.2 - For the following exercises, graph the function...Ch. 6.2 - For the following exercises, graph the...Ch. 6.2 - For the following exercises, graph the...Ch. 6.2 - For the following exercises, graph the...Ch. 6.2 - For the following exercises, describe the end...Ch. 6.2 - For the following exercises, describe the end...Ch. 6.2 - For the following exercises, describe the end...Ch. 6.2 - For the following exercises, start with the graph...Ch. 6.2 - For the following exercises, start with the graph...Ch. 6.2 - For the following exercises, start with the graph...Ch. 6.2 - For the following exercises, start with the graph...Ch. 6.2 - For the following exercises, start with the graph...Ch. 6.2 - For the following exercises, start with the graph...Ch. 6.2 - For the following exercises, each graph is a...Ch. 6.2 - For the following exercises, each graph is a...Ch. 6.2 - For the following exercises, each graph is a...Ch. 6.2 - For the following exercises, find an exponential...Ch. 6.2 - For the following exercises, find an exponential...Ch. 6.2 - For the following exercises, evaluate the...Ch. 6.2 - For the following exercises, evaluate the...Ch. 6.2 - For the following exercises, evaluate the...Ch. 6.2 - For the following exercises, use a graphing...Ch. 6.2 - For the following exercises, use a graphing...Ch. 6.2 - For the following exercises, use a graphing...Ch. 6.2 - For the following exercises, use a graphing...Ch. 6.2 - For the following exercises, use a graphing...Ch. 6.2 - Explore and discuss the graphs of f(x)=(b)x and...Ch. 6.2 - Prove the conjecture made in the previous...Ch. 6.2 - Explore and discuss the graphs of...Ch. 6.2 - Prove the conjecture made in the previous...Ch. 6.3 - Write the following logarithmic equations in...Ch. 6.3 - Write the following exponential equations in...Ch. 6.3 - Solve y=log121(11) without using a calculator.Ch. 6.3 - Evaluate y=log2(132) without using a calculator.Ch. 6.3 - Evaluate y=log(1,000,000).Ch. 6.3 - Evaluate y=log(123) to four decimal places using a...Ch. 6.3 - The amount of energy released from one earthquake...Ch. 6.3 - Evaluate ln(500).Ch. 6.3 - What is a base b logarithm? Discuss the meaning...Ch. 6.3 - How is the logarithmic function f(x)=logb(x)...Ch. 6.3 - How can the logarithmic equation logbx=y besolved...Ch. 6.3 - Discuss the meaning of the common logarithm.What...Ch. 6.3 - Discuss the meaning ofthe natural logarithm....Ch. 6.3 - For the following exercises, rewrite each equation...Ch. 6.3 - For the following exercises, rewrite each equation...Ch. 6.3 - For the following exercises, rewrite each equation...Ch. 6.3 - For the following exercises, rewrite each equation...Ch. 6.3 - For the following exercises, rewrite each equation...Ch. 6.3 - For the following exercises, rewrite each equation...Ch. 6.3 - For the following exercises, rewrite each equation...Ch. 6.3 - For the following exercises, rewrite each equation...Ch. 6.3 - For the following exercises, rewrite each equation...Ch. 6.3 - For the following exercises, rewrite each equation...Ch. 6.3 - For the following exercises, rewrite each equation...Ch. 6.3 - For the following exercises, rewrite each equation...Ch. 6.3 - For the following exercises, rewrite each equation...Ch. 6.3 - For the following exercises, rewrite each equation...Ch. 6.3 - For the following exercises, rewrite each equation...Ch. 6.3 - For the following exercises, rewrite each equation...Ch. 6.3 - For the following exercises, rewrite each equation...Ch. 6.3 - For the following exercises, rewrite each equation...Ch. 6.3 - For the following exercises, rewrite each equation...Ch. 6.3 - For the following exercises, rewrite each equation...Ch. 6.3 - For the following exercises, solve for x by...Ch. 6.3 - For the following exercises, solve for x by...Ch. 6.3 - For the following exercises, solve for x by...Ch. 6.3 - For the following exercises, solve for x by...Ch. 6.3 - For the following exercises, solve for x by...Ch. 6.3 - For the following exercises, solve for x by...Ch. 6.3 - For the following exercises, solve for x by...Ch. 6.3 - For the following exercises, solve for x by...Ch. 6.3 - For the following exercises, solve for x by...Ch. 6.3 - For the following exercises, solve for x by...Ch. 6.3 - For the following exercises, use the definition of...Ch. 6.3 - For the following exercises, use the definition of...Ch. 6.3 - For the following exercises, use the definition of...Ch. 6.3 - For the following exercises, use the definition of...Ch. 6.3 - For the following exercises, use the definition of...Ch. 6.3 - For the following exercises, use the definition of...Ch. 6.3 - For the following exercises, evaluate the base b...Ch. 6.3 - For the following exercises, evaluate the base b...Ch. 6.3 - For the following exercises, evaluate the base b...Ch. 6.3 - For the following exercises, evaluate the base b...Ch. 6.3 - For the following exercises, evaluate the common...Ch. 6.3 - For the following exercises, evaluate the common...Ch. 6.3 - For the following exercises, evaluate the common...Ch. 6.3 - For the following exercises, evaluate the common...Ch. 6.3 - For the following exercises, evaluate the natural...Ch. 6.3 - For the following exercises, evaluate the natural...Ch. 6.3 - For the following exercises, evaluate the natural...Ch. 6.3 - For the following exercises, evaluate the natural...Ch. 6.3 - For the following exercises, evaluate each...Ch. 6.3 - For the following exercises, evaluate each...Ch. 6.3 - For the following exercises, evaluate each...Ch. 6.3 - For the following exercises, evaluate each...Ch. 6.3 - For the following exercises, evaluate each...Ch. 6.3 - Is x=0 in the domain of the function f(x)=log(x)...Ch. 6.3 - Is f(x)=0 in the range ofthe function f(x)=log(x)...Ch. 6.3 - Is there a number x such that ln x=2 ? If so,...Ch. 6.3 - Is the following true: log3(27)log4(1 64)=1 ?...Ch. 6.3 - Is the following true: ln(e1.725)ln(1)=1.725 ?...Ch. 6.3 - The exposure index E1 for a 35 millimeter camera...Ch. 6.3 - Refer to the previous exercise. Suppose the...Ch. 6.3 - The intensitylevels I of two earthquakes measured...Ch. 6.4 - What is the domain of f(x)=log5(x2)+1 ?Ch. 6.4 - What is the domain of f(x)=log(x5)+2 ?Ch. 6.4 - Graph f(x)=log15(x). State the domain, range, and...Ch. 6.4 - Sketch a graph of f(x)=log3(x+4) alongside its...Ch. 6.4 - Sketch a graph of f(x)=log2(x)+2 alongside its...Ch. 6.4 - Sketch a graph of f(x)=12log4(x) alongside its...Ch. 6.4 - Sketch a graph of the function f(x)=3log(x2)+1....Ch. 6.4 - Graph f(x)=log(x). State the domain, range, and...Ch. 6.4 - Solve 5log(x+2)=4log(x) graphically. Round to the...Ch. 6.4 - What is the vertical asymptote of f(x)=3+ln(x1) ?Ch. 6.4 - Give the equation ofthe natural logarithm graphed...Ch. 6.4 - The inverse of every logarithmic function is...Ch. 6.4 - What type (s) of translation(s), if any, affect...Ch. 6.4 - What type (s) of translation (s), if any, affect...Ch. 6.4 - Consider the general logarithmic function...Ch. 6.4 - Does the graph of a general logarithmic...Ch. 6.4 - For the following exercises, state the domain and...Ch. 6.4 - For the following exercises, state the domain and...Ch. 6.4 - For the following exercises, state the domain and...Ch. 6.4 - For the following exercises, state the domain and...Ch. 6.4 - For the following exercises, state the domain and...Ch. 6.4 - For the following exercises, state the domain and...Ch. 6.4 - For the following exercises, state the domain and...Ch. 6.4 - For the following exercises, state the domain and...Ch. 6.4 - For the following exercises, state the domain and...Ch. 6.4 - For the following exercises, state the domain and...Ch. 6.4 - For the following exercises, state the domain,...Ch. 6.4 - For the following exercises, state the domain,...Ch. 6.4 - For the following exercises, state the domain,...Ch. 6.4 - For the following exercises, state the domain,...Ch. 6.4 - For the following exercises, state the domain,...Ch. 6.4 - For the following exercises, state the domain,...Ch. 6.4 - For the following exercises, state the domain,...Ch. 6.4 - For the following exercises, state the domain,...Ch. 6.4 - For the following exercises, state the domain,...Ch. 6.4 - For the following exercises, state the domain,...Ch. 6.4 - For the following exercises, match each function...Ch. 6.4 - For the following exercises, match each function...Ch. 6.4 - For the following exercises, match each function...Ch. 6.4 - For the following exercises, match each function...Ch. 6.4 - For the following exercises, match each function...Ch. 6.4 - For the following exercises, match each function...Ch. 6.4 - For the following exercises, match each function...Ch. 6.4 - For the following exercises, match each function...Ch. 6.4 - For the following exercises, sketch the graphs of...Ch. 6.4 - For the following exercises, sketch the graphs of...Ch. 6.4 - For the following exercises, sketch the graphs of...Ch. 6.4 - For the following exercises, sketch the graphs of...Ch. 6.4 - For the following exercises, match each function...Ch. 6.4 - For the following exercises, match each function...Ch. 6.4 - For the following exercises, match each function...Ch. 6.4 - For the following exercises, sketch the graph of...Ch. 6.4 - For the following exercises, sketch the graph of...Ch. 6.4 - For the following exercises, sketch the graph of...Ch. 6.4 - For the following exercises, sketch the graph of...Ch. 6.4 - For the following exercises, sketch the graph of...Ch. 6.4 - For the following exercises, sketch the graph of...Ch. 6.4 - For the following exercises, write a logarithmic...Ch. 6.4 - For the following exercises, write a logarithmic...Ch. 6.4 - For the following exercises, write a logarithmic...Ch. 6.4 - For the following exercises, write a logarithmic...Ch. 6.4 - For the following exercises, use a graphing...Ch. 6.4 - For the following exercises, use a graphing...Ch. 6.4 - For the following exercises, use a graphing...Ch. 6.4 - For the following exercises, use a graphing...Ch. 6.4 - For the following exercises, use a graphing...Ch. 6.4 - Let b be any positive real number such that b1....Ch. 6.4 - Explore and discuss the graphs of f(x)=log12(x)...Ch. 6.4 - Prove the conjecture made in the previous...Ch. 6.4 - What is the domain of the function f(x)=ln(x+2x4)...Ch. 6.4 - Use properties of exponents to find the...Ch. 6.5 - Expand logb(8k).Ch. 6.5 - Expand log3(7x2+21x7x(x1)(x2)).Ch. 6.5 - Expand ln(x2).Ch. 6.5 - Expand ln(1x2).Ch. 6.5 - Rewrite 2log3(4) using the power rule for logs to...Ch. 6.5 - Expand log(x2y3z4).Ch. 6.5 - Expand ln(x23) .Ch. 6.5 - Expand ln((x1)(2x+1)2x29)Ch. 6.5 - Condense log(3)log(4)+log(5)log(6).Ch. 6.5 - Rewrite log(5)+0.5log(x)log(7x1)+3log(x1) as a...Ch. 6.5 - Condense 4(3log(x)+log(x+5)log(2x+3)).Ch. 6.5 - How does the pH change when the concentration of...Ch. 6.5 - Change log0.5(8) to a quotient of natural...Ch. 6.5 - Evaluate log5(100) using the change-of-base...Ch. 6.5 - How does the power rule for logarithm help...Ch. 6.5 - What does the change-of-base formula do? Whyis...Ch. 6.5 - For the following exercises, expand each logarithm...Ch. 6.5 - For the following exercises, expand each logarithm...Ch. 6.5 - For the following exercises, expand each logarithm...Ch. 6.5 - For the following exercises, expand each logarithm...Ch. 6.5 - For the following exercises, expand each logarithm...Ch. 6.5 - For the following exercises, expand each logarithm...Ch. 6.5 - For the following exercises, condense to a single...Ch. 6.5 - For the following exercises, condense to a single...Ch. 6.5 - For the following exercises, condense to a single...Ch. 6.5 - For the following exercises, condense to a single...Ch. 6.5 - For the following exercises, condense to a single...Ch. 6.5 - For the following exercises, condense to a single...Ch. 6.5 - For the following exercises, use the properties of...Ch. 6.5 - For the following exercises, use the properties of...Ch. 6.5 - For the following exercises, use the properties of...Ch. 6.5 - For the following exercises, use the properties of...Ch. 6.5 - For the following exercises, use the properties of...Ch. 6.5 - For the following exercises, condense each...Ch. 6.5 - For the following exercises, condense each...Ch. 6.5 - For the following exercises, condense each...Ch. 6.5 - For the following exercises, condense each...Ch. 6.5 - For the following exercises, condense each...Ch. 6.5 - For the following exercises, rewrite each...Ch. 6.5 - For the following exercises, rewrite each...Ch. 6.5 - For the following exercises, suppose log5(6)=a and...Ch. 6.5 - For the following exercises, suppose log5(6)=a and...Ch. 6.5 - For the following exercises, suppose log5(6)=a and...Ch. 6.5 - For the following exercises, use properties of...Ch. 6.5 - For the following exercises, use properties of...Ch. 6.5 - For the following exercises, use properties of...Ch. 6.5 - For the following exercises, use the...Ch. 6.5 - For the following exercises, use the...Ch. 6.5 - For the following exercises, use the...Ch. 6.5 - For the following exercises, use the...Ch. 6.5 - For the following exercises, use the...Ch. 6.5 - Use the product rule for logarithms to find all...Ch. 6.5 - Use the quotient rule for logarithms to find all...Ch. 6.5 - Can the power property oflogarithms be derivedfrom...Ch. 6.5 - Prove that logb(n)=1logn(b) for any positive...Ch. 6.5 - Does log81(2401)=log3(7) ? Verify the...Ch. 6.6 - Solve 52x=53x+2.Ch. 6.6 - Solve 52x=253x+2.Ch. 6.6 - Solve 5x=5.Ch. 6.6 - Solve 2x=100.Ch. 6.6 - Solve 2x=3x+1.Ch. 6.6 - Solve 3e0.5t=11.Ch. 6.6 - Solve 3+e2t=7e2t.Ch. 6.6 - Solve e2x=ex+2.Ch. 6.6 - Solve 6+ln(x)=10.Ch. 6.6 - Solve 2ln(x+1)=10.Ch. 6.6 - Use a graphing calculator to estimate the...Ch. 6.6 - Solve ln(x2)=ln(1).Ch. 6.6 - How long will it take before twenty percent of our...Ch. 6.6 - How can an exponential equation be solved?Ch. 6.6 - When does an extraneous solution occur? How canan...Ch. 6.6 - When can the one-to-one property oflogarithms...Ch. 6.6 - For the following exercises, use like bases to...Ch. 6.6 - For the following exercises, use like bases to...Ch. 6.6 - For the following exercises, use like bases to...Ch. 6.6 - For the following exercises, use like bases to...Ch. 6.6 - For the following exercises, use like bases to...Ch. 6.6 - For the following exercises, use like bases to...Ch. 6.6 - For the following exercises, use like bases to...Ch. 6.6 - For the following exercises, use logarithms to...Ch. 6.6 - For the following exercises, use logarithms to...Ch. 6.6 - For the following exercises, use logarithms to...Ch. 6.6 - For the following exercises, use logarithms to...Ch. 6.6 - For the following exercises, use logarithms to...Ch. 6.6 - For the following exercises, use logarithms to...Ch. 6.6 - For the following exercises, use logarithms to...Ch. 6.6 - For the fo?awing exercises, use logarithms to...Ch. 6.6 - For the following exercises, use logarithms to...Ch. 6.6 - For the following exercises, use logarithms to...Ch. 6.6 - For the following exercises, use logarithms to...Ch. 6.6 - For the following exercises, use logarithms to...Ch. 6.6 - For the following exercises, use logarithms to...Ch. 6.6 - For the following exercises, use logarithms to...Ch. 6.6 - For the following exercises, use logarithms to...Ch. 6.6 - For the following exercises, use logarithms to...Ch. 6.6 - For the following exercises, use logarithms to...Ch. 6.6 - For the following exercises, use logarithms to...Ch. 6.6 - For the following exercises, use the definition of...Ch. 6.6 - For the following exercises, use the definition of...Ch. 6.6 - For the following exercises, use the definition of...Ch. 6.6 - For the following exercises, use the definition of...Ch. 6.6 - For the following exercises, use the definition of...Ch. 6.6 - For the following exercises, use the definition of...Ch. 6.6 - For the following exercises, use the definition of...Ch. 6.6 - For the following exercises, use the one-to-one...Ch. 6.6 - For the following exercises, use the one-to-one...Ch. 6.6 - For the following exercises, use the one-to-one...Ch. 6.6 - For the following exercises, use the one-to-one...Ch. 6.6 - For the following exercises, use the one-to-one...Ch. 6.6 - For the following exercises, use the one-to-one...Ch. 6.6 - For the following exercises, use the one-to-one...Ch. 6.6 - For the following exercises, use the one-to-one...Ch. 6.6 - For the following exercises, solve each equation...Ch. 6.6 - For the following exercises, solve each equation...Ch. 6.6 - For the following exercises, solve each equation...Ch. 6.6 - For the following exercises, solve each equation...Ch. 6.6 - For the following exercises, solve each equation...Ch. 6.6 - For the following exercises, solve each equation...Ch. 6.6 - For the following exercises, solve each equation...Ch. 6.6 - For the following exercises, solve the equation...Ch. 6.6 - For the following exercises, solve the equation...Ch. 6.6 - For the following exercises, solve the equation...Ch. 6.6 - For the following exercises, solve the equation...Ch. 6.6 - For the following exercises, solve the equation...Ch. 6.6 - For the following exercises, solve the equation...Ch. 6.6 - For the following exercises, solve the equation...Ch. 6.6 - For the following exercises, solve the equation...Ch. 6.6 - For the following exercises, solve the equation...Ch. 6.6 - For the following exercises, solve the equation...Ch. 6.6 - For the following exercises, solve the equation...Ch. 6.6 - For the following exercises, solve the equation...Ch. 6.6 - For the following exercises, solve the equation...Ch. 6.6 - For the following exercises, solve the equation...Ch. 6.6 - For the following exercises, solve for the...Ch. 6.6 - For the following exercises, solve for the...Ch. 6.6 - For the following exercises, solve for the...Ch. 6.6 - For the following exercises, solve each equation...Ch. 6.6 - For the following exercises, solve each equation...Ch. 6.6 - For the following exercises, solve each equation...Ch. 6.6 - For the following exercises, solve each equation...Ch. 6.6 - For the following exercises, solve each equation...Ch. 6.6 - For the following exercises, use a calculator to...Ch. 6.6 - For the following exercises, use a calculator to...Ch. 6.6 - For the following exercises, use a calculator to...Ch. 6.6 - For the following exercises, use a calculator to...Ch. 6.6 - For the following exercises, use a calculator to...Ch. 6.6 - Use the definition of a logarithm along with the...Ch. 6.6 - Recall the formula for continually...Ch. 6.6 - Recall the compound interest formula A=a(1+rk)kt....Ch. 6.6 - Newton’s Law ofCooling states that the...Ch. 6.7 - The half-life of plutonium-244 is 80,000,000...Ch. 6.7 - Cesium-137 has a half-life of about 30 years. If...Ch. 6.7 - Recent data suggests that, as of 2013, the rate of...Ch. 6.7 - A pitcher of water at 40 degrees Fahrenheit is...Ch. 6.7 - Using the model in Example 6, estimate the number...Ch. 6.7 - Does a linear, exponential, or logarithmic model...Ch. 6.7 - Change the function y=3(0.5)x to one having e as...Ch. 6.7 - With what kind of exponential model would...Ch. 6.7 - Is carbon dating? Why does it work? Give an...Ch. 6.7 - With what kind of exponential model would doubling...Ch. 6.7 - Define Newton’s Law of Cooling. Then name at least...Ch. 6.7 - What is an order of magnitude? Why are orders of...Ch. 6.7 - The temperature of an object in degrees Fahrenheit...Ch. 6.7 - For the following exercises, use the logistic...Ch. 6.7 - For the following exercises, use the logistic...Ch. 6.7 - For the following exercises, use the logistic...Ch. 6.7 - For the following exercises, use the logistic...Ch. 6.7 - For the following exercises, use the logistic...Ch. 6.7 - For the following exercises, use the logistic...Ch. 6.7 - For the following exercises, enter the data from...Ch. 6.7 - For the following exercises, enter the data from...Ch. 6.7 - For the following exercises, enter the data from...Ch. 6.7 - For the following exercises, enter the data from...Ch. 6.7 - For the following exercises, use a graphing...Ch. 6.7 - For the following exercises, use a graphing...Ch. 6.7 - For the following exercises, use a graphing...Ch. 6.7 - For the following exercises, use a graphing...Ch. 6.7 - For the following exercises, use a graphing...Ch. 6.7 - For the following exercises, use a graphing...Ch. 6.7 - A substance has a half-life of 2.045 minutes. If...Ch. 6.7 - The formula for an increasing population is given...Ch. 6.7 - Recall the formula for calculating the magnitude...Ch. 6.7 - What is the y -intercept of the logistic growth...Ch. 6.7 - Prove that bx=exln(b) for positive b1 .Ch. 6.7 - For the following exercises, use this scenario: A...Ch. 6.7 - For the following exercises, use this scenario: A...Ch. 6.7 - For the following exercises, use this scenario: A...Ch. 6.7 - For the following exercises, use this scenario: A...Ch. 6.7 - For the following exercises, use this scenario: A...Ch. 6.7 - For the following exercises, use this scenario: A...Ch. 6.7 - For the following exercises, use this scenario: A...Ch. 6.7 - For the following exercises, use this scenario: A...Ch. 6.7 - For the following exercises, use this scenario: A...Ch. 6.7 - For the following exercises, use this scenario: A...Ch. 6.7 - For the following exercises, use this scenario: A...Ch. 6.7 - For the following exercises, use this scenario: A...Ch. 6.7 - For the following exercises, use this scenario: A...Ch. 6.7 - For the following exercises, use this scenario: A...Ch. 6.7 - For the following exercises, use this scenario: A...Ch. 6.7 - For the following exercises, use this scenario: A...Ch. 6.7 - For the following exercises, use this scenario: A...Ch. 6.7 - For the following exercises, use this scenario: A...Ch. 6.7 - For the following exercises, find the value of the...Ch. 6.7 - For the following exercises, find the value of the...Ch. 6.7 - For the following exercises, find the value of the...Ch. 6.7 - For the following exercises, find the value of the...Ch. 6.7 - For the following exercises, use this scenario:...Ch. 6.7 - For the following exercises, use this scenario:...Ch. 6.7 - For the following exercises, use this scenario:...Ch. 6.7 - For the following exercise, choose the correct...Ch. 6.8 - Table 2 shows a recent graduate’s credit card...Ch. 6.8 - Sales of a video game released in the year 2000...Ch. 6.8 - Table 6 shows the population, in thousands, of...Ch. 6.8 - What situations are best modeled by a logistic...Ch. 6.8 - What is a carrying capacity? What kind of model...Ch. 6.8 - What is regression analysis? Describe the process...Ch. 6.8 - What might a scatterplot of data points look like...Ch. 6.8 - What does the y -intercept on the graph of a...Ch. 6.8 - For the following exercises, match the given...Ch. 6.8 - For the following exercises, match the given...Ch. 6.8 - For the following exercises, match the given...Ch. 6.8 - For the following exercises, match the given...Ch. 6.8 - For the following exercises, match the given...Ch. 6.8 - To the nearest whole number, what is the initial...Ch. 6.8 - Rewrite the exponential model A(t)=1550(1.085)x as...Ch. 6.8 - A logarithmic model is given by the equation...Ch. 6.8 - A logistic model is given by the equation S...Ch. 6.8 - What is the y -intercept on the graph of the...Ch. 6.8 - For the following exercises, use this scenario:...Ch. 6.8 - For the following exercises, use this scenario:...Ch. 6.8 - For the following exercises, use this scenario:...Ch. 6.8 - For the following exercises, use this scenario:...Ch. 6.8 - For the following exercises, use this scenario:...Ch. 6.8 - For the following exercises, use this scenario:...Ch. 6.8 - For the following exercises, use this scenario:...Ch. 6.8 - For the following exercises, use this scenario:...Ch. 6.8 - For the following exercises, use this scenario:...Ch. 6.8 - For the following exercises, use this scenario:...Ch. 6.8 - For the following exercises, refer to Table 7. Use...Ch. 6.8 - For the following exercises, refer to Table 7. Use...Ch. 6.8 - For the following exercises, refer to Table 7....Ch. 6.8 - For the following exercises, refer to Table 7....Ch. 6.8 - For the following exercises, refer to Table 7. Use...Ch. 6.8 - For the following exercises, refer to Table 8. Use...Ch. 6.8 - For the following exercises, refer to Table 8. Use...Ch. 6.8 - For the following exercises, refer to Table 8....Ch. 6.8 - For the following exercises, refer to Table 8....Ch. 6.8 - For the following exercises, refer to Table 8. Use...Ch. 6.8 - For the following exercises, refer to Table 9. Use...Ch. 6.8 - For the following exercises, refer to Table 9. Use...Ch. 6.8 - For the following exercises, refer to Table 9. Use...Ch. 6.8 - For the following exercises, refer to Table 9....Ch. 6.8 - For the following exercises, refer to Table 9. Use...Ch. 6.8 - For the following exercises, refer to Table 10....Ch. 6.8 - For the following exercises, refer to Table 10....Ch. 6.8 - For the following exercises, refer to Table 10....Ch. 6.8 - For the following exercises, refer to Table 10....Ch. 6.8 - For the following exercises, refer to Table 10....Ch. 6.8 - For the following exercises, refer to Table 11....Ch. 6.8 - For the following exercises, refer to Table 11....Ch. 6.8 - For the following exercises, refer to Table 11....Ch. 6.8 - For the following exercises, refer to Table 11. To...Ch. 6.8 - For the following exercises, refer to Table 11....Ch. 6.8 - For the following exercises, refer to Table 12....Ch. 6.8 - For the following exercises, refer to Table 12....Ch. 6.8 - For the following exercises, refer to Table 12....Ch. 6.8 - For the following exercises, refer to Table 12. To...Ch. 6.8 - For the following exercises, refer to Table 12....Ch. 6.8 - Recall that the general form of a logistic...Ch. 6.8 - Use a graphing utility to find an exponential...Ch. 6.8 - Verify the conjecture made in the previous...Ch. 6.8 - Find the inverse function f1(x) for the logistic...Ch. 6.8 - Use the result from the previous exercise to graph...Ch. 6 - Determine whether the function y=156(0.825)t...Ch. 6 - The population of a herd of deer is represented...Ch. 6 - Find an exponential equation that passes through...Ch. 6 - Determine whether Table 1 could represent a...Ch. 6 - A retirement account is opened with an...Ch. 6 - Hsu-Mei wants to save 5,000 for a down paymenton a...Ch. 6 - Does the equation y=2.294e0.654t...Ch. 6 - Suppose an investment account is opened with...Ch. 6 - Graph the function f(x)=3.5(2)x. State the...Ch. 6 - Graph the function f(x)=4(18)x and its...Ch. 6 - The graph of f(x)=6.5x is reflected about the...Ch. 6 - The graph below shows transformations of the graph...Ch. 6 - Rewrite log17(4913)=x as an equivalent...Ch. 6 - Rewrite ln(s)=t as an equivalent...Ch. 6 - Rewrite a25=b as an equivalent...Ch. 6 - Rewrite e3.5=h as an equivalent...Ch. 6 - Solve for xlog64(x)=(13) to exponential form.Ch. 6 - Evaluate log5(1125) without using a calculator.Ch. 6 - Evaluate log(0.000001) without using a calculator.Ch. 6 - Evaluate log(4.005) using a calculator. Round to...Ch. 6 - Evaluate ln(e0.8648) without using a calculator.Ch. 6 - Evaluate ln(183) using a calculator. Round to...Ch. 6 - Graph the function g(x)=log(7x+21)4.Ch. 6 - Graph the function h(x)=2ln(93x)+1.Ch. 6 - State the domain, vertical asymptote, and...Ch. 6 - Rewrite ln(7r11st) in expanded form.Ch. 6 - Rewrite log8(x)+log8(5)+log8(y)+log8(13) incompact...Ch. 6 - Rewrite logm(6783) in expanded form.Ch. 6 - Rewrite ln(z)ln(x)ln(y) in compact form.Ch. 6 - Rewrite ln(1x5) as. a product.Ch. 6 - Rewrite logy(112) as a single logarithm.Ch. 6 - Use properties of logarithm to expand...Ch. 6 - Use properties of logarithms to expand ln(2bb+1b1)Ch. 6 - Condense the expression 5ln(b)+ln(c)+ln(4a)2 to a...Ch. 6 - Condense the expression 3log7v+6log7wlog7u3 to a...Ch. 6 - Rewrite log3(12.75) to base e.Ch. 6 - Rewrite 512x17=125 as a logarithm.Then applythe...Ch. 6 - Solve 2163x216x=363x+2 by rewriting each sidewith...Ch. 6 - Solve 125(1625)x3=53 by rewriting each side with a...Ch. 6 - Use logarithms to find the exact solution for...Ch. 6 - Use logarithms to find the exact solution for...Ch. 6 - Find the exact solution for 5e3x4=6. If there isno...Ch. 6 - Find the exact solution for 2e5x29=56. Ifthere is...Ch. 6 - Find the exact solution for 52x3=7x+1. Ifthere...Ch. 6 - Find the exact solution for e2xex110=0. Ifthere is...Ch. 6 - Use the definition of a logarithm to solve....Ch. 6 - Use the definition of a logarithm to find the...Ch. 6 - Use the one-to-one property of logarithms to find...Ch. 6 - Use the one-to-one property oflogarithms to findan...Ch. 6 - The formula for measuring sound intensity...Ch. 6 - The population of a city is modeled by the...Ch. 6 - Find the inverse function f1 for the exponential...Ch. 6 - Find the inverse function f1 for the...Ch. 6 - For the following exercises, use this scenario: A...Ch. 6 - For the following exercises, use this scenario: A...Ch. 6 - For the following exercises, use this scenario: A...Ch. 6 - For the following exercises, use this scenario: A...Ch. 6 - For the following exercises, use this scenario:...Ch. 6 - For the following exercises, use this scenario:...Ch. 6 - For the following exercises, use this scenario:...Ch. 6 - For the following exercises, enter the data from...Ch. 6 - For the following exercises, enter the data from...Ch. 6 - For the following exercises, enter the data from...Ch. 6 - What is the carrying capacity for a population...Ch. 6 - The population of a culture of bacteria is modeled...Ch. 6 - For the following exercises, use a graphing...Ch. 6 - For the following exercises, use a graphing...Ch. 6 - For the following exercises, use a graphing...Ch. 6 - The population of a pad of bottlenose dolphins...Ch. 6 - Find an exponential equation that passes...Ch. 6 - Drew wants to save 2,500 to go to the nextWorld...Ch. 6 - An investment account was opened with aninitial...Ch. 6 - Graph the function f(x)=5(0.5)x and its...Ch. 6 - The graph below shows transformations of thegraph...Ch. 6 - Rewrite log8.5(614.125)=a as an...Ch. 6 - Rewrite e12=m as an equivalent...Ch. 6 - Solve for x by converting the logarithmic equation...Ch. 6 - Evaluate log(10,000,000) without using a...Ch. 6 - Evaluate ln(0.716) using a calculator. Round to...Ch. 6 - Graph the function g(x)=log(126x)+3.Ch. 6 - State the domain, vertical asymptote, and...Ch. 6 - Rewrite log(17a2b) as a sum.Ch. 6 - Rewrite logt(96)logt(8) in compact form.Ch. 6 - Rewrite log8(a16) as a product.Ch. 6 - Use properties of logarithm to expand ln(y3z2x43).Ch. 6 - Condense the expression...Ch. 6 - Rewrite 163x5=1000 as a logarithm. Then applythe...Ch. 6 - Solve (181)x1243=(19)3x1 rewriting eachside with a...Ch. 6 - Use logarithm to find the exact solution for...Ch. 6 - Find the exact solution for 10e4x+2+5=56. If...Ch. 6 - Find the exact solution for 5e4x14=64. Ifthere is...Ch. 6 - Find the exact solution for 2x3=62x1. If there...Ch. 6 - Find the exact solution for e2xex72=0. If thereis...Ch. 6 - Use the definition ofa logarithm to find the...Ch. 6 - Use the one-to-one property of logarithms to find...Ch. 6 - The formula for measuring sound intensityin...Ch. 6 - A radiation safety officer is working with 112...Ch. 6 - Write the formula found in the previous exerciseas...Ch. 6 - A bottle of soda with a temperature of 71...Ch. 6 - The population of a wildlife habitat is modeledby...Ch. 6 - Enter the data from Table 2 into a graphing...Ch. 6 - The population of a lake of fish is modeled by the...Ch. 6 -
Ch. 6 -
Ch. 6 -
Additional Math Textbook Solutions
Find more solutions based on key concepts
CHECK POINT 1 In a survey on musical tastes, respondents were asked: Do you listed to classical music? Do you l...
Thinking Mathematically (6th Edition)
Fill in each blank so that the resulting statement is true. An equation that expresses a relationship between t...
Algebra and Trigonometry (6th Edition)
Stem plots. In Exercises 7 and 8, construct the stemplot.
7. Pulse Rates Refer to the data listed in Exercise 5...
Elementary Statistics (13th Edition)
The selling price
Pre-Algebra Student Edition
The following set of data is from sample of n=5: a. Compute the mean, median, and mode. b. Compute the range, v...
Basic Business Statistics, Student Value Edition
Explain why or why not Determine whether the following statements are true and give an explanation or counterex...
Calculus: Early Transcendentals (2nd Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, trigonometry and related others by exploring similar questions and additional content below.Similar questions
- Question 10 (5 points) (07.04 MC) Vectors u and v are shown in the graph. -12-11 -10 -9 -8 -7 -6 -5 What is proju? a -6.5i - 4.55j b -5.2i+2.6j с -4.7631 3.334j d -3.81i+1.905j < + 10 6 5 4 3 2 -3 -2 -10 1 -1 -2 -3 u -4 -5 -6 -7arrow_forwardFind the lengths of PR and OR in terms of the angles α and β. Find the angles ∠ONQ and ∠NPQ. Find the lengths of ON and PN in terms of the angle β. Find the length of PQ. Find the length of QR. Find the length of OM. Find the length of RM. What formula can you write down by noting that PR = QR + PQ? What formula can you write down by noting that OR = OM - RM?arrow_forward5) Solve the triangle. 2 95° 4 B с A) c=3.63, A=59.5°, B = 25.5° C) c = 4.63, A = 59.5°, B = 25.5° A B) c 4.63, A 25.5°, B = 59.5° = = D) c 5.63, A = 25.5°, B = 59.5°arrow_forward
- Find zw. Leave your answer in polar form. = လ 3π 2 z = 6 cos 6 cos 37 3π + i sin 2 57 W = 12 cos + i sin 6 6 ༠།ལྦ་arrow_forward10 Write the expression (1 – i) i)in the standard form a + bi.arrow_forward11) The letters r and 0 represent polar coordinates. Write the equation r sine = 10 using rectangular coordinates (x, y). A) x = 10y B) y = 10 C) x = 10 D) y = 10xarrow_forward
- 18) Find all the complex cube roots of - 8i. Leave your answers in polar form with the argument in degrees.arrow_forwardWrite the complex number √3 - i in polar form.arrow_forward2 10) The letters x and y represent rectangular coordinates. Write the equation x² + 4y = 4 using polar coordinates (r, e). A) 4 cos² 0 + sin² 0 = 4r C) r²(4 cos² 0 + sin² 0) = 4 B) cos² 0 + 4 sin² 0 = 4r D) r² (cos20 + 4 sin² 0) = 4arrow_forward
- 2) A radio transmission tower is 130 feet tall. How long should a guy wire be if it is to be attached 6 feet from the top and is to make an angle of 20° with the ground? Give your answer to the nearest tenth of a foot.arrow_forward4) Two sides and an angle are given. Determine whether the given information results in one triangle, two triangles, or no triangle at all. Solve any triangle(s) that results. b=6, c=7, B = 80° A) one triangle B=40°, A = 60°, a = 13 C) one triangle C 39°, A 61°, a = 15 = B) one triangle C=41°, A = 59°, a = 17 D) no trianglearrow_forward7) A painter needs to cover a triangular region 63 meters by 67 meters by 74 meters. A can of paint covers 70 square meters. How many cans will be needed?arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you

 Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning
Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning


Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill

Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning

Functions and Change: A Modeling Approach to Coll...
Algebra
ISBN:9781337111348
Author:Bruce Crauder, Benny Evans, Alan Noell
Publisher:Cengage Learning

Which is the best chart: Selecting among 14 types of charts Part II; Author: 365 Data Science;https://www.youtube.com/watch?v=qGaIB-bRn-A;License: Standard YouTube License, CC-BY