Chapter 6.4, Problem 115E

(a)

To determine

To find: The power by using Statistical Power applet.

Answer to Problem 115E

Solution: The power is 0.885.

Explanation of Solution

To obtain the power by using “Statistical Power applet”, follow the steps below:

Step 1: Go to the “Statistical power” on the website. The screenshot is shown below:

Step 2: Specify “ $H_0:\mu =0$ ” and select $H_a$ as “ $H_a:\mu \ne 0$ ”. The screenshot is shown below:

Step 3: Specify $\sigma$ as “ $\sigma =1$ ” and specify n as “ $n=10$ ”. The screenshot is shown below:

Step 4: Specify Alpha $\left(\alpha \right)$ as “ $\alpha =0.05$ ” and specify $\mu$ as “ $\mu =1$ ”. The screenshot is shown below:

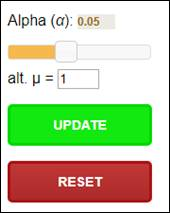
The obtained result is shown below:

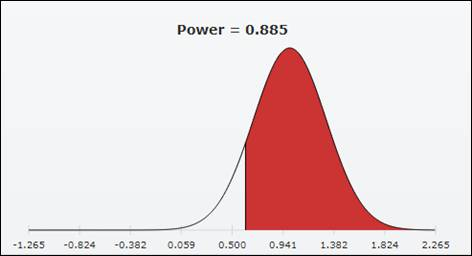
The obtained power is 0.885.

To determine

To find: The power for different values of $\mu$.

Answer to Problem 115E

Solution: The table providing $\mu$ and corresponding the power is shown below:

$\mu$	Power
0.1	0.062
0.2	0.097
0.3	0.158
0.4	0.244
0.5	0.353
0.6	0.475
0.7	0.600
0.8	0.716
0.9	0.812

Explanation of Solution

Calculation:

To obtain the power by using Statistical Power applet, follow the steps below:

Step1: Go to “Statistical power” on the website. The screenshot is shown below:

Step2: Specify “ $H_0:\mu =0$ ” and select $H_a$ as “ $H_a:\mu \ne 0$ ”. The screenshot is shown below:

Step3: Specify $\sigma$ as “ $\sigma =1$ ” and specify n as “ $n=10$ ”. The screenshot is shown below:

Step4: Specify Alpha $\left(\alpha \right)$ as “ $\alpha =0.05$ ” and specify $\mu$ as “ $\mu =0.1$ ”. The screenshot is shown below:

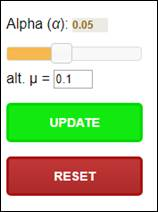
The obtained result is shown below:

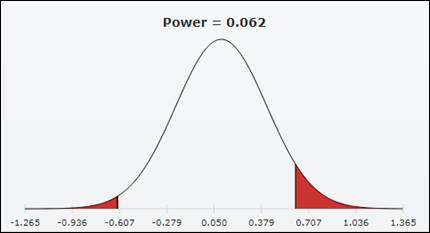
The obtained power is 0.062. Now, repeat this process for the other provided values of $\mu$. Thus, the table given $\mu$ and the power is shown below:

$\mu$	Power
0.1	0.062
0.2	0.097
0.3	0.158
0.4	0.244
0.5	0.353
0.6	0.475
0.7	0.600
0.8	0.716
0.9	0.812

To determine

To explain: The obtained power.

Answer to Problem 115E

Solution: The power decreases as the alternative changes from one-sided alternative to the two-sided alternative.

Explanation of Solution

Power of a test is the capability to discard the null hypothesis when it is not true. It can be seen from the obtained table in exercise 6.114 that as the value of $\mu$ increases, the power also increases. In the above table when one-sided alternative changes to two sided alternative, the power gets decreases.

(b)

To determine

To find: The power.

Answer to Problem 115E

Solution: The power is calculated as 1.00.

Explanation of Solution

Calculation:

To obtain the power by using the“ Statistical Power applet”, follow the steps below:

Step1: Go to the “Statistical power” on the website. The screenshot is shown below:

Step2: Specify “ $H_0:\mu =0$ ” and select $H_a$ as “ $H_a:\mu >0$ ”. The screenshot is shown below:

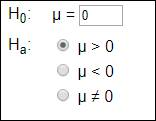
Step3: Specify $\sigma$ as “ $\sigma =0.5$ ” and specify n as “ $n=10$ ”. The screenshot is shown below:

Step4: Specify Alpha $\left(\alpha \right)$ as “ $\alpha =0.05$ ” and specify $\mu$ as “ $\mu =1$ ”. The screenshot is shown below:

The obtained result is shown below:

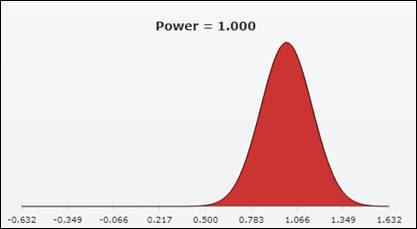
The obtained power is 1.00.

To determine

To find: The power for the different values of $\mu$.

Answer to Problem 115E

Solution: The table providing $\mu$ and the power is shown below:

$\mu$	Power
0.1	0.156
0.2	0.352
0.3	0.600
0.4	0.812
0.5	0.935
0.6	0.984
0.7	0.997
0.8	1.00
0.9	1.00

Explanation of Solution

Calculation:

To obtain the power by using the Statistical Power applet, follow the steps below:

Step1: Go to the “Statistical power” on the website. The screenshot is shown below:

Step2: Specify “ $H_0:\mu =0$ ” and select $H_a$ as $H_a:\mu >0$. The screenshot is shown below:

Step3: Specify $\sigma$ as “ $\sigma =0.5$ ” and specify n as “ $n=10$ ”. The screenshot is shown below:

Step4: Specify Alpha $\left(\alpha \right)$ as “ $\alpha =0.05$ ” and specify $\mu$ as “ $\mu =0.1$ ”. The screenshot is shown below:

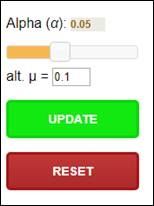
The obtained result is shown below:

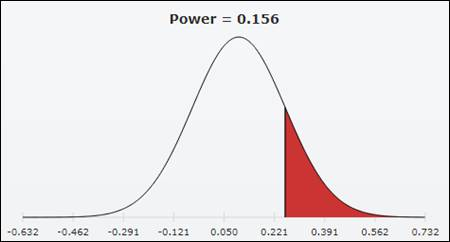
The obtained power is 0.156. Now, repeat this process for the other provided values of $\mu$. Thus, the table given $\mu$ and the power is shown below:

$\mu$	Power
0.1	0.156
0.2	0.352
0.3	0.600
0.4	0.812
0.5	0.935
0.6	0.984
0.7	0.997
0.8	1.00
0.9	1.00

To determine

To explain: The obtained power.

Answer to Problem 115E

Solution: The power increases as $\sigma$ decreases to 0.5..

Explanation of Solution

Power of a test is the capability to discard the null hypothesis when it is not true. It can be seen from the obtained table in the exercise 6.114 that as the value of $\mu$ increases, the power decreases. In the above table when the value of $\sigma$ decreases, the power gets increases.

(c)

To determine

To find: The power.

Answer to Problem 115E

Solution: The power is calculated as 1.00.

Explanation of Solution

Calculation:

To obtain the power by using the“ Statistical Power applet”, follow the steps below:

Step1: Go to the “Statistical power” on the website. The screenshot is shown below:

Step2: Specify “ $H_0:\mu =0$ ” and select $H_a$ as “ $H_a:\mu \ne 0$ ”. The screenshot is shown below:

Step3: Specify $\sigma$ as “ $\sigma =1$ ” and specify n as “ $n=30$ ”. The screenshot is shown below:

Step4: Specify Alpha $\left(\alpha \right)$ as “ $\alpha =0.05$ ” and specify $\mu$ as “ $\mu =1$ ”. The screenshot is shown below:

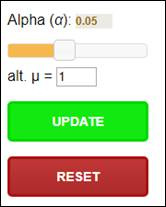
The obtained result is shown below:

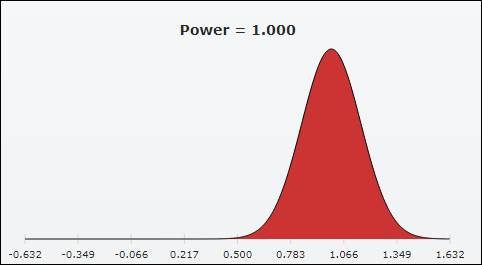
The obtained power is 1.00.

To determine

To find: The power for the different values of $\mu$.

Answer to Problem 115E

Solution: The table providing $\mu$ and the power is shown below:

$\mu$	Power
0.1	0.136
0.2	0.291
0.3	0.499
0.4	0.707
0.5	0.863
0.6	0.950
0.7	0.986
0.8	0.997
0.9	0.999

Explanation of Solution

Calculation:

To obtain the power by using the Statistical Power applet, follow the steps below:

Step1: Go to the “Statistical power” on the website. The screenshot is shown below:

Step2: Specify “ $H_0:\mu =0$ ” and select $H_a$ as $H_a:\mu >0$. The screenshot is shown below:

Step3: Specify $\sigma$ as “ $\sigma =1$ ” and specify n as “ $n=30$ ”. The screenshot is shown below:

Step4: Specify Alpha $\left(\alpha \right)$ as “ $\alpha =0.05$ ” and specify $\mu$ as “ $\mu =0.1$ ”. The screenshot is shown below:

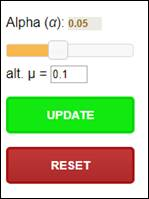
The obtained result is shown below:

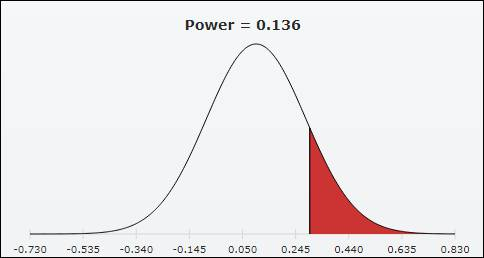
The obtained power is 0.136. Now, repeat this process for the other provided values of $\mu$. Thus, the table given $\mu$ and the power is shown below:

$\mu$	Power
0.1	0.136
0.2	0.291
0.3	0.499
0.4	0.707
0.5	0.863
0.6	0.950
0.7	0.986
0.8	0.997
0.9	0.999

To determine

To explain: The obtained power.

Answer to Problem 115E

Solution: The power increases as the ‘n’ changes 10 to 30.

Explanation of Solution

Power of a test is the capability to discard the null hypothesis when it is not true. It can be seen from the obtained table in the exercise 6.114 that as the value of $\mu$ increases, the power increases. In the above table when the value of n increases, the power gets increases.