EBK COLLEGE ALGEBRA
10th Edition
ISBN: 8220103599528
Author: Larson
Publisher: CENGAGE L
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 6.3, Problem 65E
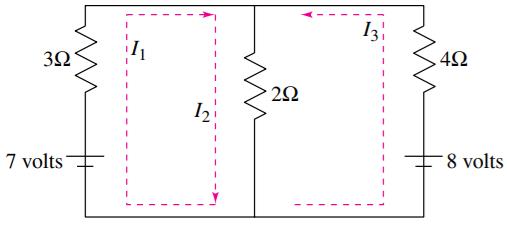
Electrical Network Applying Kirchhoff’s Laws to the electrical network in the figure, the currents
Find the currents.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Solve for the matrix X:
X
(2 7³) x + ( 2 ) - (112)
6
14
8
5.
Solve for the matrix X. (Hint: we can solve AX
-1
= B whenever A is invertible)
2
3
0
Χ
2
=
3
1
Write p(x) = 6+11x+6x² as a linear combination of ƒ (x) = 2+x+4x² and g(x) = 1−x+3x²
and h(x)=3+2x+5x²
Chapter 6 Solutions
EBK COLLEGE ALGEBRA
Ch. 6.1 - Solve the system of equations. xy=05x3y=6Ch. 6.1 - A total of $25,000 is invested in two funds paying...Ch. 6.1 - Solve the system of equations. 2x+y=5x2y+3x=1Ch. 6.1 - Solve the system of equations. 2x+y=32x2+4xy2=0Ch. 6.1 - Prob. 5ECPCh. 6.1 - Prob. 6ECPCh. 6.1 - Prob. 7ECPCh. 6.1 - Prob. 1ECh. 6.1 - Prob. 2ECh. 6.1 - Fill in the blanks. Graphically, solutions of a...
Ch. 6.1 - Prob. 4ECh. 6.1 - Checking Solutions In Exercises 5 and 6, determine...Ch. 6.1 - Checking Solutions In Exercises 5 and 6, determine...Ch. 6.1 - Solving a System by Substitution In Exercises...Ch. 6.1 - Solving a System by Substitution In Exercises...Ch. 6.1 - Solving a System by Substitution In Exercises...Ch. 6.1 - Solving a System by Substitution In Exercises...Ch. 6.1 - Solving a System by Substitution In Exercises...Ch. 6.1 - Prob. 12ECh. 6.1 - Prob. 13ECh. 6.1 - Prob. 14ECh. 6.1 - Solving a System by Substitution In Exercises...Ch. 6.1 - Solving a System by Substitution In Exercises...Ch. 6.1 - Solving a System by Substitution In Exercises...Ch. 6.1 - Solving a System by Substitution In Exercises...Ch. 6.1 - Solving a System by Substitution In Exercises...Ch. 6.1 - Prob. 20ECh. 6.1 - Solving a System by Substitution In Exercises...Ch. 6.1 - Prob. 22ECh. 6.1 - Solving a System by Substitution In Exercises...Ch. 6.1 - Prob. 24ECh. 6.1 - Prob. 25ECh. 6.1 - Prob. 26ECh. 6.1 - Prob. 27ECh. 6.1 - Prob. 28ECh. 6.1 - Solving a System with a Nonlinear Equation In...Ch. 6.1 - Solving a System with a Nonlinear Equation In...Ch. 6.1 - Solving a System with a Nonlinear Equation In...Ch. 6.1 - Solving a System with a Nonlinear Equation In...Ch. 6.1 - Solving a System of Equations Graphically In...Ch. 6.1 - Solving a System of Equations Graphically In...Ch. 6.1 - Solving a System of Equations Graphically In...Ch. 6.1 - Prob. 36ECh. 6.1 - Solving a System of Equations Graphically In...Ch. 6.1 - Prob. 38ECh. 6.1 - Solving a System of Equations Graphically In...Ch. 6.1 - Solving a System of Equations Graphically In...Ch. 6.1 - Prob. 41ECh. 6.1 - Solving a System of Equations Graphically In...Ch. 6.1 - Prob. 43ECh. 6.1 - Prob. 44ECh. 6.1 - Prob. 45ECh. 6.1 - Prob. 46ECh. 6.1 - Prob. 47ECh. 6.1 - Choosing a Solution Method In Exercises 47-54,...Ch. 6.1 - Prob. 49ECh. 6.1 - Prob. 50ECh. 6.1 - Prob. 51ECh. 6.1 - Prob. 52ECh. 6.1 - Prob. 53ECh. 6.1 - Prob. 54ECh. 6.1 - Break-Even Analysis In Exercises 55 and 56, use...Ch. 6.1 - Prob. 56ECh. 6.1 - Break-Even Analysis A small software development...Ch. 6.1 - Prob. 58ECh. 6.1 - DVD Rentals Two new DVDs, a horror film and a...Ch. 6.1 - Prob. 61ECh. 6.1 - Prob. 63ECh. 6.1 - Prob. 64ECh. 6.1 - Prob. 65ECh. 6.1 - Geometry What are the dimensions of a right...Ch. 6.1 - Prob. 67ECh. 6.1 - Prob. 68ECh. 6.1 - Prob. 69ECh. 6.1 - Prob. 70ECh. 6.1 - Think About It Consider the system of equations...Ch. 6.2 - Solve the system of linear equations. 2x+y=42xy=1Ch. 6.2 - Prob. 2ECPCh. 6.2 - Prob. 3ECPCh. 6.2 - Prob. 4ECPCh. 6.2 - Prob. 5ECPCh. 6.2 - Prob. 6ECPCh. 6.2 - Prob. 7ECPCh. 6.2 - Prob. 8ECPCh. 6.2 - Prob. 9ECPCh. 6.2 - Prob. 1ECh. 6.2 - Fill in the blanks. Two systems of equations that...Ch. 6.2 - Prob. 3ECh. 6.2 - Prob. 4ECh. 6.2 - Solving a System by Elimination In Exercises 5-12,...Ch. 6.2 - Solving a System by Elimination In Exercises 5-12,...Ch. 6.2 - Solving a System by Elimination In Exercises 5-12,...Ch. 6.2 - Prob. 8ECh. 6.2 - Solving a System by Elimination In Exercises 5-12,...Ch. 6.2 - Solving a System by Elimination In Exercises 5-12,...Ch. 6.2 - Solving a System by Elimination In Exercises 5-12,...Ch. 6.2 - Solving a System by Elimination In Exercises 5-12,...Ch. 6.2 - Solving a System by Elimination In Exercises...Ch. 6.2 - Solving a System by Elimination In Exercises...Ch. 6.2 - Solving a System by Elimination In Exercises...Ch. 6.2 - Solving a System by Elimination In Exercises...Ch. 6.2 - Solving a System by Elimination In Exercises...Ch. 6.2 - Prob. 18ECh. 6.2 - Solving a System by Elimination In Exercises...Ch. 6.2 - Prob. 20ECh. 6.2 - Prob. 21ECh. 6.2 - Solving a System by Elimination In Exercises...Ch. 6.2 - Prob. 23ECh. 6.2 - Prob. 24ECh. 6.2 - Prob. 25ECh. 6.2 - Prob. 26ECh. 6.2 - Solving a System by Elimination In Exercises...Ch. 6.2 - Prob. 28ECh. 6.2 - Prob. 29ECh. 6.2 - Prob. 30ECh. 6.2 - Matching a System with Its Graph In Exercises...Ch. 6.2 - Prob. 32ECh. 6.2 - Matching a System with Its Graph In Exercises...Ch. 6.2 - Prob. 34ECh. 6.2 - Prob. 35ECh. 6.2 - Prob. 36ECh. 6.2 - Choosing a Solution Method In Exercises 35-40, use...Ch. 6.2 - Choosing a Solution Method In Exercises 35-40, use...Ch. 6.2 - Prob. 39ECh. 6.2 - Prob. 40ECh. 6.2 - Airplane Speed An airplane flying into a headwind...Ch. 6.2 - Airplane Speed Two planes start from Los Angeles...Ch. 6.2 - Nutrition Two cheeseburgers and one small order of...Ch. 6.2 - Nutrition One eight-ounce glass of apple juice and...Ch. 6.2 - Prob. 45ECh. 6.2 - Finding the Equilibrium Point In Exercises 45-48,...Ch. 6.2 - Prob. 47ECh. 6.2 - Prob. 48ECh. 6.2 - Chemistry Thirty liters of a 40% acid solution is...Ch. 6.2 - Fuel Mixture Five hundred gallons of 89-octane...Ch. 6.2 - Investment Portfolio A total of $24,000 is...Ch. 6.2 - Investment Portfolio A total of $32,000 is...Ch. 6.2 - Pharmacology The numbers of prescriptions P (in...Ch. 6.2 - Prob. 54ECh. 6.2 - Prob. 55ECh. 6.2 - Prob. 56ECh. 6.2 - Prob. 57ECh. 6.2 - Prob. 59ECh. 6.2 - Prob. 60ECh. 6.2 - Prob. 61ECh. 6.2 - Finding the Value of a Constant In Exercises 61...Ch. 6.2 - Prob. 63ECh. 6.2 - Prob. 64ECh. 6.2 - Prob. 65ECh. 6.2 - Prob. 66ECh. 6.2 - Prob. 67ECh. 6.2 - Prob. 68ECh. 6.3 - Solve the system of linear equations....Ch. 6.3 - Prob. 2ECPCh. 6.3 - Prob. 3ECPCh. 6.3 - Prob. 4ECPCh. 6.3 - Prob. 5ECPCh. 6.3 - Solve the system of linear equations. xy+4z=34xz=0Ch. 6.3 - Prob. 7ECPCh. 6.3 - Prob. 8ECPCh. 6.3 - Prob. 1ECh. 6.3 - Prob. 2ECh. 6.3 - Prob. 3ECh. 6.3 - Prob. 4ECh. 6.3 - Prob. 5ECh. 6.3 - Prob. 6ECh. 6.3 - Checking Solutions in Exercises 7-10, determine...Ch. 6.3 - Prob. 8ECh. 6.3 - Prob. 9ECh. 6.3 - Prob. 10ECh. 6.3 - Prob. 11ECh. 6.3 - Prob. 12ECh. 6.3 - Prob. 13ECh. 6.3 - Prob. 14ECh. 6.3 - Prob. 15ECh. 6.3 - Prob. 16ECh. 6.3 - Prob. 17ECh. 6.3 - Prob. 18ECh. 6.3 - Prob. 19ECh. 6.3 - Prob. 20ECh. 6.3 - Prob. 21ECh. 6.3 - Prob. 22ECh. 6.3 - Prob. 23ECh. 6.3 - Prob. 24ECh. 6.3 - Prob. 25ECh. 6.3 - Prob. 26ECh. 6.3 - Prob. 27ECh. 6.3 - Prob. 28ECh. 6.3 - Prob. 29ECh. 6.3 - Prob. 30ECh. 6.3 - Prob. 31ECh. 6.3 - Prob. 32ECh. 6.3 - Prob. 33ECh. 6.3 - Prob. 34ECh. 6.3 - Prob. 35ECh. 6.3 - Prob. 36ECh. 6.3 - Prob. 37ECh. 6.3 - Prob. 38ECh. 6.3 - Prob. 39ECh. 6.3 - Prob. 40ECh. 6.3 - Prob. 41ECh. 6.3 - Prob. 42ECh. 6.3 - Prob. 43ECh. 6.3 - Prob. 44ECh. 6.3 - Prob. 45ECh. 6.3 - Prob. 46ECh. 6.3 - Prob. 47ECh. 6.3 - Prob. 48ECh. 6.3 - Prob. 49ECh. 6.3 - Prob. 50ECh. 6.3 - Prob. 51ECh. 6.3 - Prob. 52ECh. 6.3 - Prob. 53ECh. 6.3 - Prob. 54ECh. 6.3 - Prob. 55ECh. 6.3 - Prob. 56ECh. 6.3 - Prob. 57ECh. 6.3 - Agriculture A mixture of 5 pounds of fertilizer A,...Ch. 6.3 - Finance To expand its clothing line, a small...Ch. 6.3 - Advertising A health insurance company advertises...Ch. 6.3 - Geometry In Exercises 61 and 62, find the values...Ch. 6.3 - Prob. 62ECh. 6.3 - Geometry The perimeter of a triangle is 180 feet....Ch. 6.3 - Chemistry A chemist needs 10 liters of a 25 acid...Ch. 6.3 - Electrical Network Applying Kirchhoff’s Laws to...Ch. 6.3 - Pulley System A system of pulleys is loaded with...Ch. 6.3 - Prob. 67ECh. 6.3 - Prob. 68ECh. 6.3 - Prob. 69ECh. 6.3 - Prob. 70ECh. 6.3 - Prob. 71ECh. 6.3 - Prob. 72ECh. 6.3 - Prob. 73ECh. 6.3 - Prob. 74ECh. 6.3 - Prob. 75ECh. 6.3 - The number of sides x and the combined number of...Ch. 6.3 - Prob. 77ECh. 6.3 - Prob. 78ECh. 6.3 - Prob. 79ECh. 6.3 - Prob. 80ECh. 6.4 - Write the partial fraction decomposition of...Ch. 6.4 - Prob. 2ECPCh. 6.4 - Prob. 3ECPCh. 6.4 - Write the partial fraction decomposition of...Ch. 6.4 - Prob. 5ECPCh. 6.4 - Prob. 1ECh. 6.4 - Prob. 2ECh. 6.4 - Prob. 3ECh. 6.4 - Prob. 4ECh. 6.4 - Prob. 5ECh. 6.4 - Prob. 6ECh. 6.4 - Matching In Exercises 5-8, match the rational...Ch. 6.4 - Prob. 8ECh. 6.4 - Writing the Form of the Decomposition In Exercises...Ch. 6.4 - Prob. 10ECh. 6.4 - Writing the Form of the Decomposition In Exercises...Ch. 6.4 - Writing the Form of the Decomposition In Exercises...Ch. 6.4 - Writing the Form of the Decomposition In Exercises...Ch. 6.4 - Prob. 14ECh. 6.4 - Writing the Form of the Decomposition In Exercises...Ch. 6.4 - Prob. 16ECh. 6.4 - Writing the Partial Fraction Decomposition In...Ch. 6.4 - Prob. 18ECh. 6.4 - Writing the Partial Fraction Decomposition In...Ch. 6.4 - Prob. 20ECh. 6.4 - Writing the Partial Fraction Decomposition In...Ch. 6.4 - Prob. 22ECh. 6.4 - Prob. 23ECh. 6.4 - Prob. 24ECh. 6.4 - Prob. 25ECh. 6.4 - Prob. 26ECh. 6.4 - Prob. 27ECh. 6.4 - Prob. 28ECh. 6.4 - Prob. 29ECh. 6.4 - Prob. 30ECh. 6.4 - Prob. 31ECh. 6.4 - Prob. 32ECh. 6.4 - Prob. 33ECh. 6.4 - Prob. 34ECh. 6.4 - Prob. 35ECh. 6.4 - Prob. 36ECh. 6.4 - Prob. 37ECh. 6.4 - Prob. 38ECh. 6.4 - Prob. 39ECh. 6.4 - Prob. 40ECh. 6.4 - Prob. 41ECh. 6.4 - Prob. 42ECh. 6.4 - Prob. 43ECh. 6.4 - Prob. 44ECh. 6.4 - Prob. 45ECh. 6.4 - Prob. 46ECh. 6.4 - Prob. 47ECh. 6.4 - Prob. 48ECh. 6.4 - Prob. 49ECh. 6.4 - Prob. 50ECh. 6.4 - Prob. 51ECh. 6.4 - Prob. 52ECh. 6.4 - Prob. 53ECh. 6.4 - Prob. 54ECh. 6.4 - Prob. 55ECh. 6.4 - Prob. 56ECh. 6.4 - Prob. 57ECh. 6.4 - Prob. 58ECh. 6.4 - Environmental Science The predicted cost C (in...Ch. 6.4 - Thermodynamics The magnitude of the range R of...Ch. 6.4 - Prob. 61ECh. 6.4 - Prob. 62ECh. 6.4 - Prob. 63ECh. 6.4 - HOW DO YOU SEE IT? Identify the graph of the...Ch. 6.4 - Error Analysis Describe the error in writing the...Ch. 6.4 - Prob. 66ECh. 6.5 - Sketch the graph of x+22+y2216.Ch. 6.5 - Sketch the graph of x3.Ch. 6.5 - Prob. 3ECPCh. 6.5 - Sketch the graph of the solution set of the system...Ch. 6.5 - Prob. 5ECPCh. 6.5 - Sketch the solution set of the system of...Ch. 6.5 - Sketch the solution set of the system of...Ch. 6.5 - The demand and supply equations for a flat-screen...Ch. 6.5 - Prob. 9ECPCh. 6.5 - Prob. 1ECh. 6.5 - Prob. 2ECh. 6.5 - Prob. 3ECh. 6.5 - Prob. 4ECh. 6.5 - Prob. 5ECh. 6.5 - Graphing an Inequality In Exercises 5-18, sketch...Ch. 6.5 - Prob. 7ECh. 6.5 - Prob. 8ECh. 6.5 - Prob. 9ECh. 6.5 - Prob. 10ECh. 6.5 - Prob. 11ECh. 6.5 - Graphing an Inequality In Exercises 5-18, sketch...Ch. 6.5 - Prob. 13ECh. 6.5 - Prob. 14ECh. 6.5 - Prob. 15ECh. 6.5 - Graphing an Inequality In Exercises 5-18, sketch...Ch. 6.5 - Prob. 17ECh. 6.5 - Prob. 18ECh. 6.5 - Prob. 19ECh. 6.5 - Prob. 20ECh. 6.5 - Prob. 21ECh. 6.5 - Prob. 22ECh. 6.5 - Prob. 23ECh. 6.5 - Graphing an Inequality In Exercises 19-26, use a...Ch. 6.5 - Prob. 25ECh. 6.5 - Prob. 26ECh. 6.5 - Prob. 27ECh. 6.5 - Writing an Inequality In Exercises 27-30, write an...Ch. 6.5 - Writing an Inequality In Exercises 27-30, write an...Ch. 6.5 - Writing an Inequality In Exercises 27-30, write an...Ch. 6.5 - Prob. 31ECh. 6.5 - Prob. 32ECh. 6.5 - Prob. 33ECh. 6.5 - Prob. 34ECh. 6.5 - Prob. 35ECh. 6.5 - Prob. 36ECh. 6.5 - Prob. 37ECh. 6.5 - Prob. 38ECh. 6.5 - Prob. 39ECh. 6.5 - Prob. 40ECh. 6.5 - Prob. 41ECh. 6.5 - Prob. 42ECh. 6.5 - Prob. 43ECh. 6.5 - Prob. 44ECh. 6.5 - Prob. 45ECh. 6.5 - Prob. 46ECh. 6.5 - Prob. 47ECh. 6.5 - Prob. 48ECh. 6.5 - Prob. 49ECh. 6.5 - Prob. 50ECh. 6.5 - Prob. 51ECh. 6.5 - Prob. 52ECh. 6.5 - Writing a System of Inequalities In Exercises...Ch. 6.5 - Writing a System of Inequalities In Exercises...Ch. 6.5 - Prob. 55ECh. 6.5 - Writing a System of Inequalities In Exercises...Ch. 6.5 - Prob. 57ECh. 6.5 - Prob. 58ECh. 6.5 - Consumer Surplus and Producer Surplus In Exercises...Ch. 6.5 - Prob. 60ECh. 6.5 - Prob. 61ECh. 6.5 - Prob. 62ECh. 6.5 - Prob. 63ECh. 6.5 - Ticket Sales For a concert event, there are $30...Ch. 6.5 - Production A furniture company produces tables and...Ch. 6.5 - Prob. 66ECh. 6.5 - Nutrition A dietician prescribes a special dietary...Ch. 6.5 - Prob. 68ECh. 6.5 - Shipping A warehouse supervisor has instructions...Ch. 6.5 - Physical Fitness Facility A physical fitness...Ch. 6.5 - Prob. 71ECh. 6.5 - Prob. 72ECh. 6.5 - Prob. 73ECh. 6.5 - Prob. 74ECh. 6.5 - Matching Match the system of inequalities with the...Ch. 6.5 - Prob. 76ECh. 6.6 - Find the maximum value of z=4x+5y subject to the...Ch. 6.6 - Find the minimum value of z=12x+8y Where x0 and...Ch. 6.6 - Find the maximum value of z=12x+8y Where x0 and...Ch. 6.6 - Find the minimum value of z=3x+7y Where x0 and y0,...Ch. 6.6 - In Example 5, the candy manufacturer improves the...Ch. 6.6 - Prob. 6ECPCh. 6.6 - Prob. 1ECh. 6.6 - Prob. 2ECh. 6.6 - Prob. 3ECh. 6.6 - Prob. 4ECh. 6.6 - Prob. 5ECh. 6.6 - Prob. 6ECh. 6.6 - Prob. 7ECh. 6.6 - Prob. 8ECh. 6.6 - Prob. 9ECh. 6.6 - Prob. 10ECh. 6.6 - Prob. 11ECh. 6.6 - Prob. 12ECh. 6.6 - Prob. 13ECh. 6.6 - Solving a Linear Programming Problem In Exercises...Ch. 6.6 - Prob. 15ECh. 6.6 - Prob. 16ECh. 6.6 - Prob. 17ECh. 6.6 - Prob. 18ECh. 6.6 - Prob. 19ECh. 6.6 - Prob. 20ECh. 6.6 - Prob. 21ECh. 6.6 - Prob. 22ECh. 6.6 - Prob. 23ECh. 6.6 - Prob. 24ECh. 6.6 - Prob. 25ECh. 6.6 - Prob. 26ECh. 6.6 - Prob. 27ECh. 6.6 - Prob. 28ECh. 6.6 - Prob. 29ECh. 6.6 - Prob. 30ECh. 6.6 - Prob. 31ECh. 6.6 - Prob. 32ECh. 6.6 - Prob. 33ECh. 6.6 - Prob. 34ECh. 6.6 - Prob. 35ECh. 6.6 - Prob. 36ECh. 6.6 - Optimal Profit A merchant plans to sell two models...Ch. 6.6 - Optimal Profit A manufacturer produces two models...Ch. 6.6 - Optimal Cost A public aquarium is adding coral...Ch. 6.6 - Optimal Labor A manufacturer has two different...Ch. 6.6 - Optimal Revenue An accounting firm has 780 hours...Ch. 6.6 - Prob. 42ECh. 6.6 - Agriculture A fruit grower raises crops A and B....Ch. 6.6 - Prob. 44ECh. 6.6 - Prob. 45ECh. 6.6 - Prob. 46ECh. 6.6 - Prob. 47ECh. 6.6 - Prob. 48ECh. 6.6 - Prob. 49ECh. 6.6 - Prob. 50ECh. 6.6 - Prob. 51ECh. 6 - Solving a System by Substitution In Exercises...Ch. 6 - Solving a System by Substitution In Exercises...Ch. 6 - Prob. 3RECh. 6 - Prob. 4RECh. 6 - Prob. 5RECh. 6 - Prob. 6RECh. 6 - Prob. 7RECh. 6 - Prob. 8RECh. 6 - Prob. 9RECh. 6 - Prob. 10RECh. 6 - Prob. 11RECh. 6 - Solving a System of Equations Graphically In...Ch. 6 - Solving a System of Equations Graphically In...Ch. 6 - Prob. 14RECh. 6 - Prob. 15RECh. 6 - Prob. 16RECh. 6 - Prob. 17RECh. 6 - Prob. 18RECh. 6 - Prob. 19RECh. 6 - Prob. 20RECh. 6 - Geometry The perimeter of a rectangle is 68 feet...Ch. 6 - Prob. 22RECh. 6 - Prob. 23RECh. 6 - Prob. 24RECh. 6 - Prob. 25RECh. 6 - Prob. 26RECh. 6 - Solving a System by Elimination In Exercises...Ch. 6 - Solving a System by Elimination In Exercises...Ch. 6 - Matching a System with Its Graph In Exercises...Ch. 6 - Prob. 30RECh. 6 - Prob. 31RECh. 6 - Matching a System with Its Graph In Exercises...Ch. 6 - Finding the Equilibrium Point In Exercises 33 and...Ch. 6 - Prob. 34RECh. 6 - Prob. 35RECh. 6 - Prob. 36RECh. 6 - Prob. 37RECh. 6 - Prob. 38RECh. 6 - Prob. 39RECh. 6 - Prob. 40RECh. 6 - Prob. 41RECh. 6 - Prob. 42RECh. 6 - Prob. 43RECh. 6 - Prob. 44RECh. 6 - Prob. 45RECh. 6 - Prob. 46RECh. 6 - Prob. 47RECh. 6 - Prob. 48RECh. 6 - Agriculture A mixture of 6 gallons of chemical A,...Ch. 6 - Sports The Old Course at St Andrews Links in St...Ch. 6 - Investment An inheritance of $40,000 is divided...Ch. 6 - Investment An amount of $46,000 is divided among...Ch. 6 - Prob. 53RECh. 6 - Prob. 54RECh. 6 - Prob. 55RECh. 6 - Prob. 56RECh. 6 - Prob. 57RECh. 6 - Prob. 58RECh. 6 - Prob. 59RECh. 6 - Prob. 60RECh. 6 - Prob. 61RECh. 6 - Prob. 62RECh. 6 - Prob. 63RECh. 6 - Prob. 64RECh. 6 - Prob. 65RECh. 6 - Prob. 66RECh. 6 - Prob. 67RECh. 6 - Prob. 68RECh. 6 - Prob. 69RECh. 6 - Prob. 70RECh. 6 - Prob. 71RECh. 6 - Prob. 72RECh. 6 - Prob. 73RECh. 6 - Prob. 74RECh. 6 - Prob. 75RECh. 6 - Prob. 76RECh. 6 - Prob. 77RECh. 6 - Prob. 78RECh. 6 - Prob. 79RECh. 6 - Prob. 80RECh. 6 - Geometry Write a system of inequalities to...Ch. 6 - Prob. 82RECh. 6 - Consumer Surplus and Producer Surplus In Exercises...Ch. 6 - Consumer Surplus and Producer Surplus In Exercises...Ch. 6 - Inventory Costs A warehouse operator has 24,000...Ch. 6 - Prob. 86RECh. 6 - Prob. 87RECh. 6 - Prob. 88RECh. 6 - Prob. 89RECh. 6 - Prob. 90RECh. 6 - Prob. 91RECh. 6 - Optimal Profit A manufacturer produces two models...Ch. 6 - Prob. 93RECh. 6 - Prob. 94RECh. 6 - Prob. 95RECh. 6 - Prob. 96RECh. 6 - Prob. 97RECh. 6 - Prob. 98RECh. 6 - Prob. 99RECh. 6 - Prob. 100RECh. 6 - Prob. 101RECh. 6 - Prob. 102RECh. 6 - Prob. 103RECh. 6 - Prob. 104RECh. 6 - In Exercises 1-3, solve the system of equations by...Ch. 6 - In Exercises 1-3, solve the system of equations by...Ch. 6 - Prob. 4TCh. 6 - In Exercises 4-6, solve the system of equations...Ch. 6 - Prob. 6TCh. 6 - In Exercises 7 and 8, solve the system of...Ch. 6 - Prob. 8TCh. 6 - In Exercises 9 and 10, solve the system of linear...Ch. 6 - Prob. 10TCh. 6 - In Exercises 11-14, write the partial fraction...Ch. 6 - Prob. 12TCh. 6 - In Exercises 11-14, write the partial fraction...Ch. 6 - Prob. 14TCh. 6 - In Exercises 15-17, sketch the graph of the...Ch. 6 - Prob. 16TCh. 6 - In Exercises 15-17, sketch the graph of the...Ch. 6 - Prob. 18TCh. 6 - A total of $50,000 is invested in two funds that...Ch. 6 - Prob. 20TCh. 6 - A manufacturer produces two models of television...Ch. 6 - Prob. 1PSCh. 6 - Prob. 2PSCh. 6 - Prob. 3PSCh. 6 - Prob. 4PSCh. 6 - Prob. 5PSCh. 6 - Prob. 6PSCh. 6 - Prob. 7PSCh. 6 - Prob. 8PSCh. 6 - Prob. 9PSCh. 6 - Prob. 10PSCh. 6 - Prob. 11PSCh. 6 - Prob. 12PSCh. 6 - Prob. 13PSCh. 6 - Prob. 14PSCh. 6 - Biology Each day, an average adult moose can...Ch. 6 - Height and Weight For a healthy person who is 4...Ch. 6 - Cholesterol Cholesterol in human blood is...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, algebra and related others by exploring similar questions and additional content below.Similar questions
- 3. Let M = (a) - (b) 2 −1 1 -1 2 7 4 -22 Find a basis for Col(M). Find a basis for Null(M).arrow_forwardSchoology X 1. IXL-Write a system of X Project Check #5 | Schx Thomas Edison essay, x Untitled presentation ixl.com/math/algebra-1/write-a-system-of-equations-given-a-graph d.net bookmarks Play Gimkit! - Enter... Imported Imported (1) Thomas Edison Inv... ◄›) What system of equations does the graph show? -8 -6 -4 -2 y 8 LO 6 4 2 -2 -4 -6 -8. 2 4 6 8 Write the equations in slope-intercept form. Simplify any fractions. y = y = = 00 S olo 20arrow_forwardEXERCICE 2: 6.5 points Le plan complexe est rapporté à un repère orthonormé (O, u, v ).Soit [0,[. 1/a. Résoudre dans l'équation (E₁): z2-2z+2 = 0. Ecrire les solutions sous forme exponentielle. I b. En déduire les solutions de l'équation (E2): z6-2 z³ + 2 = 0. 1-2 2/ Résoudre dans C l'équation (E): z² - 2z+1+e2i0 = 0. Ecrire les solutions sous forme exponentielle. 3/ On considère les points A, B et C d'affixes respectives: ZA = 1 + ie 10, zB = 1-ie 10 et zc = 2. a. Déterminer l'ensemble EA décrit par le point A lorsque e varie sur [0, 1. b. Calculer l'affixe du milieu K du segment [AB]. C. Déduire l'ensemble EB décrit par le point B lorsque varie sur [0,¹ [. d. Montrer que OACB est un parallelogramme. e. Donner une mesure de l'angle orienté (OA, OB) puis déterminer pour que OACB soit un carré.arrow_forward
- 2 Use grouping to factor: 10x + 13x + 3 = 0 Identify A B and C in the chart below feach responce inarrow_forward2 Use grouping to factor: 10x² + 13x + 3 = 0 Identify A, B, and C in the chart below. (each rearrow_forward2 Use grouping to factor: 10x + 13x + 3 = 0 Identify A B and C in the chart below feach responce inarrow_forward
- Use grouping to fully factor: x³ + 3x² - 16x - 48 = 0 3 2arrow_forwardName: Tay Jones Level Two Date: Algebra 3 Unit 3: Functions and Equations Practice Assessment Class: #7-OneNote 1. The function f(x) = x² is transformed in the following functions. List the vertex for each function, circle whether the function opens up or down, and why. All three parts must be correct to receive Level 2 points. You can receive points for a, b, and c. a) g(x) = -2(x+5)² Vertex: Opens Up Opens Down Why? ais negative -2 Vertex: b) g(x) = (x + 2)² - 3 c) g(x) = -4(x + 2)² + 2 Opens Up Opens Down Vertex: Opens Up Opens Down Why? 4 Ca is negative) Why? his positive 2. The graph of the function f(x) is shown below. Find the domain, range, and end behavior. Then list the values of x for which the function values are increasing and decreasing. f(x) Domain: End Behavior: As x → ∞o, f(x) -> -6 As x, f(x) -> Range: Where is it Increasing? (002] Where is it Decreasing? (1,00)arrow_forwardShow what to do on the graph visually please!arrow_forward
- The county's new asphalt paving machine can surface 1 km of highway in 10 h. A much older machine can surface 1 km in 18 h. How long will it take them to surface 21 km of highway if they start at opposite ends and work day and night?arrow_forward3. Write a system of linear equations in slope intercept form that has exactly one solution at the point (3, 4), such that one line has positive slope (but not 1) and the other line has negative slope (but not "1). Also write your system of equations with both equations written in standard form with out any fractions 8- 7 8 5 4 3 -2- + -8-7-6-5-4-3-2-1 1 2 3 -1 2 - ° 4 -5 - -8arrow_forward2. Write a system of linear equations in slope-intercept form has exactly one solution at the point (3, 4), such that both lines have negative slope (but neither one has slope of 1). Also write your system of equations with both equations written in standard form without any fractions. B 0 5 4 3 -2 1 -8-7-6-5-4-3-2 -1 12 3 -1 2 -3 -5 6 -7 -8arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Elementary Linear Algebra (MindTap Course List)AlgebraISBN:9781305658004Author:Ron LarsonPublisher:Cengage Learning
College AlgebraAlgebraISBN:9781305115545Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Algebra and Trigonometry (MindTap Course List)
Algebra
ISBN:9781305071742
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Elementary Linear Algebra (MindTap Course List)
Algebra
ISBN:9781305658004
Author:Ron Larson
Publisher:Cengage Learning
College Algebra
Algebra
ISBN:9781305115545
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning
Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning
Matrix Factorization - Numberphile; Author: Numberphile;https://www.youtube.com/watch?v=wTUSz-HSaBg;License: Standard YouTube License, CC-BY