
Concept explainers
To approximate the distance from the Earth to stars relatively close by. astronomers often use the method of parallax. Parallax is the apparent displacement of an object caused by a change in the observer's point of view. As the Earth orbits the Sun, a nearby star will appear to move against the more distant background stars. Astronomers measure a star's position at times exactly
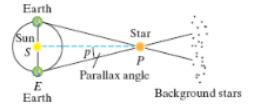
a. Find the distance between the Earth and Proxima Centauri (the closest star to the Earth beyond the Sun) if the parallax angle is
b. Write the distance in part (a) in light-years. Round to
Want to see the full answer?
Check out a sample textbook solution
Chapter 6 Solutions
ALEKS 360 ACCESS CARD PRECALCULUS 18 WK
Additional Math Textbook Solutions
Elementary Statistics: Picturing the World (7th Edition)
Algebra and Trigonometry (6th Edition)
University Calculus: Early Transcendentals (4th Edition)
Thinking Mathematically (6th Edition)
Using and Understanding Mathematics: A Quantitative Reasoning Approach (6th Edition)
- In the triangle below, x = 7. Use the Law of Cosines to solve the triangle. A = B = C = 12 cm 18 cm B x cm ° о °arrow_forwardA triangular parcel of ground has sides of length 750 feet, 650 feet, and 535 feet. Find the measure of the largest angle. (Round your answer to one decimal place.)arrow_forwardA boat is sailing due east parallel to the shoreline at a speed of 10 miles per hour. At a given time, the bearing to a lighthouse is S 70° E, and 15 minutes later, the bearing is S 63° E (see figure). The lighthouse is located at the shoreline. Find the distance d from the boat to the shoreline. (Round your answer to one decimal place.) x mi N 63° WE 70° Sarrow_forward
- A 120-foot vertical tower is to be erected on the side of a hill that makes a 6° angle with the horizontal. Find the length of each of the two guy wires that will be anchored 75 feet uphill and downhill from the base of the tower (see figure). (Note that x = 120 in the figure. Round your answers to one decimal place.) shorter wire longer wire x ft ft ft XXXX -75 ft -75 ftarrow_forwardhelp with workarrow_forward۳/۱ +① العنوان I need a detailed drawing with explanatic Le R2X2 2) slots per pole per phase = 3/31 B: 18060 msl Kas Kdl Sin (1) I sin () sin(30) Sin (30) اذا ميريد شرح الكتب بس بالفراغ Cos (30) 0.866 4) Rotating 5) Synchronous speed, 120 x 50 SE 1000-950 1000 Copper losses 5kw 6 50.05 Rotor input 5 0.05 loo kw اذا ميريد شرح الكتب فقط ok 7) rotov DC 1000 rpm ined sove in peap PU + 96er Which of the following is converge, and which diverge? Give reasons for your answers with details. When your answer then determine the convergence sum if possible. 1/n -√ Which of the following is converge, and which diverge? Give reasons for your answers. with details. When your answer then determine the convergence sum if possible. 3" 6" '1Σn=1 (2-") n T GI Marrow_forward
- V ined sove in peaper Pu+96er Which of the following is converge, and which diverge? Give reasons for your answers with details. When your answer then determine the convergence sum if possible. 21/11 55 a Which of the following is converge, and which diverge? Give reasons for your answers with details. When your answer then determine the convergence sum if possible. 1Σn=1 (2-") n° 3" 6"arrow_forward: +0 1 R2X2 العنوان I need a detailed drawing with explanation L L 2) slots per pole per phase = 3/31 B = 180-60 msl Kd Kol, Sin (Info) Isin (6) sin(30) Sin (30) اذا ميريد شرح الكتب بس 0 بالفراغ 3) Cos (30) 0.866 4) Rotating 5) Synchronous speed, 120 x 50 6 50105 1000 S=1000-950 Loco mem 6. Copper losses: 5kw Rotor input loo kw 0.05 اذا ميريد شرح الكتب فقط look 7) rotov DC ined sove in peaper Pu+965 4 Which of the following is converge, and which diverge? Give reasons for your answers with details. When your answer then determine the convergence sum if possible. 111Σm=1 sin() Lake Which of the following is converge, and which diverge? Give reasons for your answers with details. When your answer then determine the convergence sum if possible. T TH Marrow_forwardい ined sove in beaper Anting. Pu+965 na lake an accident and lands at the bottom of the lake Q2// Find the volume of the region in first octant bounded by the coordinate planes and the plane passing through (1, 0, 0), (0, 2, 0), and (0, 0, 3). Q/Evaluate({ } } 3xze* dydzdx.arrow_forward
- | Evaluate (3xze** dydzdx. ined sove in peaper +9198 PU+965 Lake Find the volume of th solid bounded above by the Cy 2=6-1 o the sides by the cylinder x+y=9, and below by the xy-planearrow_forward... +① العنوان > पर ined sove in peaper ང་ PU+965 Q2// Draw and Evaluate, or Integrate, the function f(u, v) = (1+u2+v²)3 over the region enclosed by one loop of the lemniscate (u² + v²)² - (u² + v²) = 0. Lake 2 4-2² y 7357 r QI// Evaluate f²² cos(y) dxdydz. 4-y 이arrow_forwardし ined sove in peaper Anot in PV+96252 √4-x²-y² Q4// Convert √ √ √2x-x2 √√4-x-2_ 21xy² dzdydx to (a) cylindrical coordinates, (b) Spherical coordinates. ln3 (m3)2-x2 Q Draw and Evaluate Lake √x²+ dydarrow_forward
- Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
 Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,  Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage Learning Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,





