Mathematics For Machine Technology
8th Edition
ISBN: 9781337798310
Author: Peterson, John.
Publisher: Cengage Learning,
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 61, Problem 15A
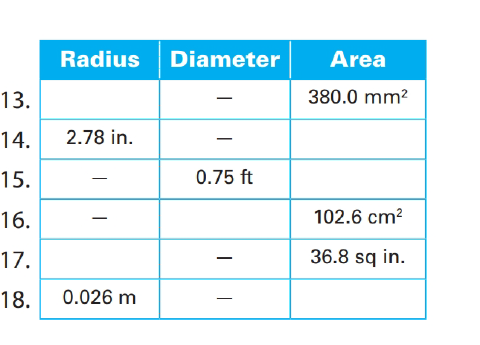
Find the unknown area, or diameter for each of the circles 7 through 18. Where necessary, round the answers to 1 decimal place.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
COMPLETE
THREE-VIEW ORTHOGRAPHIC SKETCHES OF THE
FOLLOWING OBJECTS
USE ORTHO GRID PAPER.
Drawn By:
No chatgpt pls will upvote
2
Q/ Let d₂
+d, di, d2: R² XR² R² defined as follow
((x+x), (2, 1) = √(x-2)² + (x_wx
• d₁ ((x,y), (z, w)) = max {1x-z\, \y-w\}
•
1
1
dq ((x,y), (Z, W)) = \ x=2\+\-w|
2
• show that dod₁, d₂ are equivalent?
2
Chapter 61 Solutions
Mathematics For Machine Technology
Ch. 61 - Plans call for triangle BCDto be sheared off a...Ch. 61 - Find the area of the shaded portion of this...Ch. 61 - Four circles are equally spaced on a bolt circle...Ch. 61 - What is the radius of a circle with a...Ch. 61 - What is the supplement of a 17548'29" angle?Ch. 61 - Prob. 6ACh. 61 - Find the unknown area, or diameter for each of the...Ch. 61 - Find the unknown area, or diameter for each of the...Ch. 61 - Find the unknown area, or diameter for each of the...Ch. 61 - Find the unknown area, or diameter for each of the...
Ch. 61 - Find the unknown area, or diameter for each of the...Ch. 61 - Find the unknown area, or diameter for each of the...Ch. 61 - Find the unknown area, or diameter for each of the...Ch. 61 - Find the unknown area, or diameter for each of the...Ch. 61 - Find the unknown area, or diameter for each of the...Ch. 61 - Find the unknown area, or diameter for each of the...Ch. 61 - Find the unknown area, or diameter for each of the...Ch. 61 - Find the unknown area, or diameter for each of the...Ch. 61 - A rectangular steel plate 15.10 inches long and...Ch. 61 - Hydraulic pressure of 705.0 pounds per square inch...Ch. 61 - A circular base is shown. The base is cut from a...Ch. 61 - Find the area of the template shown. Round the...Ch. 61 - A force of 62,125 pounds pulls on a steel rod that...Ch. 61 - A piece shown by the shaded portion is to be cut...Ch. 61 - Find the unknown area, radius, or central angle...Ch. 61 - Find the unknown area, radius, or central angle...Ch. 61 - Find the unknown area, radius, or central angle...Ch. 61 - Find the unknown area, radius, or central angle...Ch. 61 - Find the unknown area, radius, or central angle...Ch. 61 - Find the unknown area, radius, or central angle...Ch. 61 - Find the unknown area, radius, or central angle...Ch. 61 - Find the unknown area, radius, or central angle...Ch. 61 - Prob. 33ACh. 61 - Prob. 34ACh. 61 - Prob. 35ACh. 61 - Find the unknown area, radius, or central angle...Ch. 61 - A section of a piece of round stock with a...Ch. 61 - Three pieces, each in the shape of a sector, are...Ch. 61 - Prob. 39ACh. 61 - Prob. 40ACh. 61 - Find the area of each of the segments ACE for...Ch. 61 - Prob. 42ACh. 61 - Prob. 43ACh. 61 - Prob. 44ACh. 61 - Prob. 45ACh. 61 - Prob. 46ACh. 61 - Compute the area of the steel insert (shaded...Ch. 61 - A pattern is shown. a. Find the surface area of...Ch. 61 - The shaded piece shown is cut from a circular...Ch. 61 - Prob. 50A
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, advanced-math and related others by exploring similar questions and additional content below.Similar questions
- 2 +d, di, d2: R² XR² > R² defined as follow Q/ Let d₂ 2/ d((x+x), (2, 1)) = √(x-2)² + (x-wsc • d₁ ((x,y), (z, w)) = max {| x-z\, \y-w\} • d₂ ((x, y), (Z, W)) = 1x-21+ \y-w| 2 • show that ddi, d₂ are equivalent? އarrow_forwardNumerical anarrow_forward1. Prove the following arguments using the rules of inference. Do not make use of conditional proof. (а) а → (ЪЛс) ¬C ..¬a (b) (pVq) → →r יור (c) (c^h) → j ¬j h (d) s→ d t d -d ..8A-t (e) (pVg) (rv¬s) Лѕ קר .'arrow_forward
- 2. Consider the following argument: (a) Seabiscuit is a thoroughbred. Seabiscuit is very fast. Every very fast racehorse can win the race. .. Therefore, some thoroughbred racehorse can win the race. Let us define the following predicates, whose domain is racehorses: T(x) x is a thoroughbred F(x) x is very fast R(x) x can win the race : Write the above argument in logical symbols using these predicates. (b) Prove the argument using the rules of inference. Do not make use of conditional proof. (c) Rewrite the proof using full sentences, avoiding logical symbols. It does not need to mention the names of rules of inference, but a fellow CSE 16 student should be able to understand the logical reasoning.arrow_forwardFind the inverse of the matrix, or determine that the inverse does not exist for: € (b) 7 -12 240 1 1 1 (c) 2 3 2 2 17 036 205 20 (d) -1 1 2 1 T NO 1 0 -1 00 1 0 02 (e) 1 0 00 0 0 1 1arrow_forward4. Prove the following. Use full sentences. Equations in the middle of sentences are fine, but do not use logical symbols. (a) (b) (n+3)2 is odd for every even integer n. It is not the case that whenever n is an integer such that 9 | n² then 9 | n.arrow_forward
- 3. (a) (b) Prove the following logical argument using the rules of inference. Do not make use of conditional proof. Vx(J(x)O(x)) 3x(J(x) A¬S(x)) . ·.³x(O(x) ^ ¬S(x)) Rewrite the proof using full sentences, avoiding logical symbols. It does not need to mention the names of rules of inference, but a fellow CSE 16 student should be able to understand the logical reasoning.arrow_forwardNo chatgpt pls will upvote Already got wrong chatgpt answerarrow_forward16.4. Show that if z' is the principal value, then 1+e** z'dz = (1-i), 2 where is the upper semicircle from z = 1 to z = -1.arrow_forward
- L 16.8. For each of the following functions f, describe the domain of ana- lyticity and apply the Cauchy-Goursat Theorem to show that f(z)dz = 0, where is the circle |2|=1:1 (a). f(z) = 1 z 2 + 2x + 2 (b). f(z) = ze*. What about (c). f(z) = (2z-i)-2?arrow_forward16.3. Evaluate each of the following integrals where the path is an arbitrary contour between the limits of integrations (a). [1 ri/2 edz, (b). (b). La cos COS (2) d dz, (c). (z−3)³dz. 0arrow_forwardQ/ prove that:- If Vis a finite dimensional vector space, then this equivalence relation has only a single equivalence class.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
- Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL 
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,
Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill
Holt Mcdougal Larson Pre-algebra: Student Edition...
Algebra
ISBN:9780547587776
Author:HOLT MCDOUGAL
Publisher:HOLT MCDOUGAL
Elementary Geometry For College Students, 7e
Geometry
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Cengage,
Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
Problems on Area and Circumference of Circle| Basics of Circle| Questions on Circle||BrainPanthers; Author: Brain Panthers;https://www.youtube.com/watch?v=RcNEL9OzcC0;License: Standard YouTube License, CC-BY